10 Fun Math Projects for All Grade Levels
Math projects are fun inquiry-based activities that give students a chance to extend their understanding of math skills and concepts to real-world problems in a hands-on way. There are many types of math projects, and they often involve creative thinking, collaboration, and meaningful applications of math including modeling real-world scenarios, working with hands-on materials, and analyzing data.
Last Updated: May 2025
If you are looking for a few fun math project ideas for mixing up your instruction or for keeping your students interested and engaged for any reason, then you will love this collection of fun math projects for students in grades 1-8. Having students work on fun math projects (and math art projects) is a great way to keep their attention and break up the monotony of the normal classroom routine.
The following math project ideas for elementary school, middle school, and high school students can all be modified to appropriately challenge and engage your math students based on their interests and skill/ability levels. We highly recommend that you differentiate whatever math project you choose to best meet the needs of your students.
Table of Contents (Jump To):
Now, are you ready to learn about some fun math projects that you can use to engage your students this school year?
1.) The Ultimate Paper Airplane Competition
Suggested Grade Levels: Grades 3-6
Materials/Supplies: Printer Paper, Card Stock, Measuring Tape, Masking Tape, Stopwatch or Phone Timer
The Ultimate Paper Airplane Competition is a fun math project involving data collection and analysis. (Image: Mashup Math g)
Description: This math project focuses on data collection and analysis in the context of flying paper airplanes. Students can start by building their own paper airplanes and then flying them in an open area such as a hallway, a gymnasium, or outdoors. By working in small groups, students can take turns throwing their paper airplanes from a fixed starting point. They can collect data related to things like air time, distance flown, and target accuracy. After three to five test flights, they can use their collected data to calculate data such as:
The mean flight air time and/or distance of their plane.
The range between the longest and shortest flights.
The median and mode of the data points (if applicable).
Students can also collaborate with other groups to track the data of other paper airplanes in relation to their own. Finally, a large group data-driven discussion can be had to determine which group had the highest performing paper airplane and why.
Advanced Application: If you want to modify this activity to be more challenging, include a graphing component where students have to compare two data points such as distance vs air time, or distance vs. wingspan.
Click here to learn more about the Ultimate Paper Airplane Competition Project
2.) Dream Home Design Project
Suggested Grade Levels: Grades 5-8
Materials/Supplies: Graph Paper, Rulers and Protractors, Colored Pencils or Markers, Clipboards
Are your students ready to design their dream home blueprints?
Description: For this fun math art project, students are tasked with designing the floor plans for their dream homes and backyards by applying math skills including measurement, defining dimensions, scale, area, and perimeter. Students can use graph paper and markers or digital tools like Google Sketchup to create their home’s blueprints, calculate the cost of building materials and furniture, and design the layout of their house’s interior and exterior.
Students can also be given the opportunity to present their dream home blueprint to their classmates.
Advanced Application: You can make this math project more challenging by incorporating a research phase and including a budget constraint, so students have to maximize their total living space under a budget.
3.) Turn Your Classroom into an Escape Room
Suggested Grade Levels: 4-8
Materials/Supplies: Envelopes or plastic “lock boxes,” printable clue cards, timers or stopwatches, UV pens and black-lights (if using invisible clues), Clipboards
Math Projects for Middle School Students: Puzzles, Riddles, and Brain Teasers (Image Source: Jackson Academy)
Description: Can your students escape before the bell rings? The escape room project has students working in groups of 3-5 to “escape” your classroom before the bell rings by solving a sequence of math problems and puzzles. Every time the group solves a problem, they earn a keyword or a clue that will help them with the next step.
You can use the math escape room project for a variety of topics including working with fractions, solving equations, identifying shapes and figures, etc.
This math project will take some time to plan and implement, but it’s an activity that you can reuse year after year.
4.) Data Journalism - Class Survey
Suggested Grade Levels: 1-8
Materials/Supplies: Clipboards, Tally Charts, Data Entry Charts, Graph Paper, Rulers, Colored Pencils
Have your students collect responses from their classmates, analyze the data, and write a blog post highlighting their results. (Image: Mashup Math FP)
Description: The data journalism activity is a highly-flexible math project idea that works with any topic or grade level. For this project, students must design a survey that collects data by asking 5 or more questions such as What is your favorite lunch type of candy? or How many minutes, on average, do you spend working on homework on a school night? Once students have created their surveys, they can collect responses from their classmates (aim to have each student collect responses from at least 25 people). Next, students can track data using tally marks, frequency tables, and/or charts and graphs. Using the collected data, students can compute and analyze a variety of statistics ranging from simple frequency comparisons to central tendency to standard deviation.
Advanced Application: In addition to collection data and analyzing it, students can draw conclusions and share them via writing their own blog post or news story (with a catchy headline, of course). For example, a student could write a bombshell article titled “Are Teachers Giving Us Too Much Homework? 70% of Students Spend 2+ Hours Per Night on HW!”
Do you more FREE K-8 math resources and activity ideas in your inbox every week?
5.) Budgeting Your Dream Vacation
Suggested Grade Levels: 4-8
Materials/Supplies: Maps, Travel Brochures, Graph Paper, Laptops with Internet Access
Are your students ready to plan and budget their dream vacation?
Description: For this math project, choose an appropriate budget for your students to spend on planning a dream vacation for themselves and a friend. Students must research the cost of travel, including transportation, lodging, meals, activities and experiences, and shopping for a 7 day vacation at the destination of their choice. Students can track their spending in a variety of ways, including simple budget tables and spreadsheets. Students can also use graphic tools like bar graphs and pie charts to visualize spending categories.
Advanced Application: You can make this math project more challenging by requiring students to choose a foreign country destination that requires them to make currency conversions and to research travel requirements such as passports, visas, vaccinations, and travel insurance.
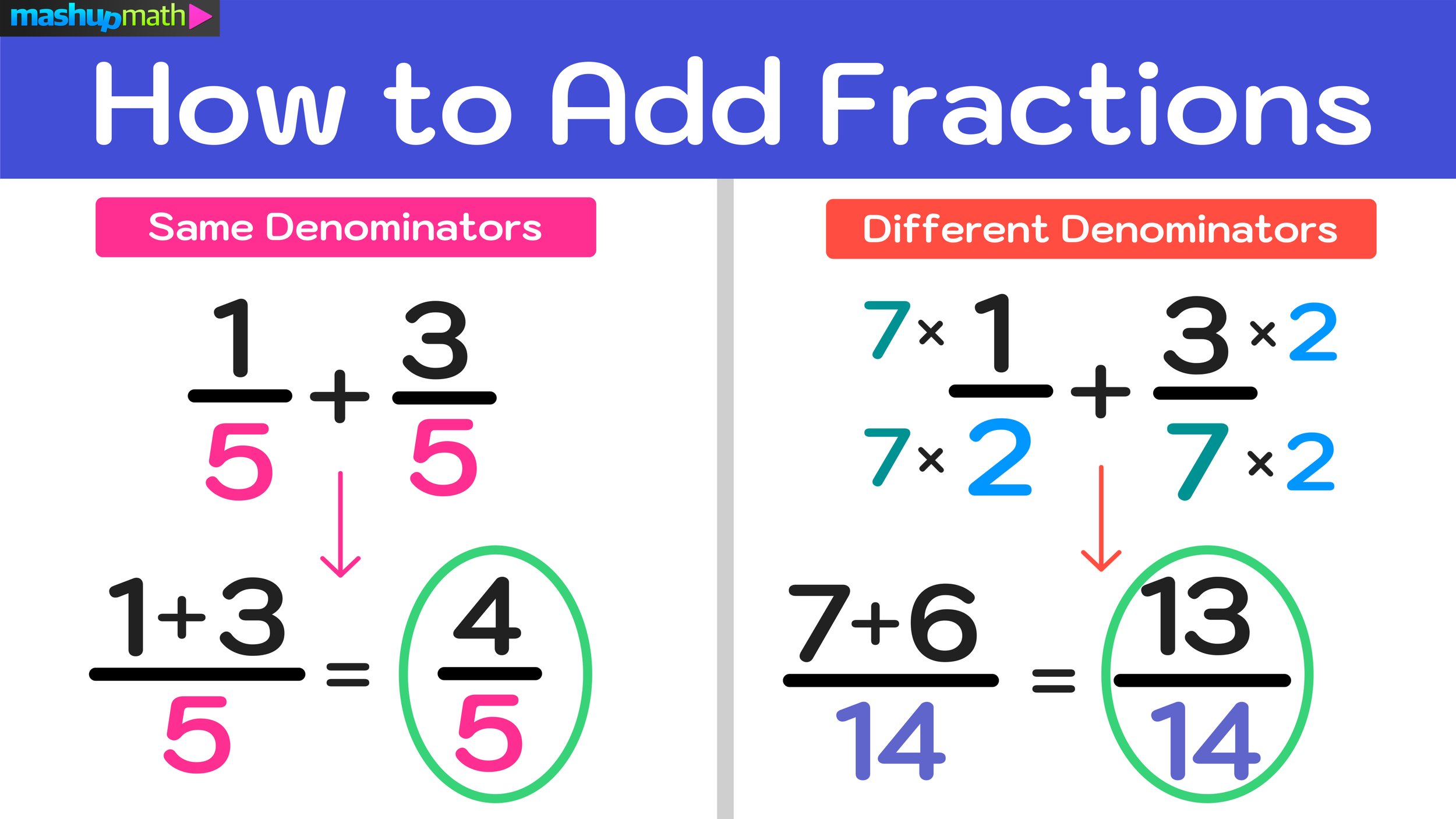
6.) Build a Fraction Kit
Suggested Grade Levels: 4-8
Materials/Supplies: Construction Paper, Scissors, Markers, Rulers
Image via www.mashupmath.com
Description: Building a fraction kit using colored construction paper is one of the best ways to help your students to understand math concepts related to fractions, including simplifying fractions, equivalent fractions, comparing fractions, and adding and subtracting fractions.
Students can keep their completed fraction kits in their math notebooks to use a reference guide whenever they need help with things like comparing fractions, simplifying fractions, or adding/subtracting fractions.
Click here for step-by-step instructions on building a fraction kit
7.) Research a Famous Mathematician
Grade Levels: 1-8
Materials/Supplies: Laptops with Internet Access, List of Research Questions
Math Projects: Research a Famous Mathematician or Civilization
Description: For this math project, students will research a famous individual or civilization and their contributions to the field of mathematics and create a profile that they will present to their classmates.
Students will start by selecting a mathematician or civilization and then researching key facts and details such as biographical details, historical context, and major contributions. Students can then create a presentation that profiles their mathematician that includes an image or portrait, key information, and fun facts.
Here are a few great resources for inspiring students to learn about some lesser-known mathematicians and their amazing contributions to mathematics:
8.) Create Your Own Math Board Game
Grade Levels: 1-8
Materials/Supplies: Poster board, scissors, colored pens or markers, 6-sided dice, spinners
Having your students create their own math board game is a fun and creative math project idea.
Description: For this math project, students are tasked with creating their own math-related board games based on an assigned topic/skill or one of their choosing. To complete this project, students must choose a concept, plan their game, create a game board, design the game pieces, uses spinners or dice to determine how players will navigate the board, test and revise the game, and present their final product to the class.
Students can also be given time to rotate around the classroom in groups to attempt to play each other’s board games.
9.) Stock Market Project
Grade Levels: 6-8
Materials/Supplies: Laptops or Desktop Computers or Tablets (with Internet Access), Spreadsheet Software (Excel or Google Sheets)
The stock market math project teaches students about investing.
Description: Are your students ready to learn about investing? The stock market math project has students start with a simulated $10,000 investment budget that they must invest into ten publicly traded companies such as Nike, Microsoft, Tesla, McDonald’s, etc. Students must complete a spreadsheet or chart that includes their ten stocks (and each stock’s ticker symbol), the purchase price, the number of shares purchased, and the total cost invested into each position. Students can then track the performance of their portfolios over time by updating the performance every month or so.
We recommend showing this short TED-Ed Video How Does the Stock Market Work and using Google to research companies, find stock symbols, and see corresponding graphs and charts during the research phase.
10.) Tessellation Art Project
Grade Levels: 4-8
Materials/Supplies: Construction Paper, Scissors, Rulers, Colored Pencils or Markers, Poster Board
Description: This final math project idea combines geometry and art, where students have to create an Escher Tile from a regular polygon (a square, a hexagon, or an equilateral triangle).
The video below teaches you how to create your own Escher tile. Once students have a few shapes, they can pick one that resembles something recognizable such as an animal or a fruit.
Then, they can use their tile to make a pattern image that they can then color in and display on the walls of your classroom.
Are Your Students Ready to Play Math Jeopardy?
In addition to working on fun math projects, you can also mix up your instruction by giving your students the opportunity to play our interactive Math Jeopardy Games for grades 3-6.
Click on any of the links below to play Math Jeopardy.
More Math Education Insights You Will Love:
Search Tags: math art projects, math projects for middle school, math projects, math project ideas, 21st century math projects, middle school math projects, math projects for 5th graders