How to Multiply Fractions with Whole Numbers
Step-by-Step Guide: How to Multiply Fractions with Whole Numbers, Multiplying Fractions by Whole Numbers Examples
Multiplying fractions with whole numbers can seem like a challenging math skill, but, with some simple strategies and an easy step-by-step method, it can be a relatively easy task that any student can master.
In this free guide, we will work through several examples of how to multiply fractions with whole numbers using a simple step-by-step process. As long as you can follow three easy steps, you will be able to confidently and accurately solve a variety of math problems where you have to multiply fractions with whole numbers.
You can work through the sections in this free guide in sequential order, or you can click on any of the quick-links below to jump to one particular section.
Quick Intro: Multiply Fractions with Whole Numbers
Before we dive into any examples of how to multiply fractions with whole numbers, let’s do a quick introductory review of what it means when we multiply fractions with whole numbers.
For example, let’s consider the example 3 x 1/4:
3 is the whole number
1/4 is the fraction
Whenever you multiply a fraction by a whole number, you are really just performing repeated addition (i.e. you are adding the fraction to itself a number of repeated times that is determined by the whole number).
If we think of multiplication in terms of repeated addition, we can rewrite 3 x 1/4 as follows:
3 x 1/4 = 1/4 + 1/4 + 1/4
And, since 1/4 + 1/4 + 1/4 is equal to 3/4, we can conclude that:
3 x 1/4 = 1/4 + 1/4 + 1/4 = 3/4
Final Answer: 3 x 1/4 = 3/4
Figure 01: How to Multiply Fractions with Whole Numbers Using Repeated Addition.
The process of multiplying fractions with whole numbers using repeated addition is shown in Figure 01.
While we will not use repeated addition to solve the examples in this guide, understanding this basic relationship between multiplication and repeated addition is the first step to easily learning how to multiply fractions with whole numbers.
Now, let’s go ahead and work through some examples of multiplying fractions with whole numbers using a simple 3-step method.
Multiplying Fractions by Whole Numbers Examples
For all of the multiplying fractions with whole numbers examples that follow, we will be using the following 3-step method for solving:
Step 1: Rewrite the whole number as a fraction with a denominator of 1.
Step 2: Multiply the numerators together and then multiply the denominators together.
Step 3: Simplify if possible.
Example A: Multiplying whole numbers with fractions.
Example A: Multiply 2 x 1/3
For our first example, we have to multiply the whole number 2 by the fraction ⅓, and we will do that by following our 3-step process as follows:
Step 1: Rewrite the whole number as a fraction with a denominator of 1.
First, we can rewrite the whole number, 2, as a fraction with a numerator of 1 as follows:
2 → 2/1
Now we have a new multiplication problem:
2 x 1/3 → 2/1 x 1/3
Step 2: Multiply the numerators together and then multiply the denominators together.
Now that we have a new expression with two fractions being multiplied by each other, we can perform multiplication by multiplying the numerators together and then multiplying the denominators together as follows:
2/1 x 1/3 = (2x1) / (1x3) = 2/3
After completing Step 2, we are left with the fraction 2/3.
Step 3: Simplify if possible.
Finally, we just have to check if our result from Step 2, 2/3, can be simplified.
In this case, the fraction 2/3 can not be simplified because there is no common factor between the numerator (2) and the denominator (3) other than 1.
Final Answer: 2 x 1/3 = 2/3
The complete step-by-step process for solving this first example is shown in Figure 02 below.
Figure 02: How to multiply fractions with whole numbers step-by-step.
Now that you are familiar with our 3-step method for multiplying fractions with whole numbers, let’s gain some more experience by using them to solve another example.
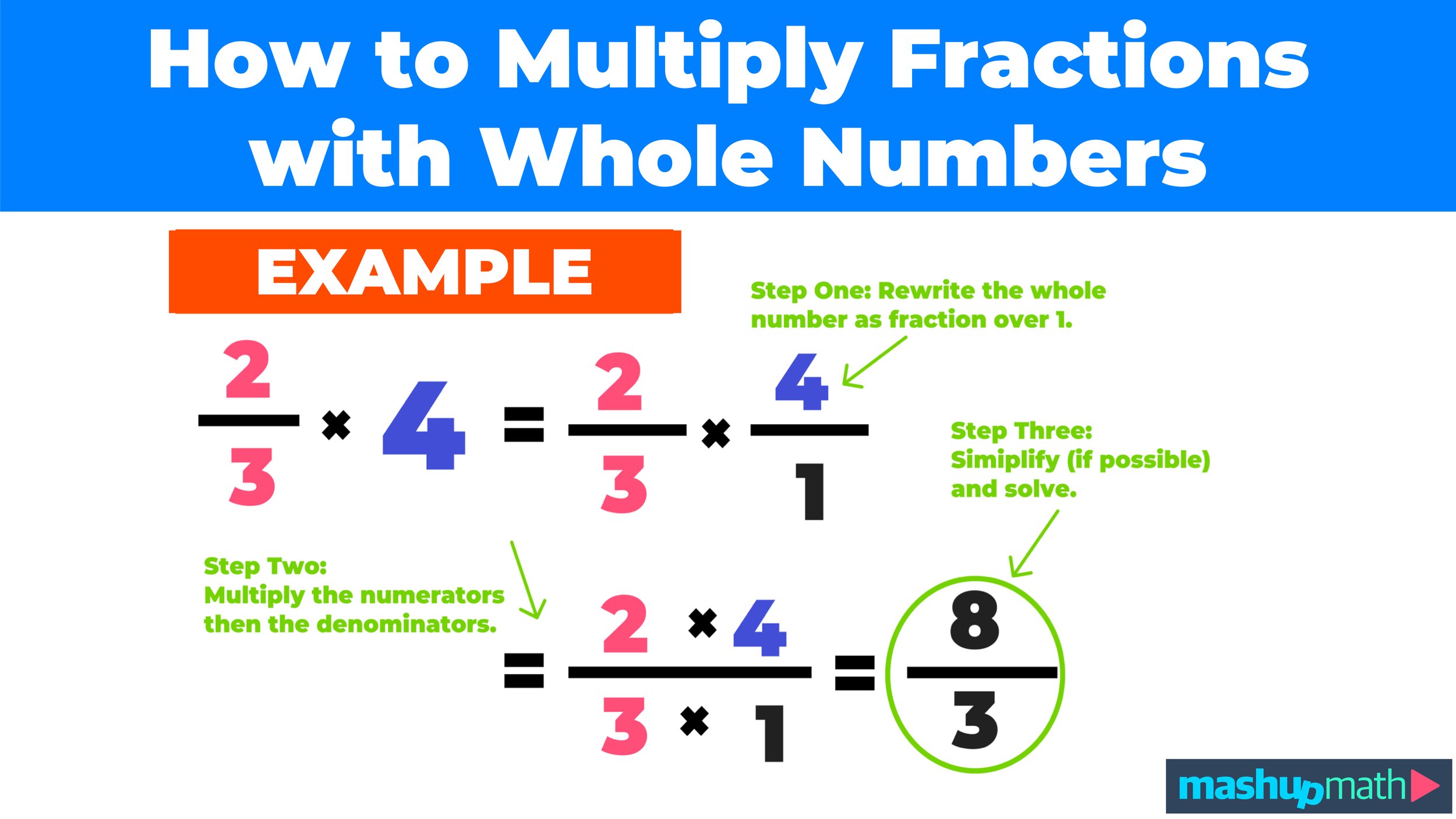
Example B: 2/3 × 34 = ?
Example B: Multiply 2/3 x 4
For this next example, notice how, in this case, the first term is a fraction and the second term is the whole number (this is a reverse situation compared to Example A). However, the commutative property of multiplication tells us that the order of the terms does not matter, so we can still use our 3-step process to solve this problem as follows:
Step 1: Rewrite the whole number as a fraction with a denominator of 1.
We can leave the fraction 2/3 alone and rewrite the whole number 4 as a fraction with a denominator of 1 as follows:
2/3 x 4 → 2/3 x 4/1
Step 2: Multiply the numerators together and then multiply the denominators together.
Next, we can take our new expression and simply multiply the numerators together, and then the denominators together as follows:
2/3 x 4/1 = (2x4) / (3x1)
(2x4) / (3x1) = 8/3
After completing the second step, our result is 8/3. We can now move onto the third and final step.
Step 3: Simplify if possible.
Let’s see if our result from Step 2, 8/3, can be simplified.
Since there is no common factor (other than 1) between the numerator (8) and the denominator (3), we know that the fraction 8/3 can not be simplified. However, since 8/3 is an improper fraction, we do have the option of either expressing it as 8/3 or as the mixed number 2 2/3 (in this case, we will choose to express our answer as 8/3).
Final Answer: 2/3 x 4 = 8/3
Figure 03 below illustrates our step-by-step process for solving this second example.
Figure 03: Multiplying fractions with whole numbers Example B solved.
5Example C: 10 × 1/5 = ?
Example C: Multiply 10 x 1/5
Let’s gain some more practice using our 3-step method for multiplying fractions with whole numbers.
Step 1: Rewrite the whole number as a fraction with a denominator of 1.
In this example, we have to rewrite the whole number (10) as a fraction with a denominator of 1.
10 x 1/5 → 10/1 x 1/5
Step 2: Multiply the numerators together and then multiply the denominators together.
Next, let’s take our new expression, 10/1 x 1/5, and multiply the numerators and denominators together:
10/1 x 1/5 = (10x1) / (1x5)
(10x1) / (1x5) = 10/5
Finally, let’s move onto Step 3 to see if our result (10/5) can be simplified.
Step 3: Simplify if possible.
In this case, the numerator (10) and the denominator (5) share a common factor of 5. So, we can simplify 10/5 by dividing both the numerator and denominator by 5 as follows:
10 ÷ 5 = 2
5 ÷ 5 = 1
After dividing, we can say that 10/5 = 2/1, and we can rewrite 2/1 as 2.
Final Answer: 10 x 1/5 = 10/5 = 2/1 = 2
The entire process of solving Example C is shown in Figure 04 below illustrates our step-by-step process for solving this second example.
Figure 05: Example C Solved.
Example D: 7 × 5/6 = ?
Example D: Multiply 7 x 5/6
Let’s work through one final example of multiplying fractions with whole numbers.
Step 1: Rewrite the whole number as a fraction with a denominator of 1.
In this case, we can rewrite the whole number (7) as a fraction as follows:
7 x 5/6 → 7/1 x 5/6
Step 2: Multiply the numerators together and then multiply the denominators together.
Next, we can multiply the two fractions together as follows:
7/1 x 5/6 = (7x5) / (1x6)
(7x5) / (1x6) = 35/6
Step 3: Simplify if possible.
Finally, we have to see if our result from Step 2 (35/6) can be simplified. Since 35 and 6 do not share any common factors besides 1, we know that it can not be simplified any further.
Final Answer: 7 x 5/6 = 35/6
Figure 06 shows how we solved this final example.
Figure 06: How to Multiply Fractions with Whole Numbers.
Looking for Free Math Worksheets?
Download hundreds of free PDF Math Worksheets with complete answer keys.
More Free Math Resources: