Viewing entries tagged
calculating percent increase

Log Rules Explained! (Free Chart)

Comment

Log Rules Explained! (Free Chart)

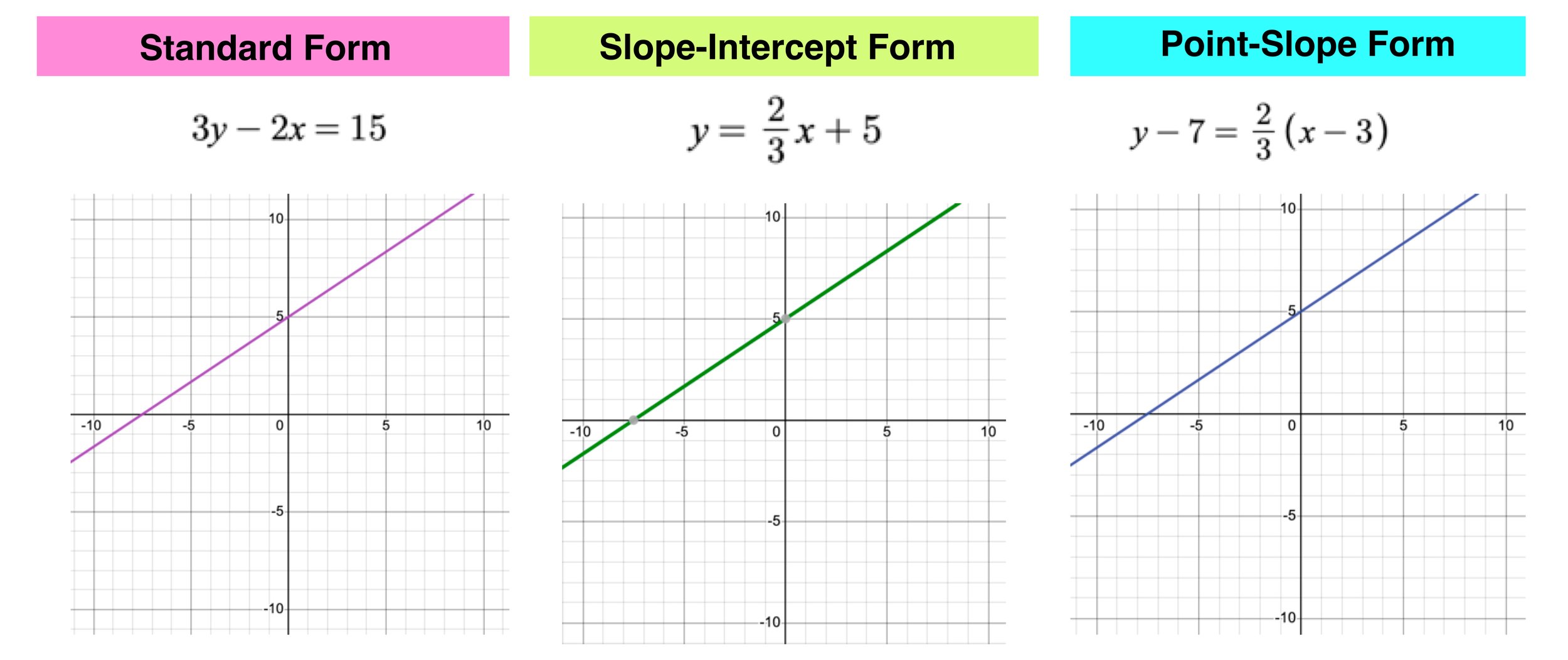
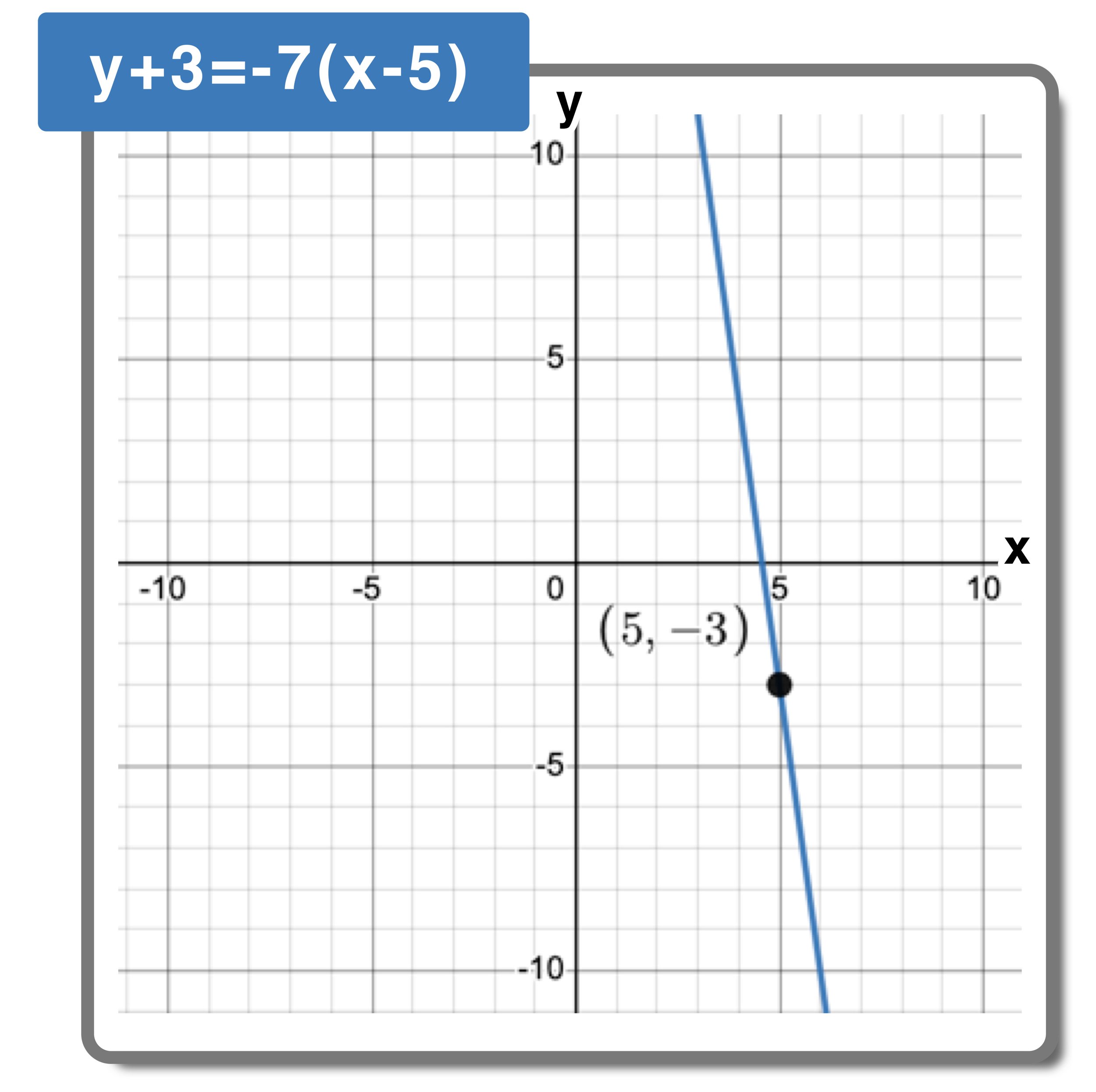
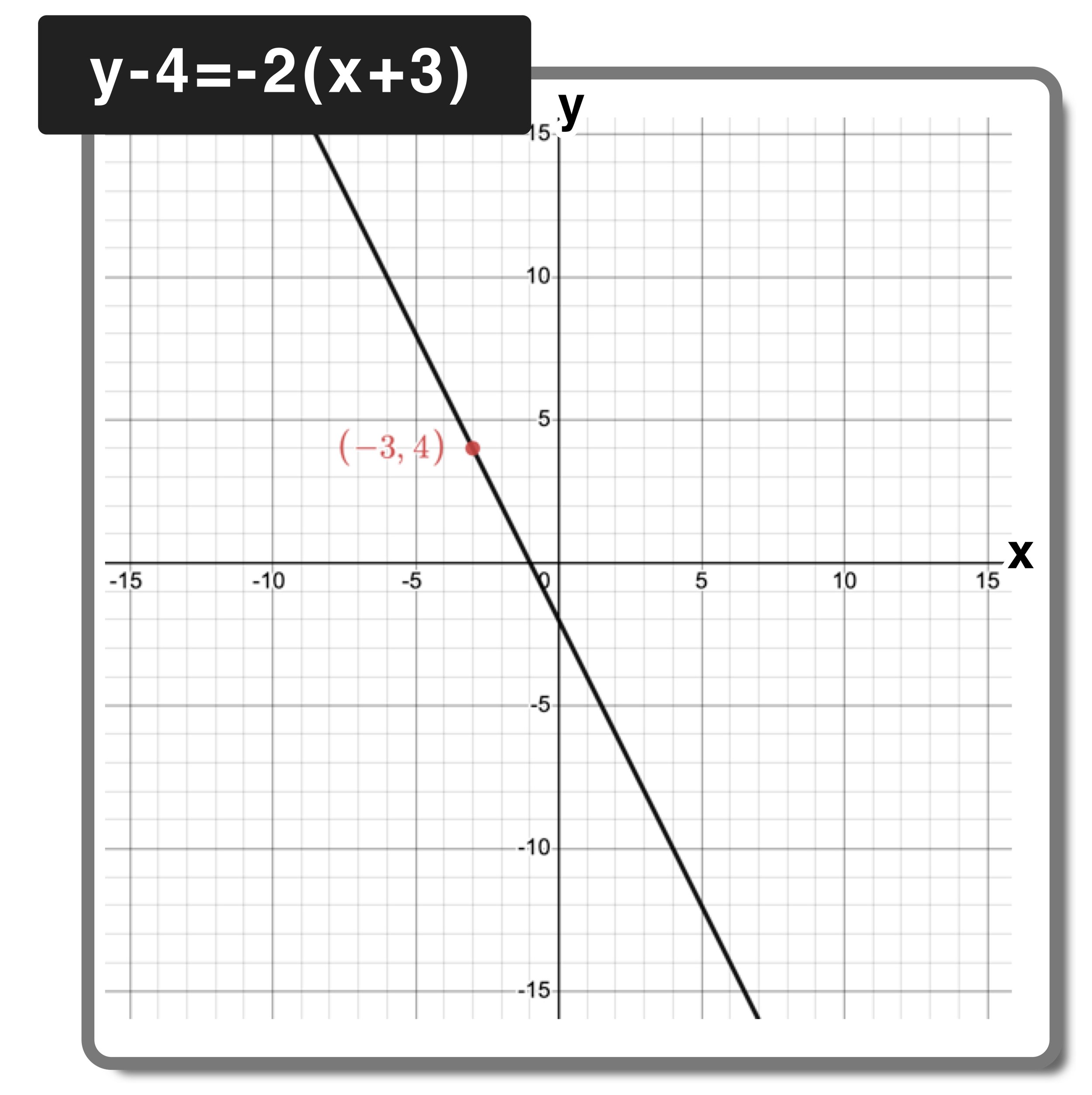
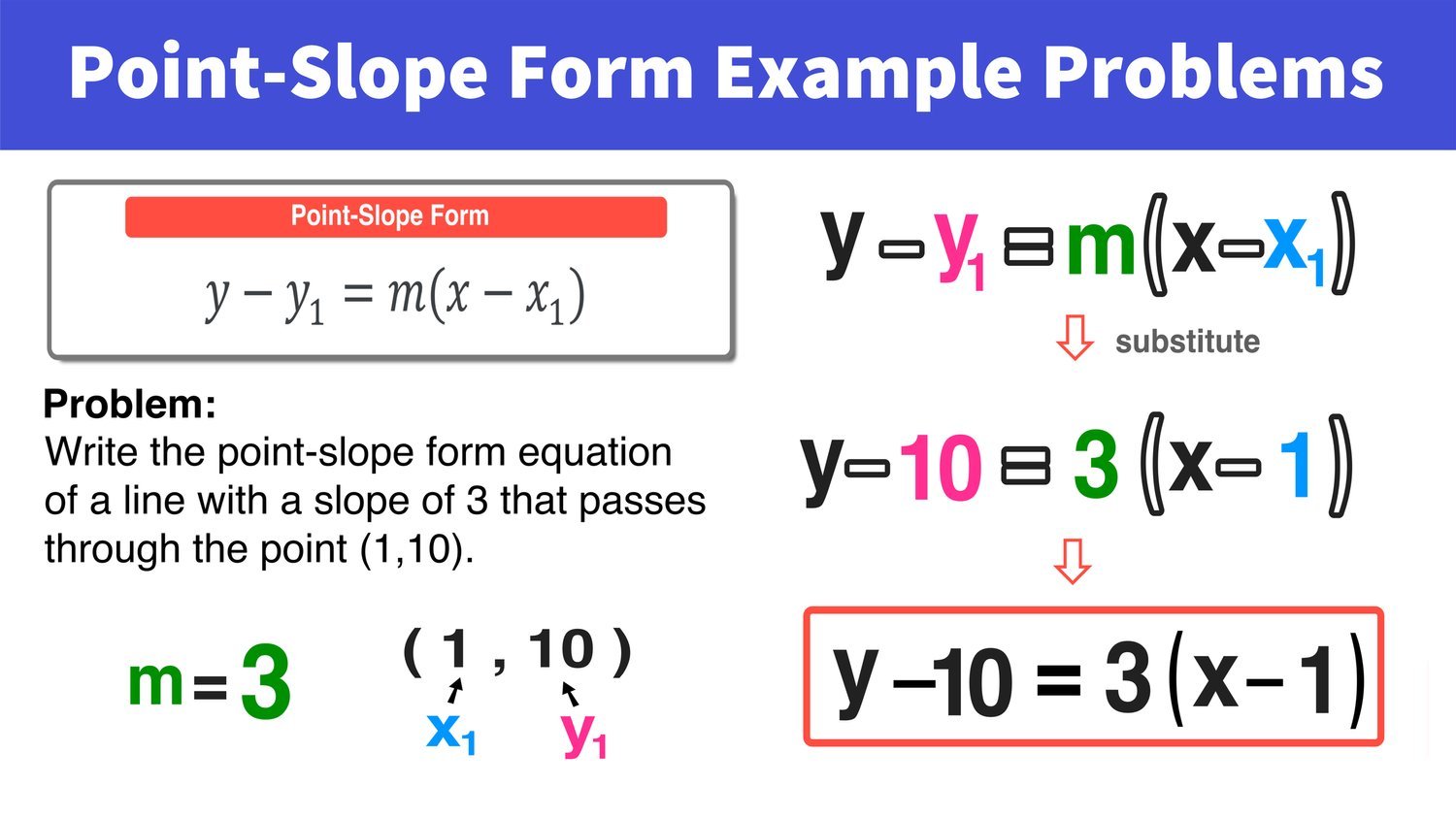
Everything you need to know about the natural log rules.

The Natural Log Rules Explained

In math, log rules (also known as logarithm rules) are a set of rules or laws that you can use whenever you have to simplify a math expression containing logarithms. Basically, log rules are a useful tool that, when used correctly, make logarithms and logarithmic equations simpler and easier to work with when solving problems.

Once you become more familiar with the log rules and how to use the, you will be able to apply them to a variety of math problems involving logarithms. In fact, understanding and remembering the log rules is essentially a requirement when it comes to working with logarithmic expressions, so understanding these rules is essential for any student who is currently learning about logarithms.

The following free guide to the Log Rules shares and explains the rules of logs (including exponent log rules), what they represent, and, most importantly, how you can use them to simplify a given logarithmic expression.

You have the option of clicking on any of the text links below to jump to any one section of this log rules guide, or you can work through each section in order—the choice is up to you!

When you reach the end of this free guide on the rules of logs, you will have gained a solid understanding of the natural log rules and how to apply them to solving complex math problems. Let’s get started!

 

Figure 01: What is the relationship between the logarithmic function and the exponential function?

 

Quick Review: Logarithms

While this first section is optional, we recommend that you start off with a quick recap of some key math concepts and vocabulary terms related to logs.

The first important thing to understand is that logarithmic functions and exponential functions go hand-in-hand, as they are considered inverses of each other. So, make sure that you have a strong understanding of the laws of exponents before moving forward.

Since the logarithmic function is an inverse of the exponential function, we can say that:

  • aˣ = M → logₐM = x

  • logₐM = x → aˣ = M

The log of any value, M, can be expressed in exponential form as the exponent to which the base value of the logarithm must be raised to in order to equal M.

Understanding this inverse relationship between the logarithmic function and the exponential function will help you to better understand the log rules described in the following sections of this guide.

Learning natural log rules shared in the next section will help you to break down complex log expressions into simpler terms, which is a critical skill when it comes to learning how to successfully work with logs, how to model situations using logs, and how to solve a variety of math problems that involve logs.

What are the Log Rules?

The natural log rules are set of laws that you can use to simplify, expand, or solve logarithmic functions and equations.

The chart in Figure 02 below illustrates all of the log rules. Simply click the blue text link below the chart to download it as a printable PDF, which you can use as a study tool and a reference guide.

The section that follows the log rules chart will share an in-depth explanation of each of the log rules along with examples.

 

Figure 02: The Natural Log Rules and the Change of Base Formula

 

Each of the following log rules apply provided that:

  • a≠1 and a>0, b≠1 and b>0, a=b, and M, N, and x are real numbers where M>0 and N>0

Log Rules: The Product Rule

The first of the natural log rules that we will cover in this guide is the product rule:

  • logₐ(MN) = logₐM + logₐN

 

Figure 03: The product rule of logarithms.

 

The product rule states that the logarithm a product equals the sum of the logarithms of the factors that make up the product.

For example, we could use the product rule to expand log₃(xy) as follows:

  • log₃(xy) = log₃x + log₃y

Pretty straightforward, right? The product rule of logarithms is a simple tool that will allow you to expand logarithmic expressions and equations, which often makes them easier to work with.

Log Rules: The Quotient Rule

The second of the natural log rules that we will cover in this guide is called the quotient rule, which states that:

  • logₐ(M/N) = logₐM - logₐN; or

  • logₐ(M➗N) = logₐM - logₐN

 

Figure 04: Natural Log Rules: The Quotient Rule

 

The quotient rule of logs says that the logarithm a quotient equals the difference of the logarithms that are being divided (i.e. it equals the logarithm of the numerator value minus the logarithm of the denominator value).

For example, we could use the quotient rule to expand log₇(x/y) as follows:

  • log₇(x/y) = log₇x - log₇y

Notice that the product rule and the quotient rule of logarithms are very similar to the corresponding laws of exponents, which should make sense because the logarithmic function and the exponential function are inverses of each other.

Log Rules: The Power Rule

The next natural log rule is called the power rule, which states that:

  • logₐ(Mˣ) = x ⋅ logₐM

 

Figure 05: Log Rules: The Power Rule

 

The power rule of logarithms says that the log of a number raised to an exponent is equal to the product of the exponent value and the logarithm of the base value.

For example, we could use the power rule to rewrite log₄(k⁸) as follows:

  • log₄(k⁸) = 8 ⋅ log₄k

The power rule of logarithms is extremely useful and it often comes in handy when you are dealing with the logarithms of exponential values, so make sure that you understand it well before moving forward.

Log Rules: The Zero Rule

Moving on, the next log rule on our list is the zero rule, which states that:

  • logₐ(1) = 0

 

Figure 06: Log Rules: The Zero Rule

 

Simply put, the zero rule of logs states that the log of 1 will always equal zero as long as the base value is positive and not equal to one.

For example, we could use the zero rule to rewrite log₂(1) as follows:

  • log₂(1) = 0

This simple rule can be very useful whenever you are trying to simplify a complex logarithmic expression or equation. The ability to zero out or cancel out a term can make things much simpler and easier to work with.

Log Rules: The Identity Rule

The fifth log rule on our list is called the identity rule, which states that:

  • logₐ(a) = 1

 

Figure 07: Natural Log Rules: The Identity Rule

 

The identity rule says that whenever you take the logarithm of a value that is equal to its base value, then the result will always equal 1 provided that the base value is greater than zero and not equal to one.

For example, we could use the identity rule to rewrite log₈(8) as follows:

  • log₈(8) = 1

Similarly, we could also use the identity rule to rewrite logₓ(x) as follows:

  • logₓ(x) = 1

Just like the zero rule, the identity rule is useful as it can sometimes help you with simplifying complex log expressions and equations.

Log Rules: The Inverse Property of Logs

The next log rule that we will cover in this guide is called the inverse property of logarithms rule, which states that:

  • logₐ(aˣ) = x

 

Figure 08: The inverse property of logs rule.

 

The inverse property of logs rule states that the log of a number raised to an exponent with a base value that is equal to the base value of the logarithm is equal to the value of the exponent.

For example, we could use the inverse property of logs rule to rewrite log₃(3ᵏ) as follows:

  • log₃(3ᵏ) = k

Again, this is another useful tool that you can use to simplify complicated log expressions and equations.

Log Rules: The Inverse Property of Exponents

The seventh log rule that we will cover is the inverse property of exponents rule, which states that:

  • a^(logₐ(x)) = x

 

Figure 09: Log Rule #7: The Inverse Property of Exponents

 

The inverse property of exponents log rule states whenever a base number with an exponent that is a logarithm equal to that base number, the result will equal the number in parenthesis.

For example, we could use the inverse property of exponents log rule to rewrite x^(logₓ(y²)) as follows:

  • x^(logₓ(y²)) = y²

Log Rules: The Change of Base Formula

The eighth and final log rule is the change of base formula, which states that:

  • logₐ(x) = (log꜀(x)) / (log꜀(a))

 

Figure 10: Log Rule: The Change of Base Formula

 

Conclusion: Natural Log Rules

In algebra, you will eventually have to learn how to simplify, expand, and generally work with logarithmic expressions and equations. The logarithm function is the inverse of the exponential function, and the corresponding log rules are similar to the exponent rules (i.e. they are a collection of laws that will help you to make complex log expressions and equations easier to work with). By studying and learning how to the natural log rules, you will be better able to understand logarithms and to solve difficult math problems involving logarithms. Feel free to bookmark this guide and return whenever you need a review of the rules of logs.

Keep Learning:

Comment

Exponent Rules Explained! (Free Chart)

4 Comments

Exponent Rules Explained! (Free Chart)

Everything you need to know about the exponent rules!

Exponent Rules Explained

In algebra, exponent rules (also known as the laws of exponents), are a set of laws that you can use to simplify mathematical expressions that have exponents. In other words, exponent rules can be used to make complicated math expressions containing exponents much easier to work with.

Once you learn the rules of exponents, you will be able to use them to quickly and easily perform operations (adding, subtracting, multiplying, dividing, etc.) on expressions that have exponents.

The following free guide to Exponent Rules will describe and explain the rules of exponents are, what they look like, and examples of how you can use them to simplify expressions and solve math problems involving exponents.

You can use the quick links below to jump to any section of this guide, or you can follow along step-by-step.

By the time that you reach the conclusion of this free guide on the rules of exponents, you will have a deep understanding of how to apply the exponent rules to simplify and solve math problems.

Are you ready to get started?

Before we start learning about the exponent rules, let’s do a quick review of exponents and cover some key vocabulary terms that you will need to be familiar with in order to make the most out of this guide.

 

Figure 01: The number 5 raised to a power of 3.

 

Quick Review: Exponents

In math, an exponent is a number or a variable at the top right of a base number or expression. The value of the exponent tells you what power the base number will be raised to.

For example, the expression 5³ has a base number of 5 and an exponent of 3. This expression means that the base number, 5, is being raised to a power of 3, meaning:

  • 5³ = 5 x 5 x 5 = 125

In short, an exponent tells you how many times to multiply the base number by itself.

Now that you understand the basic definition of an exponent, it’s time to move onto learning about the Exponent Rules.

What are the Exponent Rules?

The rules of exponents are a collection of specific ways to simplify math expressions involving exponents.

The chart in Figure 02 below shows all of the exponent rules. You can click the link below the chart to download a PDF copy which you can print out and use as a reference whenever you need to.

Below the chart, you will find a section that explains each individual exponent rule along with examples.

 

Figure 02: The Exponent Rules

 

Exponent Rules: Zero Exponent Rule

The first exponent rule described in this guide will be the zero exponent rule:

  • a⁰=1

 

Figure 03: The zero exponent rule.

 

The zero exponent rule states that any number, variable, or expression raised to the zero power is equal to 1.

For example:

  • 8⁰=1

  • x⁰=1

  • (a+b)⁰=1

Pretty simple, right?

To understand the zero exponent rule, you only have to remember that anything raised to the zero power is equal to one.

Exponent Rules: Product Rule

The next exponent rule that we will cover is called the product rule:

  • a^b x a^c = a^(b+c)

 

Figure 04: The product exponent rule.

 

The product rule of exponents applies whenever you have to multiply two or more expressions that have the same base.

The rule goes as follows: whenever you have to multiply two expressions with the same base value, you can simplify the expression by adding the exponents together and keeping the base value the same.

For example:

  • 4³ x 4² = 4⁵

  • m² x m² = m⁴

  • (a+b)⁵ x (a+b)³ = (a+b)⁸

Are you wondering where the product exponent rule comes from, let’s take a closer look at the example:

  • 4³ x 4² = 4⁵

We can rewrite each individual expression as follows:

  • 4³ = 4 x 4 x 4

  • 4² = 4 x 4

Therefore,

  • 4³ x 4² = 4 x 4 x 4 x 4 x 4 = 4⁵

The product rule allows you to simplify the expression 4³ x 4² as 4⁵. They both mean the same thing!

Before we move onto the next rule of exponents, note that the product rule only applies when the expressions have the same base value!

Exponent Rules: Quotient Rule

Our next stop on our chart of the rules of exponents is called the quotient rule.

The exponent rule comes into play when you have to divide two expressions with exponents that have the same base value.

The quotient rule of exponents goes as follows:

  • a^b ➗ a^c = a^(b-c); or

  • (a^b)/(a^c) = a^(b-c)

 

Figure 05(a): The quotient exponent rule with a division symbol.

Figure 05(b): The quotient exponent rule with a fraction.

 

Whether two expressions with the same base are being divided using a division sign (as shown in Figure 05(a)) or with a fraction (as shown in Figure 05(b)), you can simplify the expression by subtracting the second exponent from the first exponent and keeping the base value the same.

For example:

  • 8⁸ ➗ 8² = 8⁶

  • 8⁵ / 8² = 8³

  • n¹⁰ / n⁸ = n²

  • (a+b)⁹ ➗ (a+b)² = (a+b)⁷

Remember that the quotient exponent rule only applies if both expressions have the same base value.

Exponent Rules: Power of a Power Rule

What happens when you take an expression with an exponent and raise it to another power?

In case like this, you can use the power of a power exponent rule, which states that, whenever you have a base number, variable, or expression with an exponent raised to another exponent, the expression can be simplified by multiplying the two exponents together and keeping the base value the same.

  • (a^b)^c = a^(bc)

 

Figure 06: The power of a power exponent rule.

 

Whenever you have a single base with two exponents in a row, you can simplify the expression by multiplying the two exponents together, for example:

  • (9³)⁴ = 9¹²

  • (y²)² = y⁴

  • (x⁷)³ = x²¹

The power of a power exponent rule is a useful law of exponents that you can use to simplify complicated expressions involving multiple exponents.

Exponent Rules: Power of a Product Rule

What happens when you take an entire product and raise the entire thing to a power?

In cases like this, whenever you are raising a product of two numbers inside of a set of parentheses by an exponent, you can distribute the exponent and apply it to each term of the product as follows:

  • (ab)^c = a^b x a^c

 

Figure 07: The power of a product exponent rule.

 

In cases when your base is a product raised to an exponent, you can distribute the exponent to each term, for example:

  • (ab)³ = a³b³

  • (xy²)³ = x³y⁶

  • (m²n³)⁴ = m⁸n¹²

The power of a product exponent rule is one of the most important and use exponent rules on our list! Make sure that you feel comfortable with how to use it before moving onto the next law of exponents.

Exponent Rules: Negative Exponent Rule

The final exponent rule that we will cover in this guide is the negative exponent rule, which states that:

  • a^-b = 1/(a^b)

 

Figure 08: The negative exponent rule.

 

The negative exponent rule states that any number, variable, or expression raised to a negative power can be rewritten as a fraction with one in the numerator and the same original expression in the denominator, but with the negative sign removed from the exponent.

For example:

  • 8^-2 = 1/(8^2)

  • x^-7 = 1/(x^7)

  • (a+b)^-4 = 1/((a+b)^4)

That’s all that there is to it! If you ever have to solve a problem that requires you to give an answer that includes only positive exponents, the negative exponent rule will come in handy.

Conclusion: Exponent Rules

In math, you will need to be able to work with expressions that have exponents, and being able to simplify them quickly and correctly is an important skill. By learning and understanding the rules of exponents, you can easily simplify exponents in a variety of situations. Whenever you need to review the exponent rules, we encourage you to revisit this guide for a quick refresher.

Need More Help?

Check out our animated video lessons on the power to a power rule and the product to a power rule.

Keep Learning:

4 Comments

Calculating Percent Change in 3 Easy Steps

Comment

Calculating Percent Change in 3 Easy Steps

Calculating Percent Change in 3 Easy Steps

Learning how to calculate percent change is an immensely handy and essential mathematical skill that has various applications inside of your classroom and in the real world as well.

(Looking for a Percent Change Calculator to make a super fast calculation: Click here to access our free Percent Change Calculator)

The ability to calculate, comprehend, and analyze percentages does not only come in handy on class assignments, assessments, quizzes, exams, the SAT, etc., but also in practical matters associated with every day life and several careers as well. In fact, percentages is one of the most useful math skills outside of the classroom, so understanding them is should not be discounted or overlooked.

While it is common for students to think that calculating percent change can be a confusing and demanding task with many steps, the reality is that correctly solving any percent change problem is actually extremely simple and painless.

Are you ready to learn how to easily solve any percent change problem? This post shares a free Calculating Percent Change step-by-step lesson guide that will painlessly teach you how to calculate percent change using an uncomplicated three-step process. If you can learn how to follow the three-step process demonstrated in this guide, you will gain the ability to swiftly and flawlessly solve any math problems or exercises that require you to find percent change.

Now that we are just about ready to learn the three-step process for calculating percent change, lets quickly review a handful of essential vocabulary words, concepts, and definitions related to percentages and percent change.

Looking to learn how to calculate percent increase or percent decrease? Use the links below to download our free step-by-step guides:

Percent Definition

In terms of numeracy and mathematics, the word percent relates to parts per one hundred and the numerical symbol used to express percentages is %.

A simple example of a percent would be the value 60%. In this case, 60% represents 60 per 100.

Take a look at the diagram below. You should notice that the green shaded region makes up 60% of the whole.

Another way to express percent is to say that it is defined as a ratio of a value out of one hundred.

For another simple example, consider 30%. In this case, 30% is defined as 30 out of every 100. When viewing percentages as ratios of values out of one hundred, you can conclude that if 30% of 500 total people ride the train to work, then 150 total people ride the train—since 30 out of every 100 means that for every group of 100, we have to consider 30 people. So, 30 x 5 = 150 and 30% of 500 is 150.

Absolute Value Definition

To correctly calculate percent change, you will have to use absolute values. So, let’s do a quick review before we move on.

In math, the absolute value of a real number x, denoted using the vertical brackets |x|, is the positive (or non-negative) value of x.

The key thing to understand is that absolute values are always positive.

For example, the absolute value of 25 is equal to positive 25 AND the absolute value of -25 is equal to positive 25.

  • |25| = 25

  • |-25| = 25

This fact also applies to expressions inside of absolute value bars, for example:

  • | 5 - 7 | = | -2 | = 2

  • | 88 - 10 | = | 78 | = 78

  • | 0 - 33 | = | -33 | = 33

Again, notice how the result is always positive.

Percent Change Definition

Now you are ready to consider the question: what is percent change?

In math, the percent change between two values—a starting value and a final value—is simply the difference between those two values expressed as a percentage.

Note that percent change can also be referred to in terms of percent increase or percent decrease. These terms are more specific than percent change.

  • Percent Increase means that the final value is larger than the starting value. Final Value > Starting Value

  • Percent Decrease means that the final value is smaller than the starting value. Final Value < Starting Value

Note that percent change will be expressed as a number with a percentage (%) symbol attached to it.

As for identifying the starting value and the final value, simply look for the first value that is given and the second value that is given (percent change problems will always involve two values).

For example, if Chris drove 120 miles in September and 150 miles in October, and you wanted to calculate the percent change in miles driven between the two months, you would start by identifying that the starting value is 120 and the final value is 150.

 

Simple enough? If not, please review the previous section again as being able to correctly identify the starting value and the final value is critical for finding the solution to percent change math problems.

Calculating Percent Change

Are you ready to learn how to calculate percent change by apply our easy three-step process?

For our first example, we will continue with the scenario involving Chris and his monthly driving mileage totals.

Calculating Percent Change Example #1

In our first example, we will calculate the percent change for the following situation:

Chris drove 120 miles in September and 150 miles in October. What is the percent change in miles driven between the two months?

Remember that you already figured out that the starting value is 120 and the final value is 150.

Now, you are ready to apply the three-step process to calculating percent change. Here is a preview of how it will work:

 

Step 1: Find the absolute value of the difference.

To perform step one, simply take the absolute value of the difference of the starting value and the final value.

  • | Starting Value - Final Value | = ?

In this example:

  • | 120 - 150 | = | -30 | = 30

Again, since absolute value is involved, the end result of step 1 will always be a positive number.

Step 2: Divide the difference by the starting value.

The second step is to take your result from step 1 (30 in this example) and divide it by the starting value (120 in this example) as follows:

  • 30/120 = 0.25

For the second step, you will always express your result as decimal (never as a fraction). Otherwise, you will not be able to perform the third and final step.

Step 3: Multiply by 100

The last step is easy and straightforward: take your decimal result from step 2 and multiply it by 100, then express your final result using a % symbol as follows:

  • 0.25 x 100 = 25.0%

Final Answer: 25% Change (or 25% Increase)

Note that this final answer can also be considered a percent increase since the final value was larger than the starting value (the number of miles driven increased over time).

That’s all there is to it! Applying the three-step process allows you to conclude that there was a 25% change in miles driven between September and October.

Now, let's take a look at another calculating percent change example problem where you will gain more practice applying the three-step process.

Looking for a free Percent Change Calculator?

If you need a faster way to calculate the percent change between two numbers, check out our free Percent Change Calculator tool, which lets you input the starting and final values to get an instant answer!

▶ Click here to access our free Percent Change Calculator for students

Calculating Percent Increase Example #2

Last semester, Ariana spent a total of 107 hours studying for exams. This semester, she spent a total of 86 hours studying for exams. What was the percent change in the total number of hours she spent studying between last semester and this semester?

Let’s start by identifying the starting value and the final value:

  • Starting Value: 107

  • Final Value: 86

Step 1: Find the absolute value of the difference.

Just like Example #1, start by finding the absolute value of the difference of the starting value and the final value.

  • | 107 - 86 | = | 21 | = 21

Step 2: Divide the difference by the starting value.

The next step is to take your result from step 1 (21 in this example) and divide it by the starting value (107 in this example) as follows:

  • 21/107 = 0.1962616822… ≈ 0.196

Remember to express your result as decimal!

Step 3: Multiply by 100

Finally, take your decimal result from step 2 and multiply it by 100, then express your final result using a % symbol as follows:

  • 0.196 x 100 = 19.6%

Final Answer: 19.6% Change (or 19.6% Decrease)

Note that this final answer can also be considered a percent decrease since the final value was smaller than the starting value (the number of hours spent studying decreased over time).

All done! You have concluded that there was a 19.6% change in the total hours Ariana spent studying between last semester and this semester.

 

Now, let’s gain some more experience using the three-step process for calculating percent change by working through one final example.

Calculating Percent Decrease Example #3

In 2023, 395 students attended the Loha High School Dance. In 2024, 861 students attended the Loha High School Dance. What was the percent change in the number of students who attended the Loha High School Dance between 2023 and 2024?

We will start example #3 the same as the previous two examples, by identifying the starting value and the final value:

  • Starting Value: 395

  • Final Value: 861

 Just like the last two examples, you can solve this problem by following the three-step process:

Step 1: Find the absolute value of the difference.

Start by finding the absolute value of the difference of the starting value and the final value.

  • | 395 - 861 | = | -466 | = 466

Step 2: Divide the difference by the starting value.

The next step is to take your result from step 1 (466 in this example) and divide it by the starting value (395 in this example) as follows:

  • 466/395 = 1.179746835… ≈ 1.18

Step 3: Multiply by 100

Finally, take your decimal result from step 2 and multiply it by 100, then express your final result using a % symbol as follows:

  • 1.18 x 100 = 118.0%

Final Answer: 118% Change (or 118% Increase)

Note that this final answer can also be considered a percent increase since the final value was larger than the starting value (the number students in attendance increased over time).

Also note that it is totally fine to have an end result that is greater than 100%. In this case, a 118% increase means that the number of students in attendance more than doubled!

 

After working through three percent change examples, you should be feeling more confident in your ability to solve percent change problems using the three-step process. However, I highly recommend working through the examples again to further solidify your understanding so that you are successful on problems.

Conclusion: Calculating Percent Change

You can calculate percent change using a given starting value and final value by applying the following 3-step process:

Step 1: Find the absolute value of the difference between the starting value and the final value.

Step 2: Divide the result from Step 1 by the starting value and always express the result in decimal form.

Step 3: Multiply the result from Step 2 by 100 and express your final answer as a percentage (%).

What about Calculating Percent Increase and Percent Decrease?

Learn how to calculate a percent increase or a percent decrease between two numbers using our free step-by-step guides. Click the links below to get started.

Don’t forget about our Free Percent Change Calculator

Click here to get started using our free Percent Change Calculator

Keep Learning:

Comment

What is Point-Slope Form in Math?

Comment

What is Point-Slope Form in Math?

What is Point-Slope Form?

Arranging equations in a particular format gives us insight into specific features of an equation. The point-slope form is one such form used with linear equations and is useful when building an equation of a given straight line.

Let’s walk through what the point-slope form is, and learn its use cases with examples.

By the end of this lesson guide, you will be able to construct linear equations in the point-slope form and interpret features like slope, y-intercepts, and x-intercepts.

What are the different forms of linear equations?

A linear equation or an equation of a straight line can be represented in three main forms. These forms are namely:

  • Standard form

  • Slope-intercept form

  • Point-slope form

The major differences between these forms are the way that the properties of a linear equation are presented within the equation. The key properties that are involved in a straight line are the slope and its intercepts (y-intercept and/or x-intercept).

 The standard form, also known as the general form of linear equations, is Ax + By = C, where A, B, and C are constants. Using the standard form to represent a straight line makes it easier to obtain the y and x-intercepts using substitution.

The slope-intercept form is the most commonly used form and is of the form y = mx + b, where m and b are constants. The benefit of using the slope-intercept is that the slope and y-intercept of the straight line can be obtained directly by observing the equation, as m is the slope, and b is the y-intercept.

What is Point-Slope Form?

The point-slope form is another form in which a linear equation with two variables can be represented. As the name suggests, to construct an equation in the point-slope form we require a point on the straight line and its slope.

Definition: The point-slope form of a line is expressed using the slope of the line and point that the line passes through.

Formula: y-y1 = m(x - x1) where m equals the slope of the line and x1 and y1 equal the corresponding x- and y-coordinates of a given point the line passes through.

The general structure of an equation in the point-slope form is: y - y1 = m*(x - x1)

Here (x1,y1) is any arbitrary point on the straight line, and m is the slope/gradient of the straight line. Following is a table that shows a side-by-side comparison of the same straight line represented in the three forms mentioned above.

Again, notice each equation is a different way of expressing the same linear function, namely one with a slope of 2/3 and a y-intercept of 5.

One of the unique properties of the point-slope form compared to the other forms is the flexibility in its structure. This flexibility comes from the fact that you can use any point on a particular straight line to build the point-slope form equation.

In fact, below are some out of infinitely many other valid point-form equations of the straight line above.

  • y - 9 = ⅔*(x-6)

  • y - 3 = ⅔*(x+3)

  • y +1 = ⅔*(x+9)

 Next, let’s find out more about the fundamentals behind the point-slope form.

Fundamentals of the point-slope form

The main concept behind the point-slope form is the formula for determining the slope of a straight line. The formula that is used to calculate the slope of a straight line is :

m = (y2 - y1)/(x2 - x1)

Here, m is the slope of the straight line, while (x1,y1) and (x2, y2) are the coordinates of any two points on the given straight line.

Let’s apply the slope formula to find the slope of the line given in the graph below:

(y - y1)/ (x - x1) = m

Multiply both sides by x - x1, and we get

y - y1 = m * (x-x1), which is the general structure of the point-slope form.

As mentioned before, we can replace (x1,y1) with the coordinates of any point that lies on the given straight line. Note that if you select the y-intercept (b,0), then you will end up with the slope-intercept form of the equation.

y - b = m*(x-0)

y - b = mx

y = mx + b → the slope-intercept form

Therefore, the slope intercept can be considered a special case of the point-slope form.

Now with this fundamental understanding let’s see how we can construct the equation of a straight line in point-slope form using a variety of examples.

Point-Slope Form Examples

The information provided in a scenario can be different, but the goal is to determine the slope and the coordinates of a point that lies on a straight line.

Example #1 Finding the point-slope equation from slope and a point

Problem: Consider a straight line that has a slope of -7 and passes through the point (5, -3)

We can directly substitute the given data into the point-slope general equation below:

y-y1 = m*(x-x1)

Here m is the slope, hence it is -7 in this example. The point (5,3) is the arbitrary point (x1,y1). By substituting the terms we get:

y - - 3 = -7*(x - 5)

Hence,

y + 3 = -7*(x - 5) is the point-form equation of the straight line.

 

Example #2 Finding point-slope equation from two points

Problem: Consider a straight line on which the points (-2,1) and (1,10) lie. Determine the equation of the line in point-slope form.

 

First, we can use the slope formula to determine m (slope) of the line.

m = (y2- y1)/(x2-x1)

Substituting (-2,1) and (1,10) we get:

m = (10-1)/(1- - 2)

m = 9/3

m = 3

Now that we have determined the slope of the line to be 3, we can build the point-slope equation.

y-y1 = m*(x-x1)

We can pick any point on the line for (x1,y1). Here let’s use (-2,1).

y - 1 =3*(x- -2)

Hence,

y - 1 = 3*(x+2) is the point-form equation of the straight line.

 

Example #3 Determining features from the point-slope equation

Problem: Which of the following graphs represents the straight line of equation y - 4 = -2(x + 3)

 

The equation provided is in the point-slope form. By comparing y - 4 = -2(x+3) and y - y1 = m*(x-x1), we can work out that:

m = -2, and (x1,y1) is (4,-3)

So we must look for a graph that has a slope of -2 and passes through (4,-3). Since only black, red, and blue lines clearly pass through (4,-3), we can eliminate the rest.

 

Amongst the three, only the black has a negative slope. Hence the line that represents the point-slope equation of y - 4 = -2(x+3) is the black graph.

 

Looking for more point-slope form example problems?

Are you ready to extend your thinking and work through 5 more point-slope form practice problems with step-by-step explanations and answers included? Click the link below to access our free guide for students:

⇥ 5 Point-Slope Form Example Problems for Students

Conclusion: Point-Slope Form

Amongst the three main forms of representing linear equations, the point-slope form can be considered the most versatile. By identifying the slope and a single point that lies on a straight line you can determine the point-slope of an equation. The type of information you get about a straight line may change, hence you should utilize the necessary formulas and/or visual interpretations of graphs to extract what you need.

Keep Learning:

Comment

5 Point-Slope Form Examples with Simple Explanations

Comment

5 Point-Slope Form Examples with Simple Explanations

5 Point-Slope Form Examples for Students

When it comes to graphing lines on the coordinate plane, there are several different ways to express a linear equation and figure out how to determine a given line’s slope, y-intercept, points it passes through, and what its graph looks like. Being able to understand and solve problems involving linear equations in various forms, including point-slope form, is an immensely useful and important algebra skills that will help you to solve math problems involving linear equations.

The following lesson will take you step-by-step through solving 5 point-slope formula examples (sample problems) that we will solve and find the final correct answer together. By working through these 5 examples, you will gain a much better understanding of point-slope formula, what it means, and how to use it to solve problems on future homework assignments, quizzes, tests, and exams.

But, before we start working on the point-slope form examples, we will quickly review some very important vocabulary terms and review some key information regarding lines, equations, slopes, and y-intercepts. It is helpful to review this information, even if you are already familiar with it, as you will be building upon it as this lesson progresses and having a strong foundation makes a world of difference.

What is Point-Slope Form?

In math, there are more than one way to express the equation of a line (also referred to as a linear equation).

Most algebra students first learn about slope-intercept form: y=mx+b, where m equals the slope of the line and b equals the y-intercept.

With slope-intercept form, as long as you know the slope and y-intercept of the line, you can determine its equation, graph the line on the coordinate plane, and figure out what points the line passes through.

What is point-slope form?

Definition: The point-slope form of a line is expressed using the slope of the line and point that the line passes through.

Formula: y-y1 = m(x - x1) where m equals the slope of the line and x1 and y1 equal the corresponding x- and y-coordinates of a given point the line passes through.

Unlike slope-intercept form, point-slope form does not require you to know the function’s y-intercept. Instead, you are only concerned with the slope and the coordinates of one point that the line passes through.

If you find this definition confusing, take a look at the graphic below that compares slope-intercept form and point-slope form and further analyze the key similarities and differences between the two forms.

In terms of similarities, both slope-intercept and point-slope form require you to know the slope of the line.

(Looking to learn how to find the slope of a line? click here to access our free guide )

However, the key difference is that slope-intercept form requires you to know the y-intercept of the graph, while point-slope form requires you only to know the coordinates of one point that the graph passes through (this point can be anywhere on the line).

Now that you are familiar with the point-slope form formula, you are almost ready to work through some point-slope form examples. But, before we explore point-slope form further, let’s take a quick look two simple situations involving graphing linear functions using given information.

▶ Situation A: Graph the Linear Function

Problem: Graph a line with a slope of 3 and a y-intercept at 6 and write its equation in y= form.
Notice that Situation A gives us enough information right from the start to write the equation in y=mx+b form since we already know the slope, m, and y-intercept b.

  • m=3 and b=6

 We can write the equation as follows:

  •  y=3x+6

 This line has a slope of 3 (or 3/1) and a y-intercept at positive 6. We can now graph this line as follows:

 

Situation B▶: Graph the Linear Function

 Problem: Graph a line with a slope of 3 that passes through the point (2,12) and write its equation in y= form

Notice that situation B does not give us enough information to write the equation of the line in y= form since we only know the slope and not the y-intercept.

 This is where point-slope form comes into play!

In cases like this, m represents the slope, which is 3 and x1 and y1 are the corresponding x- and y-coordinates of whatever given point the line passes through.

Since we already know that the line passes through the point (2,12), we know that x1=2 and y1=12 

We can substitute these values into the point slope formula as follows

 

 After substituting m=3, x1=2, and y1=12, you end up with the following equation in point-slope form:

  • y-12 = 3(x-2) 

If we want to graph the line on the coordinate plane, it may be easier to rearrange this formula into y=mx+b form as follows:

 

To convert from point-slope form to slope-intercept form:

  • y-12=3(x-2) ➞ y-12=3x-6 ➞ y=3x+6

Now that we have converted the equation into y=mx+b form, we can go ahead and graph this line since we know that the slope is 3 and the y-intercept is positive 6.

 

Notice that the line does pass through the point (2,12).

What else do you notice about this graph? It turns out that situation A and situation B both represent the same linear equation, just in different forms.

  • Situation A: slope-intercept form

  • Situation B: point-slope form

If you are comfortable with solving problems involving equations in y=mx+b form (slope-intercept form), then you can surely be successful at solving problems involving point-slope form (y-y1=m(x-x1).

To take this next step, practice is key! So, now let’s look at 5 examples of solving algebra problems involving point-slope form to give you some more experience.

Point-Slope Form Example #1

Problem: Determine the point-slope form of a line that has a slope of 1/2 and passes through the point (8,2).

To determine the equation of this line in point-slope form, you have to know the following pieces of information:

  • the slope of the line, m

  • the coordinates of a point that the line passes through, (x1, y1)

In this first example, both of these pieces of information are given to you, so you are ready to write the equation of the line in point-slope form by substituting m=1/2, x1=8, and y1=2 as follows:

 

Answer: The equation of the line in point-slope form is: y-2=1/2x(x-8)

Point-Slope Form Example #2

Problem: Determine the point-slope form of a line that has a slope of 3/4 and passes through the point (4,-6).

Again, to determine the equation of this line in point-slope form, you have to know the following pieces of information:

  • the slope of the line, m

  • the coordinates of a point that the line passes through, (x1, y1)

Just like example #1, both of these pieces of information are given to you, so you are ready to write the equation of the line in point-slope form by substituting m=3/4, x1=4, and y1=-6 as follows:

 

In this example, the value of y1 is a negative number (-6). When you substitute this value into the point-slope formula, on the left-side of the equation, you end up with: y - - 6

You can simplify this double-negative by rewriting it as a positive: y- -6 ➞ y + 6

Answer: The equation of the line in point-slope form is: y+6=3/4x(x-4)

Point-Slope Form Example #3

Problem: Determine the point-slope form of a line that passes through the points (1,10) and (3,16)

Just like the previous two examples, to determine the equation of this line in point-slope form, you have to know the following pieces of information:

  • the slope of the line, m

  • the coordinates of a point that the line passes through, (x1, y1)

Unlike the first two examples, in this case you are not given all of the required information upfront. While you know that coordinates of a point that the line passes through (you actually know two of them), you do not know the slope of the line.

Luckily, you can use the slope formula to find the slope of a line that passes through two given points.

 

The slope formula is equal to change in y over change in x.

To correctly use the slope formula, you have to correctly label and identify the (x1, y1) and (x2, y2) values so you can substitute them into the formula. The first point that you use will have the 1’s and the second point that you identify will have the 2’s.

  • (1) First Point: (x1,y1) ➞ (1,10) where x1=1 and y1=10

  • (2) Second Point: (x2,y2)➞ (3,16) where x2=3 and y2=16

You are now ready to calculate the slope as follows:

 

By substituting the values of (x1,y1) and (x2,y2) into the slope formula, you end up with :

  • m = (16-10)/(3-1) = 6/2 = 3

After simplifying 6/2 to 3, you can conclude that the slope of the line, m, equals 3

Now that you know the slope of the line and the coordinates of a point that the line passes through, you can write the equation of the line in point-slope form. Note that you can choose either given point that the line passes through (1,10) or (3,16). Below, we will use the first given point (1,10):

 

Answer: The equation of the line in point-slope form is: y-10=3(x-1)

Point-Slope Form Example #4

Problem: Determine the point-slope form of a line that passes through the points (-5,15) and (-10,18)

Again, in order to determine the equation of this line in point-slope form, you have to know the following pieces of information:

  • the slope of the line, m

  • the coordinates of a point that the line passes through, (x1, y1)

Just like in example #3, you are not given all of the required information upfront. While you know that coordinates of a point that the line passes through, you do not know the slope of the line.

Following the same process as example #3, you can use the slope formula to determine the value of m as follows:

 

By substituting the values of (x1,y1) and (x2,y2) into the slope formula, you end up with :

  • m = (18-15)/(-10- -5)

Notice that the double negative in the denominator can be rewritten as: (-10- -5) ➞ (-10 + 5)

  • m = (18-15)/(-10 + 5) = 3/-5

You can express these results as -3/5, so you can conclude that the slope of the line, m, equals -3/5

Now that you know the slope of the line and the coordinates of a point that the line passes through, you can write the equation of the line in point-slope form. Just like the last example, you can choose either given point that the line passes through (-5,15) or (-10,18). Below, we will use the first given point (-5,15):

 

Answer: The equation of the line in point-slope form is: y-15=-3/5(x+1)

Point-Slope Form Example #5

Problem: Graph the line with the following point-slope form equation: y-4=2(x+6)

In this final example, you will have to graph a line and all that you are given is a linear equation in point-slope form.

All that you need to graph a line is the slope of the line and a point that the passes through—which is exactly what you can find when you look at the equation in point slope form.

Remember that point slope form is as follows: y-y1 = m(x = x1) where m equals the slope of the line and (x1,y1) are the corresponding x- and y-coordinates of the point the line passes through.

So, in this example, we can see that the slope of the line m=2 (or 2/1)

Furthermore, we can see that the y1=4, but what about x1?

Since we see that ‘+’ sign on the right-side of the equation (x+6), we know that a double-negative must have occurred, so the x1 value is equal to -6 (not positive 6).

Now we have all of the information that we need:

  • Slope: m=4

  • Point: (x1,y1) = (-6,4)

All that we have to do now is graph the line. Start by plotting the (x1,y1) point, which is (-6,4) in this example as follows:

 

Next, use the slope m=2/1 to “build” more points by using the rise over run method (rise two units, and run two units to the right) as follows:

 

Finally, draw the line that intersects the points that you plotted to complete your graph:

Answer:

 

Keep Learning:

Comment