Associative Property of Multiplication Explained in 3 Easy Steps

Associative Property of Multiplication Definition

In math, the associative property of multiplication is a rule which states that the groupings of values or variables being multiplied together does not affect the product or outcome.

The associative property of multiplication can help you to model and solve simple and complex multiplication problems. This rule is a fundamental law in mathematics and applies to any multiplication problem.

The following guide to understanding and applying the associative property of multiplication will share a step-by-step tutorial as well as a free associative property of multiplication worksheet.

Let’s start off by looking at a few examples…

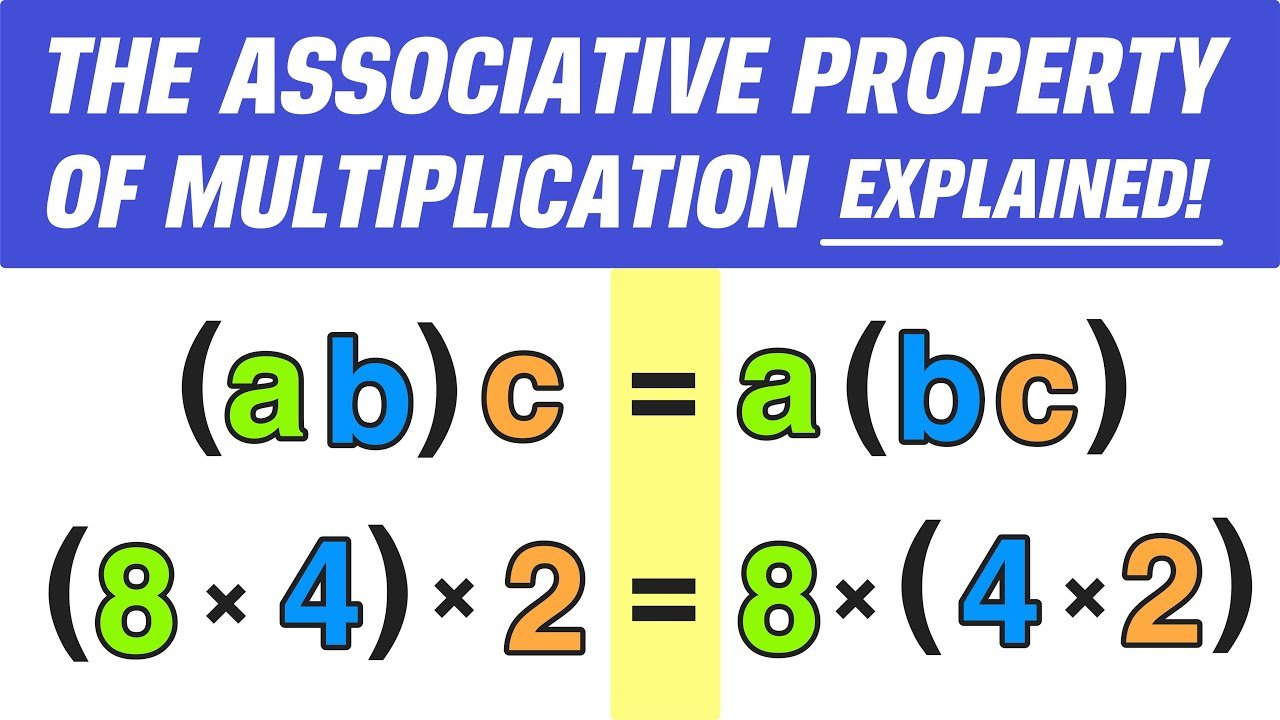
Associative Property of Multiplication Example

Take a look at the equation above:

(a x b) x c = a (b x c)

Notice that the terms (a, b, and c) are in the same order, but grouped differently. On the left side of the equals sign, a and b are in parenthesis. On the right side of the equal side, b and c are in parenthesis. And, according to the order of operations, you must perform operations inside of parenthesis first.

So, according to the associative property of multiplication, the left side of the equal sign and the right side of the equal sign will always equal the same value, no matter what values a, b, and c represent.

Now, let’s go ahead and explore why the associative property proves this equation to be true by substituting numerical values in for a, b, and c:

a=8, b=4, c=2

Step One: Follow the order of operations by performing multiplication inside of the parenthesis first.

In this example, perform (8 x 4) on the left side of the equation and (4 x 2) on the right side of the equation as follows:

(8 x 4 ) x 2 = 8 x (4 x 2)

32 x 2 = 8 x 8

Step Two: After working out the products inside of the parenthesis, the next step is to multiply the next line of the equation. In this case, 32 x 2 on the left side, and 8 x 8 on the right side.

32 x 2 = 8 x 8

64 = 64

Step Three: Verify your answer.

Remember that, according to the associative property of multiplication definition, the groupings of values or variables being multiplied together does not affect the product or outcome. Therefore, the left side of the equation and the right side of the equation should equal the same value.

In this example, the associative property holds true since both sides of the equation are equal to 64.

See Also: Check Out This Awesome Mean, Median, and Mode Activity

Associative Property of Multiplication Example Recap:

(8 x 4 ) x 2 = 8 x (4 x 2)

32 x 2 = 8 x 8

64 = 64 ✓

What About Division?

Now that you understand the associative property of multiplication, let’s see if the inverse of multiplication—division—is also associative.

What if we reused the associative property of multiplication example from before, but changed the multiplication signs to division signs?

Just like the last example, a=8, b=4, and c=2, the order of the terms is the same on both sides of the equal sign, but the terms are grouped differently. And also notice that the operation is no longer multiplication, but division.

Step One: Follow the order of operations by performing division inside of the parenthesis first.

In this example, perform (8 ÷ 4) on the left side of the equation and (4 ÷ 2) on the right side of the equation as follows:

(8 ÷ 4 ) ÷ 2 = 8 ÷ (4 ÷ 2)

2 ÷ 2 = 8 ÷ 2

Step Two: After working out the quotients inside of the parenthesis, the next step is to divide the next line of the equation. In this case, 2 ÷ 2 on the left side, and 8 ÷ 8 on the right side.

2 ÷ 2 = 8 ÷ 2

1 ≠ 4

Step Three: Verify your answer.

Remember that, the associative property states that different groupings of the terms in an equation should not change the result. In the first example, we proved that the associative property works for multiplication since both sides of the equation equaled the same number (64=64).

However, in the division example, we see that the left side of the equation and the right side of the equation result in different values. In this case, 1 and 4, which are obviously nit equal.

Therefore, in this example, the associative property does not hold true for division since both sides of the equation are not equal.

Associative Property of Division Example Recap:

(8 ÷ 4 ) ÷ 2 = 8 ÷ (4 ÷ 2)

2 ÷ 2 = 8 ÷ 2

1 ≠ 4 ✕

Conclusion: Associative Property Math Facts

The previous example has shown us that the associative property works for multiplication, but it does not work for division.

Therefore, as long as all of the terms are being multiplied (not divided), the groupings of values or variables being multiplied together does not affect the product or outcome.

So, (a x b) x c = a x (b x c) for any real number values represented by a, b, and c.

Key idea: The associative property of multiplication can be applied when the terms are in the SAME ORDER, but GROUPED DIFFERENTLY.

The Associative Property of Multiplication Video

Are you looking for a more in-depth and visual explanation of the associative property of multiplication? Check out our free YouTube video using the link below:

Tags: associative property of multiplication, associative property example, associative property of multiplication example, associative property of multiplication definition, associative property in math, associative law of multiplication, the associative property of multiplication

---

Have thoughts? Share your thoughts in the comments section below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

By Anthony Persico

Anthony is the content crafter and head educator for YouTube's MashUp Math. You can often find me happily developing animated math lessons to share on my YouTube channel . Or spending way too much time at the gym or playing on my phone.

More Free Math Resources You Will Love:

Number Bonds Explained: Free Worksheets Included

What is a Number Bond?

Number Bond Definition

What is a number bond? A number bond is a simple visual math aid that is used to show a given number can be represented as the sum of two numbers.

Do you want free K-8 math resources in your inbox every week? Click here to join our mailing list

Number Bond Example: 5

Let’s take a closer look at the number 5. You know that the number 5 can be thought of as the sum of 4 and 1, the sum of 3 and 2, or the sum of 5 and 0. In terms of number bonds, these different ways of expressing the number 5 as the composite sum of two numbers can be visually represented using number bonds as follows:

And, since addition is commutative, meaning that the order of the terms does not matter (e.g. 2+3=5 and 3+2=5), you can also express the above number bonds in reverse order as follows:

Looking to learn more about the commutative math property? Click here to access our free step-by-step guide

Now that you understand what number bonds are and what they represent, it’s time to explore why they are such a useful tool for helping your students to develop strong number sense and fluency with performing operations on numbers.

For starters, the visual nature of number bonds is an excellent way to help students to conceptualize numbers and how they can be expressed as a composite sum of two numbers. By understanding this composite nature of numbers, students are better equipped to perform mental math quickly and accurately and perform advanced operations.

 For example, imagine a student becoming familiar with the following number bonds for 10:

With this understanding, a student could easily and accurately solve an expanded addition problem by combining terms that equal 10 as follows:

Number Bond Example: Number Bonds to 10

Now that you understand the value of having your students practice and understand number bonds, you can start by focusing on number bonds to 10. The diagram below shows the number bonds to 10.

Free Number Bonds Worksheet

 Are you looking for free printable number bonds worksheets (with answer keys included) that will give your students plenty of practice with completing number bonds to 10? If so, use the links below to download your free pdf number bonds worksheet.

➔ Click here to download your free Number Bonds worksheet

Subtraction Number Bonds

Everything related to number bonds that we have covered so far have related to the fact that number bonds represent how a number can be expressed as the sum of two other numbers. But what about subtraction? We know that addition and subtraction share an inverse relationship. This inverse relationship can be explored via number bonds (note that this is an advanced step that should not be explored until your students have completely mastered the number bonds to 10 and possibly beyond.

Number Bond Example 03: Subtraction

For example, how could a student use her understanding of number bonds to solve the problem 10 - ___ = 7?

In this example, the student knows that one of the number bonds for 10 includes the number 7 and that the other number is 3 (because 7+3=10).

With this understanding in mind, it is a logical conclusion that 10 – 3 = 7 meaning that the missing number is 3.

This answer may seem extremely simple to find and you may even think that the use of a number bond is not even necessary. However, if we rewrite this problem as:

10 – X = 7 where x=3

We can see how understanding number bonds can apply to more advanced problems including high school level algebra.

Observe the diagram below that represents this application of subtraction number bonds:

Conclusion

Number bonds are simple visual tools that are used to show how a number can be represented as a composite sum of two other numbers. A number bond includes a whole number with two branches stemming from the number and leading to the two other numbers whose sum is equal to the original whole number. Number bonds are a valuable visual tool for helping students to practice and develop number sense, which is a critical foundational math skill that students will need to be successful at higher level of mathematics.

Do you want free K-8 math resources in your inbox every week? Click here to join our mailing list

Tags:  number bond, number bonds to 10, number bond example, number bonds worksheet, number bonds to 10 worksheet, number bonds to 5, number bonds kindergarten, subtraction number bonds, complete the number bond

---

Share your thoughts in the comments section below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

By Anthony Persico

Anthony is the content crafter and head educator for YouTube's MashUp Math. You can often find me happily developing animated math lessons to share on my YouTube channel . Or spending way too much time at the gym or playing on my phone.

More Free Math Resources You Will Love:

Box and Whisker Plots Explained in 5 Easy Steps

Box and Whisker Plot Definition

Source: Mashup Math MJ

A box and whisker plot is a visual tool that is used to graphically display the median, lower and upper quartiles, and lower and upper extremes of a set of data.

Box and whisker plots help you to see the variance of data and can be a very helpful tool.

This guide to creating and understanding box and whisker plots will provide a step-by-step tutorial along with a free box and whisker plot worksheet.

Let’s get started by looking at some basketball data!

How to Make a Box and Whisker Plot

Observe the following data set below that shares a basketball players points scored per game over a seven-game span:

Step One: The first step to creating a box and whisker plot is to arrange the values in the data set from least to greatest.

In this example, arrange the points scored per game from least to greatest.

Step Two: Identify the upper and lower extremes (the highest and lowest values in the data set).

The lower extreme is the smallest value, which is 5 in this example.

The upper extreme is the highest value, which is 32 in this example.

Step Three: Identify the median, or middle, of the data set.

In this example, the median is 17.

See Also: Check Out This Awesome Mean, Median, and Mode Activity

Step Four: Identify the upper and lower quartiles.

To find the lower quartile and the upper quartile, start by splitting the data set at the median into lower and upper regions.

The upper quartile is the median of the upper region, and the lower quartile is the median of the lower region.

In this example, the upper quartile is 20 and the lower quartile is 10.

Now we have all of the information that we will need to construct our box and whisker plot!

Step Five: Construct the Box and Whisker Plot

To construct a box and whisker plot, start by drawing a number line that fits the data set.

Start by plotting points over the number line at the lower and upper extremes, the median, and the lower and upper quartiles.

Next, construct two vertical lines through the upper and lower quartiles, and then constructing a rectangular box that encloses the median value point.

Then construct a vertical line through the median point that extends to the top and bottom of the rectangle.

This is the box in the box and whisker plot.

Finally, draw horizontal lines that connect the lower quartile to the lower extreme and the upper quartile to the upper extreme to complete the box and whisker plot.

The box and whisker plot is complete!

Box and Whisker Plot Worksheet

Are you looking to get some more practice with making and analyzing box and whisker plots?

Check out the following free box and whisker plot worksheet, which is available as a PDF download!

Click here to download your free Box and Whisker Plot worksheet.

Answer key included.

Box and Whisker Plot Video Lesson

Check out our free Box and Whisker Plots Explained video lesson on YouTube for a more in-depth look:

Tags:  box and whisker plot explained, box and whisker plot definition, box and whisker plot problems, box and whisker plot outliers, box and whisker plot worksheet, box and whisker plot range

---

Have thoughts? Share your thoughts in the comments section below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

By Anthony Persico

Anthony is the content crafter and head educator for YouTube's MashUp Math. You can often find me happily developing animated math lessons to share on my YouTube channel . Or spending way too much time at the gym or playing on my phone.

Related Posts: