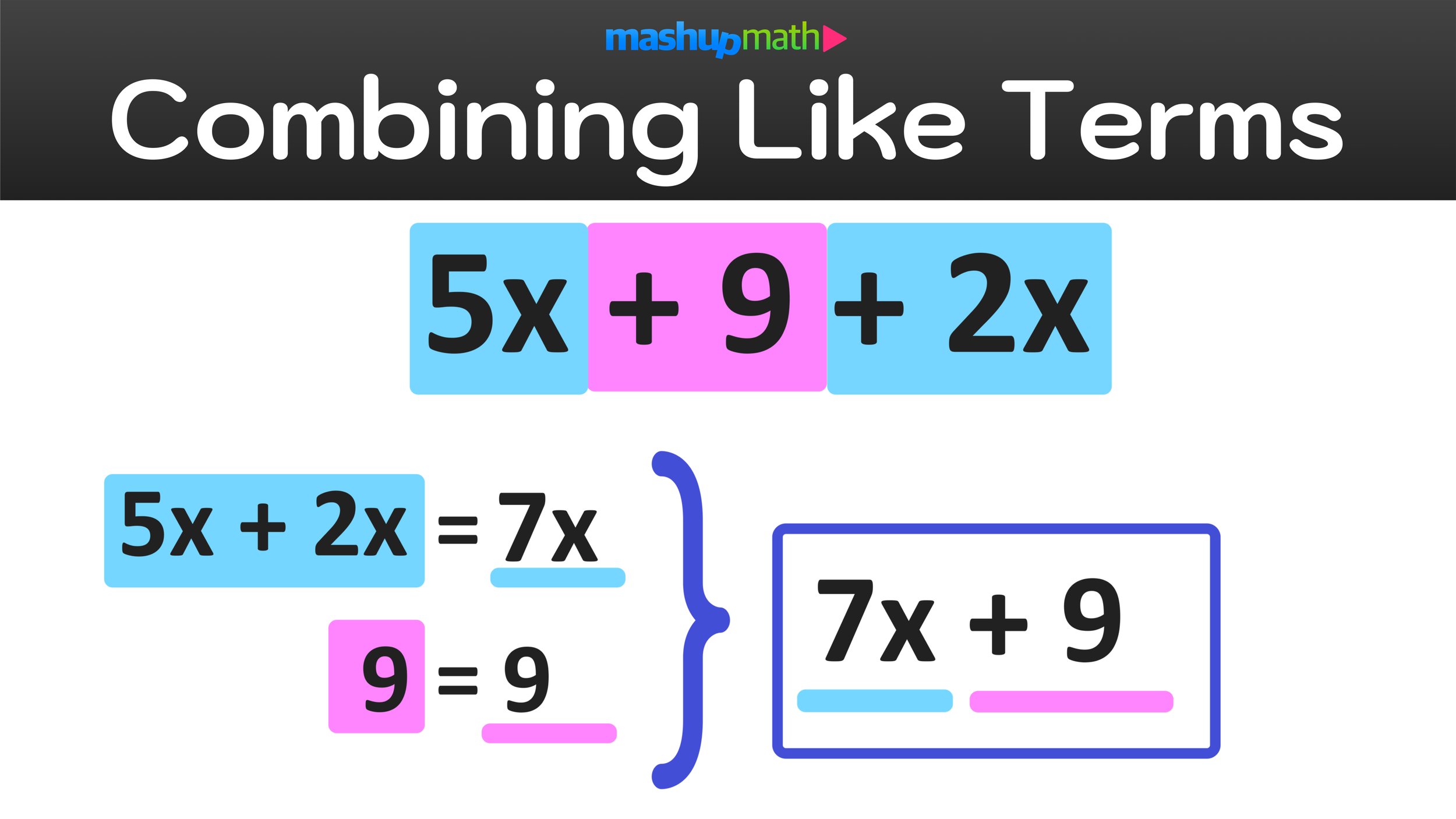
How to Find Perimeter in 3 Easy Steps

How to Find Perimeter in 3 Easy Steps

Math Skills: Learn how to find the perimeter of a rectangle, square, triangle, parallelogram, and circle.

Free Step-by-Step Guide: How to Find the Perimeter of a Shape.

Are you ready to learn how to find perimeter of various shapes and figures?

This free guide will teach you all about perimeter and how to find the perimeter of several common shapes. You can use the quick links below to jump to any section of this guide:

• Review: How to Find Perimeter

• Example: How to Find the Perimeter of a Square

• Example: How to Find the Perimeter of a Rectangle

• Example: How to Find the Perimeter of a Triangle

• Example: How to Find the Perimeter of a Parallelogram

• Example: How to Find the Perimeter of a Circle

In math, every student must learn how to find the perimeter of a two-dimensional shape. As long as you understand what perimeter means, you can easily find the perimeter of a shape by following a few easy steps.

For each example of how to find perimeter in this guide, we will use a simple 3-step method for finding perimeter that you can use to find the perimeter of any shape and to solve any math problem involving perimeter. As long as you can follow the three simple steps shared in this guide, you will always be able to find perimeter.

Let’s get started with a quick review of perimeter including some key vocabulary terms as well as an explanation of how to find perimeter before we move onto several specific examples.

How to Find Perimeter: Quick Review

Before you can learn how to find perimeter, you should be familiar with some important math concepts and vocabulary terms, which we will quick review here in this first section.

What is Perimeter?

Definition: In math, the perimeter of a shape is the total distance around the outer boundary of the shape.

Another way to describe the perimeter of a figure is the total measured length of the outline of the shape. Note that perimeter applies to two-dimensional shapes including triangles, rectangles, squares, etc.

For example, consider an equilateral triangle where each side has a length of 5 centimeters. To find the perimeter of the triangle, you would simply have to add up the lengths of all three sides as follows:

• 5 + 5 + 5 = 15 cm

Therefore, we could say that the triangle has a perimeter of 15 cm.

We can also think of perimeter as taking all of the side lengths of a shape and laying them out as one long straight line. The total length of this line would be the perimeter of the figure. This idea is illustrated in Figure 01 below.

Figure 01: How to Find Perimeter Explained.

On a larger scale, we can imagine a rectangular shaped park with a paved sidewalk surrounding it. The perimeter of the park would be the total distance that you would have to walk to travel the complete outer distance of the park (i.e. how far you would have to walk along the sidewalk to go around the park and end up back where you started.)

We can say that perimeter is the total distance around the outer boundary of a two-dimensional shape or figure.

Also, note that, unlike area, perimeter is only concerned with the outer boundary of a figure (not the inside of a figure).

Figure 02 below further illustrates the idea that the perimeter of a figure is the length of its outer boundary.

Figure 02: Perimeter is the total distance around the outer boundary of a two-dimensional shape or figure.

Now that you understand the concept of perimeter, you are ready to learn how to find a perimeter of several common two-dimensional shapes including squares, rectangles, triangles, parallelograms, and circles.

---

How to Find the Perimeter of a Square

Example #1: Find the Perimeter of a Square

For our first example, we have to find the perimeter of a square with a side length of 8m, as shown in Figure 03 below.

Figure 03: How to Find the Perimeter of a Square

We can solve this first example of how to find the perimeter of a square (and all of the examples in this guide) by following the following three steps:

• Step #1: Identify the Shape

• Step #2: Identify all of the side lengths and add them together

• Step #3: Determine the perimeter and use appropriate units of measurement

Let’s go ahead and apply these three steps to this first example of how to find perimeter of a square.

Step #1: Identify the Shape

Our first step is super easy. We know that the figure in question is a square with four sides that all have the same length (8m in this case).

Step #2: Identify all of the side lengths and add them together

We know that each side of the square has a length of 8m, so we can add them together as follows:

• 8 + 8 + 8 + 8 = 32

Step #3: Determine the perimeter and use appropriate units of measurement

Finally, we can say that the length of the outer boundary of the figure is 32m and we can conclude that:

Final Answer: P=32m

Our procedure for solving this first example is illustrated in Figure 04 below.

Figure 04: How to find the perimeter of a square with a side length of 8m.

That’s all that there is to finding perimeter! In this example, we learned how to find a perimeter of a square, but the steps that we used can be used to find the perimeter of any two-dimensional shape, as you will see in the following examples.

---

How to Find the Perimeter of a Rectangle

Example #2: Find the Perimeter of a Rectangle

Figure 05: How to find the perimeter of a rectangle

In this next example, we will learn how to find the perimeter of a rectangle with a length of 12 ft and a width of 7 ft.

Just like the previous example, we can find the perimeter of this rectangle by using our three step strategy as follows:

Step #1: Identify the Shape

We already know that the shape in question is a rectangle that has four sides (two lengths and two widths). We also know that the opposite sides have equal measure, meaning that the two lengths equal 12 feet each and the two widths equal 7 feet each.

In many cases, all four sides of the figure will not be labeled, but you may find it helpful to go ahead and label them as shown in Figure 06 below:

Figure 06: How to Find Perimeter of a Rectangle: It can be helpful to label all four side lengths (opposite sides of a rectangle have congruent lengths).

Step #2: Identify all of the side lengths and add them together

Now that we have labeled all four side lengths, we can add them together as follows:

• 12 + 12 + 7 + 7 = 38

Step #3: Determine the perimeter and use appropriate units of measurement

And now, for the final step, we can say that the perimeter of the rectangle is 38 feet, and we can conclude that:

Final Answer: P=38 ft

The diagram in Figure 07 below summarizes how we found the perimeter of the rectangle in this example.

Figure 07: The rectangle has a perimeter of 38 feet.

Now, let’s work through one more example of how to find perimeter of a rectangle.

---

Example #3: Find the Perimeter of a Rectangle

Figure 08: How to Find the Perimeter of a Rectangle

Notice that the rectangle whose perimeter we have to find in Figure 08 above has decimal side lengths.

This, however, will not change how we solve this problem and we can again use our three step strategy as follows:

Step #1: Identify the Shape

Since the shape in this example is a rectangle, we know that it has four sides, two of which have a length of 4.7 mm and two of which have a length of 8.1 mm.

Step #2: Identify all of the side lengths and add them together

Next, we can go ahead and find the sum of all four side lengths as follows:

• 4.7 + 4.7 + 8.1 + 8.1 = 25.6

Step #3: Determine the perimeter and use appropriate units of measurement

Now we can conclude that the rectangle has a perimeter of 25.6 mm.

Final Answer: P=25.6 mm

The diagram in Figure 09 below illustrates how we found the perimeter of a rectangle with a length of 4.7 and a width of 8.1.

Figure 09: How to Find the Perimeter of a Rectangle in 3 Easy Steps

---

How to Find the Perimeter of a Triangle

In this next section, we will focus on two examples of how to find the perimeter of a triangle. Unlike the last two sections where we focus on squares and rectangles, triangles only have three sides and they do not necessarily have right angles. However, these differences will not prevent us from finding the perimeter of a triangle using our three step strategy, as you will see in the examples below.

Example #4: Find the Perimeter of a Triangle

Figure 10: How to Find the Perimeter of a Triangle Example

For this next example of how to find the perimeter of a triangle, we can again use our three step strategy to find the solution.

Step #1: Identify the Shape

In this example, we want to find the perimeter of a triangle (a three-sided shape). In this example, our triangle has side lengths of 30, 64, and 68.

Note that the triangle in this example, as shown in Figure 10 above, already has all three side lengths labeled for us.

Step #2: Identify all of the side lengths and add them together

For the second step, we have to add all three side lengths together as follows:

• 30 + 64 + 68 = 162

Step #3: Determine the perimeter and use appropriate units of measurement

Finally, we can say that the perimeter of the triangle is 162 units and we can conclude that:

Final Answer: P=162

The entire process for how to find the perimeter of a triangle is shown in Figure 11 below.

Figure 11: How to Find the Perimeter of a Triangle Explained

---

Example #5: Find the Perimeter of a Triangle

Figure 12: How to Find Perimeter of a Triangle

Here is another example of finding the perimeter of a triangle (in this case, a right triangle). Let’s go ahead and use our three step strategy to find the solution.

Step #1: Identify the Shape

Even though this example features a right triangle, we still know the side lengths to be 3.6, 4.8, and 6, so we have enough information to move onto the next step.

Step #2: Identify all of the side lengths and add them together

Now, we can find the sum of all three sides of the triangle as follows:

• 6 + 3.6 + 4.8 = 14.4

Step #3: Determine the perimeter and use appropriate units of measurement

From here, we know that the perimeter of the triangle is 14.4 units.

Final Answer: P=14.4

The steps for solving this problem on finding the perimeter of a triangle are in the diagram in Figure 13 below.

Figure 13: How to Find the Perimeter of a Triangle Explained

---

How to Find the Perimeter of a Parallelogram

Next, let’s take a look at an example of how to find the perimeter of a parallelogram.

Note that, in math, a parallelogram is a four-sided figure with two pairs of parallel sides where opposite sides are congruent in length. So, if you can find the perimeter of a rectangle or a square, then you can easily learn how to find the perimeter of a parallelogram.

Example #6: Find the Perimeter of a Parallelogram

Figure 14: How to Find the Perimeter of Parallelogram

Step #1: Identify the Shape

Notice that this example specifically asks us to find the perimeter of a parallelogram, so knowing the properties of parallelograms will be incredibly useful here (namely that the opposite sides of a parallelogram have congruent, or equal, lengths).

So, even though the parallelogram in the diagram shown in Figure 14 above only has two sides labeled, we actually have enough information to find the perimeter of the parallelogram.

Before we move onto the next step, let’s go ahead and label all four sides of the figure as shown in Figure 15 below.

Figure 15: Finding the Perimeter of a Parallelogram: Label all four side lengths.

Step #2: Identify all of the side lengths and add them together.

From here, we have a parallelogram with four side lengths: 40, 40, 54, and 54. For this second step to finding the perimeter of a parallelogram, we can find the sum of these four side lengths as follows:

• 40 + 40 + 54 + 54 = 188

Step #3: Determine the perimeter and use appropriate units of measurement

Now that we have found the sum of the four side lengths of the parallelogram, we can say that the perimeter equals 188 units.

Final Answer: P=188

The complete three step process of how to find the area of a parallelogram is illustrated in Figure 16 below.

Figure 16: How to find the perimeter of a parallelogram explained.

Now that we have learned how to find the perimeter of a parallelogram, let’s move onto the final section of this guide on how to find the perimeter of shapes by looking at circles.

---

How to Find the Perimeter of a Circle

For this last section, we will focus on how to find a perimeter of a circle.

All of the shapes that we have previously covered in this guide (squares, rectangles, triangles, and parallelograms) had straight side lengths. However, circles do not have any sides at all, so how can we possibly find the perimeter of a circle?

Figure 17: How to Find Perimeter of a Circle Using the Circumference Formula

Remember that the concept of perimeter refers to the length of the outer boundary of a figure. And, when it comes to circles, the term that refers to its outer boundary is called its circumference.

So, anytime you are wondering how to find the perimeter of a circle, you should really be wondering how to find the circumference of that circle!

And, to find the circumference of a circle, we will have to use the circumference of a circle formula:

• C=πd

In other words, the perimeter of a circle (i.e. the circumference of a circle) is equal to the product of π and its diameter.

With this in mind, let’s work through two examples of how to find the perimeter of a circle using the circumference formula.

*Note that in the examples below, we will use a calculator to make calculations using the exact value for π. However, you can approximate by as 3.14 to find estimated answers if you do not have access to a calculator will a π button.

Example #7: Find the Perimeter of a Circle

Figure 18: How to Find the Perimeter of the Circle Example

In the case of finding the perimeter of a circle, our three step strategy will not work.

In fact, you will rarely see questions that ask you to find the perimeter of a circle. Rather, they will more often require you to find the circumference of the circle, which is exactly what we will do to solve this problem.

Again, we will be using the formula C=πd where d is the length of the circle’s diameter, which, in this example, is 116 inches.

So, we can use the formula as follows:

• C = π x d

• C = π x 116

• C = 364.424747…

• C ≈ 364.4

For this example, we will round our answer to the nearest tenth and we can conclude that the perimeter of the circle is 364.4 inches.

Final Answer: P = 364.4 inches

Figure 19: How to Find Perimeter of a Circle Explained

Now, let’s move onto our very last perimeter example where you will gain more practice with how to find a perimeter of a circle.

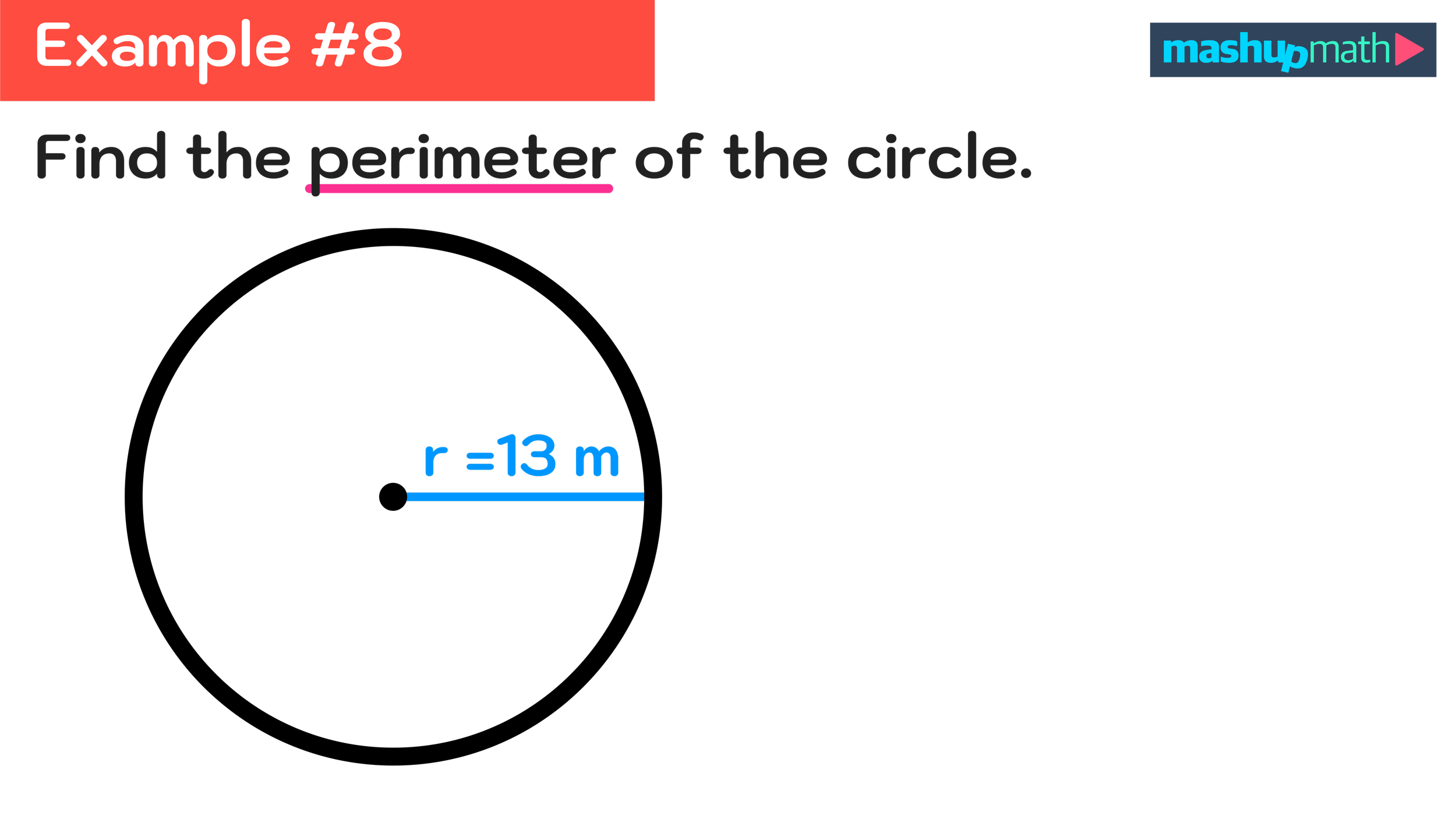
Example #8: Find the Perimeter of a Circle

Figure 20: How to find the perimeter of a circle with a radius of 13.

We can solve this example of finding the perimeter of a circle the same way that we did the previously one.

However, notice that this example only gives us the length of the circle’s radius and not its diameter.

To find the length of the radius, we simply have to double the length of the given radius (13 m) as follows:

• d = r x 2

• d = 13 x 2

• d = 26

Now that we know that the circle has a radius of 26m, we can use the circumference of a circle formula to find its perimeter as follows:

• C = π x d

• C = π x 26

• C = 81.6814089…

• C ≈ 81.7

Rounding our answer to the nearest tenth, we can conclude that the circle has a perimeter of approximately 81.7.

Final Answer: P = 81.7 m.

Figure 21: How to Find the Perimeter of a Circle Using the Circumference Formula.

---

Conclusion: How to Find Perimeter

In math, the perimeter of a shape is the total distance around the outer boundary of the shape. You can also think of perimeter as the measured distance along the outline of the shape.

In this guide on how to find perimeter, we worked through several examples of how to find the perimeter of a rectangle, a square, a triangle, and a parallelogram using the following three step strategy:

• Step #1: Identify the Shape

• Step #2: Identify all of the side lengths and add them together

• Step #3: Determine the perimeter and use appropriate units of measurement

We also looked at the special case of how to find the perimeter of a circle, where we used the circumference formula, C = π x d, the find the length of the outer boundary of any circle.

Finding the perimeter of a shape is a relatively easy math skill that you can learn through practice and understanding the meaning of perimeter and the procedure for finding it will help you to solve problems inside and outside of the math classroom.

Keep Learning

How to Find the Area of a Parallelogram in 3 Easy Steps

Learn how to find the area of any parallelogram in this free step-by-step guide.

---

Search Tags: how to subtract fractions, subtracting fractions, how to subtract fractions with different denominators, fraction subtraction, subtracting fractions with unlike denominators, how do you subtract fractions?

How to Multiply Fractions in 3 Easy Steps

How to Multiply Fractions in 3 Easy Steps

Math Skills: Learn how to multiply fractions by fractions, how to multiply fractions with whole numbers, and how to multiply mixed fractions.

Free Step-by-Step Guide: How to Multiply Fractions Explained

Once you have learned how to add fractions and how to subtract fractions, you are ready to start multiplying fractions in a variety of scenarios.

This free guide will teach you everything you need to know about how to multiply fractions in the following ways:

• How to Multiply Fractions by Other Fractions

• How to Multiply Fractions with Whole Numbers

• How to Multiply Mixed Fractions (or Mixed Numbers)

The task of having to multiply fractions together may seem challenging at first, but the procedure is actually quite simple and something that you can learn by working through a handful of practice problems, which is exactly what we will be doing in this free step-by-step guide.

While we highly recommend that you work through the guide from start to finish, you can use the quick-links above to jump to a particular topic section.

For all of the problems in this guide, we will be using an easy 3-step strategy that can be used to solve any problem where you have to multiply fractions to get a solution. So, if you can learn how to follow three easy steps, you can correctly answer any multiplying fractions problem!

Are you ready to get started?

Before we start working on the multiplying fractions practice problems, let’s do a quick recap of some key vocabulary terms related to fractions.

How to Multiply Fractions: Vocabulary Recap

This guide uses several math vocabulary terms related to fractions that you will need to be familiar with in order to learn this new math skill. Be sure that you understand the meaning of the following key vocabulary terms before moving forward in this guide.

The Numerator of a Fraction

For any fraction, the numerator is the number at the top of the fraction. For example, the fraction 4/5 has a numerator of 4 since 4 is at the top of the fraction.

The Denominator of a Fraction

For any fraction, the denominator is the number at the bottom of the fraction. For example, the fraction 4/5 has a denominator of 5 since 5 is at the bottom of the fraction.

In this guide, any mention of a numerator is referring to the top number of a fraction and any mention of a denominator is referring to bottom number of a fraction. This relationship between the numerator and the denominator of a fraction is illustrated in Figure 01-A below.

Figure 01-A: In any fraction, the numerator is the top number and the denominator is the bottom number.

Moving on, let’s recap three more key vocabulary terms and concepts related to fractions:

Whole Numbers

A whole number refers to any number that does not contain any fractions or decimals (i.e. an integer). Some examples of whole numbers include 4, 51, and 263.

Note that any whole number can be expressed in fraction form by rewriting it as a fraction with a denominator of 1. For example, the number 4 can be rewritten as a fraction as 4/1. This fact will come in handy later on when you learn how to multiply a fraction by a whole number.

Figure 01-B: How to Rewrite a Whole Number as a Fraction: Rewrite the given whole number as a fraction with a denominator of 1.

Proper Fractions

A fraction is a number that represents a part of a whole number. 1/4, 3/5, and 7/8 are all examples of fractions.

Note that proper fractions have a numerator that is smaller than the denominator.

Mixed Fractions (Mixed Numbers)

A mixed fraction (or a mixed number) is any number that represents the sum of a whole number and a proper fraction. For example, 7 5/8 is a mixed number that is equal to the sum of 7 and 5/8.

Note that mixed fractions can also be expressed as improper fractions (fractions whose numerator is greater than its denominator).

Figure 02: What is the difference between a whole number, a fraction, and a mixed number.

Now that you are familiar with these vocabulary terms, you’re ready to learn how to multiply fractions using our easy 3-step strategy.

---

How to Multiply Fractions by Fractions

How to Multiply Fractions by Fractions: Example #1

Example #1: 1/2 x 3/4

To solve this first example, where we are tasked with finding the product of 1/2 (one-half) and 3/4 (three-quarters), we will use the following three-step strategy for multiplying fractions:

How to Multiply Fractions in 3 Easy Steps

• Step One: Multiply the numerators together.

• Step Two: Multiply the denominators together.

• Step Three: See if the resulting fraction can be simplified or reduced.

When it comes to multiplying fractions, the process is extremely straightforward, and you can use these three steps to solve any fraction multiplication problem!

So, let’s go ahead and use these three steps to solve this first problem: 1/2 x 3/4 = ?

Step One: Multiply the numerators together.

For this problem, we have two fractions: 1/2 x 3/4

The first step requires us to multiply the two numerators (top numbers) together as follows:

• 1 x 3 = 3

Step Two: Multiply the denominators together.

The second step requires us to multiply the two denominators (bottom numbers) together as follows:

• 2 x 4 = 8

Step Three: See if the resulting fraction can be simplified or reduced.

Now we have a new fraction with a numerator of 3 and a denominator of 8:

• 1/2 x 3/4 = 3/8

For the final step, we have to see if the result can be further simplified or reduced. If it can’t, our result will be our final answer.

In this case, 3/8 can not be simplified or reduced any further and we can conclude that:

Final Answer: 1/2 x 3/4 = 3/8

Figure 03 below illustrates how we solved the first example using the three-step strategy.

Figure 03: How to Multiply Fractions: Multiply the numerators together, then multiply the denominators together. Finally, simplify the resulting fraction if possible and you’re done!

Note that it’s okay if you are still a little confused. The more experience you gain using this 3-step strategy to multiply fractions, the easier these type of problems will become. Let’s go ahead and work through another example.

---

How to Multiply Fractions by Fractions: Example #2

Example #2: 5/6 x 4/7

Let’s go ahead and solve Example #2 exactly the same as we did the previous example.

Step One: Multiply the numerators together.

For step one, we have to find the product of the two numerators, 5 and 4:

• 5 x 4 = 20

Step Two: Multiply the denominators together.

For step two, we have to find the product of the two denominators, 6 and 7:

• 6 x 7 = 42

Step Three: See if the resulting fraction can be simplified or reduced.

Our result is a fraction with a numerator of 20 and a denominator of 42:

• 5/6 x 4/7 = 20/42

Can the result, 20/42, be reduced? The answer is yes, because both numbers have a greatest common factor of 2. So, after dividing both the numerator and the denominator by 2, we can conclude that:

Final Answer: 5/6 x 4/7 = 20/42 = 10/21

Figure 04 below illustrates how we determined that 5/6 x 4/7 = 10/21

Figure 04: How to Multiply Fractions: Be sure to express your answer in reduced form.

Next, let’s go ahead and solve one more practice problem where we’ll have to multiply one fraction by another fraction.

---

How to Multiply Fractions by Fractions: Example #3

Example #3: 9/16 x 7/12

Again, we can use the same three-step strategy to solve this third example as follows:

Step One: Multiply the numerators together.

In this case, the numerator of the first fraction is 9 and the numerator of the second fraction is 7.

• 9 x 7 = 63

Step Two: Multiply the denominators together.

In this case, the denominator of the first fraction is 16 and the denominator of the second fraction is 12.

• 16 x 12 = 192

Step Three: See if the resulting fraction can be simplified or reduced.

Now we have a new fraction with a numerator of 62 and a denominator of 192:

• 9/16 x 7/12 = 63/192

To see if this result can be reduced, we have to see if 63 and 192 both share a GCF. Since both of these numbers are divisible by 3, we can divide both the numerator and the denominator by 3 to get our final answer in reduced form:

Final Answer: 9/16 x 7/12 = 63/192 = 21/64

The graphic in Figure 05 below shows we successfully multiplied two fractions in this example.

Figure 05: How to Multiply Fractions Step-by-Step.

Now that you have some experience with multiplying fractions, let’s move onto the next section where you will learn how to multiply a fraction by a whole number and how to multiple a whole number by a fraction.

---

How to Multiply Fractions with Whole Numbers

The second section of this guide will focus on how to multiply a fraction with by a whole number and how to multiply a whole number by a fraction.

Luckily, the three-step method that we used to solve problems in the previous section will work here as well. If you are unfamiliar with how to multiply a fraction by a fraction, we highly recommend that you go back and work through the above examples.

Now, let’s jump into our first example on how to multiply fractions with whole numbers!

How to Multiply a Fraction by a Whole Number: Example #1

Example #1: 3/8 x 2

When learning how to multiply fractions with whole numbers, it is important to remember that any whole number can be rewritten as an equivalent fraction by rewriting it as a fraction with a denominator of 1.

Since any whole number can be expressed as a fraction in this way, we can solve problems where you have to multiply a fraction by a whole number simply by rewriting the whole number as a fraction and then using the same 3-step strategy from the previous section to solve it.

For this example, we can rewrite the whole number, 2, as 2/1 and rewrite the original problem as:

• 3/8 x 2 → 3/8 x 2/1

Now, we can find the product by using our three steps:

Step One: Multiply the numerators together.

In this case, the numerator of the first fraction is 3 and the numerator of the second fraction is 2.

• 3 x 2 = 6

Step Two: Multiply the denominators together.

In this case, the denominator of the first fraction is 8 and the denominator of the second fraction is 1.

• 8 x 1 = 8

Step Three: See if the resulting fraction can be simplified or reduced.

Finally, we are left with a new fraction with a numerator of 6 and a denominator of 8:

• 3/8 x 2/1 = 6/8

And, since 6 and 8 are both divisible by 2, we know that the fraction 6/8 can be simplified to:

Final Answer: 3/8 x 2/1 = 6/8 = 3/4

Figure 06 illustrates how we solved this problem.

Figure 06: How to Multiply a Fraction by a Whole Number: Rewrite the whole number as a fraction with a denominator of 1.

Next, let’s take a look at another example of how to multiply fractions by whole numbers.

---

How to Multiply a Fraction by a Whole Number: Example #2

Example #2: 5/8 x 6

For this next practice problem, we can rewrite the whole number, 6, as 6/1 and rewrite the original problem as:

• 5/8 x 6 → 5/8 x 6/1

From here, we can solve this problem as follows:

Step One: Multiply the numerators together.

Start by multiplying the numerators of both fractions together:

• 5 x 6 = 30

Step Two: Multiply the denominators together.

Next, continue with multiplying the denominators of both fractions together:

• 8 x 1 = 8

Step Three: See if the resulting fraction can be simplified or reduced.

Here we have a resulting fraction with a numerator of 30 and a denominator of 8:

• 5/8 x 6/1 = 30/8

Notice that this result is an improper fraction since the numerator, 30, is greater than the denominator, 8. We can reduce this result down to 15/4 and leave it as our final answer or we can convert 15/4 to a mixed number, 3 3/4.

Final Answer: 5/8 x 6/1 = 30/8 = 15/4 or 3 3/4

The graphic in Figure 07 shows how we solved solve this problem by multiplying fractions by whole numbers.

Figure 07: How to Multiply a Fraction by a Whole Number Step-by-Step.

---

How to Multiply a Whole Number by a Fraction: Example #3

Example #3: 9 x 2/3

Notice that this third example requires you to multiply a whole number by a fraction (rather than a fraction by whole number like in the last two examples). Since multiplication is associative, the order of the terms won’t change the way that you solve the problem, so we can again use our three-step strategy as follows:

First, just like the previous two examples, rewrite the whole number (9 in this case) as a fraction with a denominator of 1.

• 9 x 2/3 → 9/1 x 2/3

Step One: Multiply the numerators together.

Multiply the numerators together as follows:

• 9 x 2 = 18

Step Two: Multiply the denominators together.

Multiply the denominators together as follows:

• 1 x 3 = 3

Step Three: See if the resulting fraction can be simplified or reduced.

The resulting fraction has a numerator of 18 and a denominator of 3:

• 9/1 x 2/3 = 18/3

The resulting improper fraction, 18/3, can be simplified. Since both 18 and 3 share a GCF of 3, we can perform 18/3 = 6/1 = 6 and conclude that

Final Answer: 9/1 x 2/3 = 18/3 = 6

All of the steps for solving this example are shown in Figure 08 below.

Figure 08 How to Multiply a Whole Number by a Fraction Step-by-Step

---

How to Multiply a Whole Number by a Fraction: Example #4

Example #4: 12 x 7/8

For this next example, we have to rewrite the whole number (12 in this case) as a fraction with a denominator of 1 and then use our three-step strategy to solve:

• 12 x 7/8 → 12/1 x 7/8

Step One: Multiply the numerators together.

For step one, multiply both of the numerators:

• 12 x 7 = 84

Step Two: Multiply the denominators together.

For step two, multiply both of the denominators:

• 1 x 8 = 8

Step Three: See if the resulting fraction can be simplified or reduced.

The result is a new fraction with a numerator of 84 and a denominator of 8:

• 12/1 x 7/8 = 84/8

Now we have to see if 84/8 can be simplified. Both 84 and 8 share a GCF of 4, so after dividing both numbers by 4, the result is 21/2. While 21/2 can not be simplified further, it is an improper fraction that can be expressed as a mixed number, 10 1/2.

Final Answer: 12/1 x 7/8 = 84/8 = 21/2 or 10 1/2

Figure 09 below illustrates how we were able to multiply a whole number by a fraction to solve this problem.

Figure 12: Whenever you end up with an improper fraction, you will likely have to convert it to a mixed number.

Now that you know how to multiply a fraction by a whole number and how to multiply a whole number by a fraction, it’s time to move onto the final section where we will go over how to multiply mixed fractions.

---

How to Multiply Mixed Fractions

How to Multiply Fractions with Mixed Numbers: Example #1

Example #1: 3/5 x 4 1/2

Multiplying fractions with mixed numbers can be done using our same three-step strategy, but with one small extra step at the very beginning.

Before you can solve this problem, you will have to convert the mixed number into an improper fraction.

For this example, the mixed number 4 1/2 can be rewritten as 9/2 (both of these are equivalent):

• 3/5 x 4 1/2 → 3/5 x 9/2

Now, we can solve 3/5 x 9/2 to find the answer to this problem as follows:

Step One: Multiply the numerators together.

Start off multiplying the numerators of both fractions:

• 3 x 9 = 27

Step Two: Multiply the denominators together.

Continue by multiplying the denominators of both fractions:

• 5 x 2 = 10

Step Three: See if the resulting fraction can be simplified or reduced.

After steps one and two, we are left with the improper fraction 27/10.

• 3/5 x 9/2 = 27/10

While 27/10 can not be simplified, it can be expressed as the mixed number 2 7/10.

Final Answer: 3/5 x 9/2 = 27/10 = 2 7/10

Note that 27/10 is technically a correct answer, but most problems requiring you to multiply mixed fractions will call for you to express your final answer as a mixed fraction.

See Figure 10 for step-by-step details of how we solved this problem.

Figure 10: How to Multiply Fractions with Mixed Numbers

Moving on, let’s work through one final practice problem where we will have to multiply a mixed number by another mixed number.

---

How to Multiply Mixed Fractions: Example #2

Example #2: 4 1/5 x 3 2/3

For this example, notice that there are two mixed numbers.

Similar to the previous example, you will have to convert both mixed numbers into equivalent improper fractions before you can use the three-step strategy to solve:

• 4 1/5 = 21/5

• 3 2/3 = 11/3

Now we can go ahead and find the answer by solving 21/5 x 11/3

Step One: Multiply the numerators together.

First, find the product of the numerators of both fractions:

• 21 x 11 = 231

Step Two: Multiply the denominators together.

Next, find the product of the denominators of both fractions:

• 5 x 3 = 15

Step Three: See if the resulting fraction can be simplified or reduced.

Finally, we are left with the improper fraction 231/15

• 21/5 x 11/3 = 231/15

While 231/15 is a pretty ugly improper fraction, it can actually be simplified since 231 and 15 share a GCF of 3. So, after dividing both numbers by 3, we are left with 77/5.

• 21/5 x 11/3 = 231/15 = 77/5

Assuming that we have to express our final answer as a mixed number, we lastly have to rewrite 77/5 as 15 2/5 and we have solved the problem!

Final Answer: 21/5 x 11/3 = 231/15 = 77/5 = 15 2/5

The graphic in Figure 11 below illustrates how we used the 3-step method to solve this problem.

Figure 14: How to Multiply Fractions with Mixed Numbers (Step-by-Step)

---

Conclusion: How to Multiply Fractions

Multiplying fractions is an important math skill that you can master with a little bit of practice.

This guide shared a simple and effective 3-step strategy that you can use to solve any problem requiring you to multiply fractions together in any of the following scenarios:

• multiplying fractions by other fractions

• multiplying fractions by whole numbers

• multiplying whole numbers by fractions

• multiplying mixed fractions

• multiplying mixed numbers

The good news is that the 3-step strategy shared in this guide can be used to solve problems for all of the above scenarios and can be summarized as follows:

• Step One: Multiply the numerators together.

• Step Two: Multiply the denominators together.

• Step Three: See if the resulting fraction can be simplified or reduced.

The more practice you get using these three steps, the better at multiplying fractions you will become!

Keep Learning

How to Divide Fractions in 3 Easy Steps (Free Guide)

---

Search Tags: how to subtract fractions, subtracting fractions, how to subtract fractions with different denominators, fraction subtraction, subtracting fractions with unlike denominators, how do you subtract fractions?

How to Divide Fractions in 3 Easy Steps

How to Divide Fractions in 3 Easy Steps

Math Skills: How do you divide fractions by fractions, how do you divide fractions with whole numbers, and how to divide mixed fractions?

Free Step-by-Step Guide: How to Divide Fractions Step-by-Step Guide

Performing operations on fractions can be challenging. Once you have mastered how to add fractions and how to subtract fractions, the next step is learning how to multiply and how to divide fractions.

The following free guide specifically focuses on teaching you how to divide fractions in the following scenarios:

• How to Divide Fractions by Other Fractions

• How to Divide Fractions with Whole Numbers

• How to Divide Mixed Fractions (or Mixed Numbers)

While dividing fractions may seem challenging at first glance, you can actually easily solve all three types of problems by using a simple 3-step method for dividing fractions, which you will learn and apply to several practice problems further down in this step-by-step guide.

Together, we will learn the Keep-Change-Flip method for how to divide fractions and use it to solve a variety of practice problems.

However, before we dive into working through any examples, let’s do a quick review of some key vocabulary terms and prerequisite skills that you will need to refresh in order to be successful with this new math skill.

Before we cover how to subtract fractions and work through a few examples, let’s do a fast recap of some key characteristics and vocabulary terms related to subtracting fractions.

Are you ready to get started?

How to Divide Fractions: Key Vocabulary

Since we will be using several math vocabulary terms in this guide, it is important that you are familiar with their meanings before you start working on the practice problems.

What is the numerator of a fraction?

The numerator of a fraction is the top number of the fraction. For example, for the fraction 2/3, the numerator is 2.

What is the denominator of a fraction?

The denominator of a fraction is the bottom number of the fraction. For example, for the fraction 2/3, the numerator is 3.

Pretty simple, right? Whenever we mention the numerator of a fraction, we are talking about the top number. On the other hand, whenever we mention the denominator of a fraction, we are talking about the bottom number. Figure 01 below illustrates the relationship.

Figure 01: In any fraction, the numerator is the top number and the denominator is the bottom number.

Next, let’s make sure that you understand the difference between fractions, whole numbers, and mixed numbers (also referred to as mixed fractions).

What is a whole number?

In math, a whole number is a number that is not a fraction. For example, 7, 23, and 100 are all whole numbers.

What is a fraction?

In math, a fraction is a number that represents a part of a whole number. For example, 1/2, 2/3, and 5/8 are all fractions.

What is a mixed number?

In math, a mixed number (or a mixed fraction) is a number that consists of both a whole number and a proper fraction. For example, 3 2/5 is a mixed number that represents the sum of 3 and 2/5.

Why is it important for you to understand the key characteristics and differences between these three types of numbers? Because, in this guide, you will learn how to work with dividing each type of number using a simple 3-step method.

Figure 02: What is a whole number? What is a fraction? What is a mixed fraction?

Now that you are familiar with all of the key vocabulary related to how to divide fractions, you are ready to work through some practice problems. Let’s start with learning how to divide fractions by other fractions.

---

How to Divide Fractions by Fractions

How to Divide Fractions by Fractions: Example #1

Example #1: 1/4 ÷ 1/4

Our first dividing fractions example is very simple, and you may already know the answer. In this case, we are taking the fraction 1/4 (one-fourth) and dividing it by 1/4 (one-fourth). So, we are dividing the same non-zero value by itself, which we should already know will be equal to 1.

Even though we already know what the answer should be, let’s go ahead and learn our 3-step method for dividing fractions to this first practice problem to see if it works as it should.

How to Divide Fractions in 3 Easy Steps

• Step One: Keep the first fraction as is.

• Step Two: Change the division sign to a multiplication sign.

• Step Three: Switch the position of the numerator and the denominator in the second fraction. Then multiply the numerators together and the denominators together and simplify if possible.

We will also be referring to this 3-step method as Keep-Change-Flip. Once you learn how to successfully follow these three simple steps, you can use them to solve any problem where you have to divide fractions!

Let’s go ahead and apply them to this first example: 1/4 ÷ 1/4

Step One: Keep the first fraction as is.

This first thing that you have to do is nothing at all. Simply leave the first fraction as is. So, 1/4 stays as 1/4.

Step Two: Change the division sign to a multiplication sign.

Next, take the division sign (÷) and change it to a multiplication sign (x) as follows:

• 1/4 ÷ → 1/4 x

Step Three: Switch the position of the numerator and the denominator in the second fraction. Then multiply the numerators together and the denominators together and simplify if possible.

For this last step, take the second fraction and “flip” the position of the numerator and the denominator. So, 1/4 becomes 4/1 as follows:

• 1/4 → 4/1

Now, we have used the Keep-Change-Flip method transform the original problem:

• 1/4 ÷ 1/4 → 1/4 x 4/1

Figure 03 below illustrates how we used the keep-change-flip method to transform the original division problem into a multiplication problem.

Figure 03: How to Divide Fractions: Transform the original division problem into a multiplication problem using keep-change-flip.

Finally, we can solve this problem by multiplying these two fractions together. To do this, simply multiply the numerators together and the denominators together as follows:

• 1/4 x 4/1 = (1x4) / (4x1) = 4/4 = 1

Final Answer: 1

When we multiply these two fractions together, we are left with (1x4) / (4x1) = 4/4 and we know that 4/4 can be reduced to 1, which we expected the answer to be when we first started this problem!

(Looking for some extra help with multiplying fractions, click here to access our free student guide).

Figure 04 below illustrates how we solved this problem after performing keep-change-flip on the original expression.

Figure 04: How to solve Example #1 after performing keep-change-flip on the original problem.

Confused? If so, that’s okay. The important thing is that you gain some experience with using keep-change-flip. Now let’s go ahead and apply it to another practice problem.

---

How to Divide Fractions by Fractions: Example #2

Example #2: 2/3 ÷ 4/5

For this second practice problem, we will again be using the 3-step method:

Step One: Keep the first fraction as is.

The first step, simply keep the first fraction 2/3 the same (i.e. you can just leave it as is).

Step Two: Change the division sign to a multiplication sign.

Next, take the division sign (÷) and change it to a multiplication sign (x) as follows:

• 2/3 ÷ → 2/3 x

Step Three: Switch the position of the numerator and the denominator in the second fraction. Then multiply the numerators together and the denominators together and simplify if possible.

Finally, flip the positions of the numerator and the denominator of the second fraction as follows:

• 4/5 → 5/4

Again, these 3-steps can be referred to as keep-change-flip, and they are illustrated below in Figure 05:

Figure 05: Transform the original problem from Example #2 using Keep-Change-Flip

As you can see, we have transformed the original problem using keep change flip and we can now solve it as follows:

• 2/3 x 5/4 = (2x5) / (3x4) = 10/12 = 5/6

Final Answer: 5/6

Remember, whenever you are multiplying two fractions together, you have to multiply the numerators together and then multiply the denominators together. In this example, we are left with (2x5)/(3x4) = 10/12, and, since 10 and 12 are both divisible by 2, we can reduce and express our final answer as 5/6.

Figure 06 below shows the complete process for solving this second example.

Figure 06: How to Divide Fractions Using the Keep-Change-Flip Method

Now, let’s go ahead and work through one more example of dividing a fraction by another fraction.

---

How to Divide Fractions by Fractions: Example #3

Example #2: 7/8 ÷ 11/12

Step One: Keep the first fraction as is.

As always, start by keeping the first fraction (7/8 in this example) as is.

Step Two: Change the division sign to a multiplication sign.

Next, change the division sign (÷) to a multiplication sign (x):

• 7/8 ÷ → 7/8 x

Step Three: Switch the position of the numerator and the denominator in the second fraction. Then multiply the numerators together and the denominators together and simplify if possible.

Now, flip the positions of the numerator and the denominator of the second fraction:

• 11/12 → 12/11

The keep-change-flip process for Example #3 is shown in Figure 07 below:

Figure 07: How to transform a fractions division problem using keep-change-flip.

Now that have transformed the original problem, we can solve it as follows:

• 7/8 x 12/11 = (7x12) / (8x11) = 84/88 = 21/22

Final Answer: 21/22

In this case, we end up with (7x12) / (8x11) = 84/88 and, since 84 and 88 are both divisible by 4, we can reduce and express our final answer as 21/22.

Figure 08 illustrates how we solved this problem.

Figure 08: How to Divide Fractions Using Keep-Change-Flip.

Next, we will learn how to divide fractions with whole numbers.

---

How to Divide Fractions with Whole Numbers

This next section will teach you how to divide a fraction by a whole number and how to divide a whole number by a fraction. We will again be using the keep-change-flip method to solve these kinds of problems. If you want a more in-depth review of how to use the keep-change-flip method, you can click here to revisit the previous section.

How to Divide a Fraction by a Whole Number: Example #1

Example #1: 3/7 ÷ 2

Ready for some good news? You can use the same keep-change-flip method from the previous section to solve these kinds of problems as well. However, there is one extra step involved. Whenever you want to divide a fraction by a whole number using keep-change-flip, you have to rewrite the whole number as a fraction by giving it a denominator of 1.

For this example, we can rewrite the whole number, 2, as a fraction as follows:

• 2 → 2/1

These both mean the same thing! Now, we can say that solving the original problem is the same as solving 3/7 ÷ 2/1. And since this new problem is just dividing a fraction by another fraction, we can use the keep-change-flip method as follows:

Step One: Keep the first fraction as is.

For the first step, simply keep the first fraction, 3/7, as is.

Step Two: Change the division sign to a multiplication sign.

For the second step, change the division sign (÷) into a multiplication sign (x) as follows:

• 3/7 ÷ → 3/7 x

Step Three: Switch the position of the numerator and the denominator in the second fraction. Then multiply the numerators together and the denominators together and simplify if possible.

Finally, flip the positions of the numerator and the denominator of the second fraction as follows:

• 2/1 → 1/2

The keep-change-flip process effectively transforms the division problem into an equivalent multiplication problem that is much easier to solve. This entire process is illustrated in Figure 09 below.

Figure 09: How to Divide a Fraction by a Whole Number: Rewrite the whole number as a fraction with a denominator of 1 and use the keep-change-flip method to solve.

Using the keep change flip method transforms the original division problem into an equivalent multiplication problem. To multiply two fractions together, simply multiply the numerators together and then multiply the denominators together as follows:

• 3/7 x 1/2 = (3x1) / (7x2) = 3/14

Notice that the result, 3/14, can not be reduced. Therefore:

Final Answer: 3/14

The graphic in Figure 10 below details the entire process for solving this problem.

Figure 10: 3/7 x 1/2 = 3/14

In the next example, we will use the exact same process to divide a whole number by a fraction.

---

How to Divide a Whole Number by a Fraction: Example #2

Example #1: 9 ÷ 3/4

If we want to use the keep-change-flip method to divide a whole number by a fraction, then we will have to rewrite the whole number (9 in this case) as a fraction as follows:

• 9 → 9/1

Now we can rewrite the original problem 9 ÷ 3/4 as 9/1 ÷ 3/4 (remember that these expressions are equivalent—i.e. they both mean the same thing). Now that we have two fractions being divided by each other, we can use keep-change-flip to solve.

Step One: Keep the first fraction as is.

Just like before, start by keeping the first fraction, 9/1, as is.

Step Two: Change the division sign to a multiplication sign.

Next, change the division sign (÷) into a multiplication sign (x) as follows:

• 9/1 ÷ → 9/1 x

Step Three: Switch the position of the numerator and the denominator in the second fraction. Then multiply the numerators together and the denominators together and simplify if possible.

Finally, flip the numerator and the denominator of the second fraction as follows:

• 3/4 → 4/3

The entire process of using keep-change-flip to transform this problem is illustrated in Figure 11 below.

Figure 11: How to Divide a Whole Number by a Fraction: Start by rewriting the whole number as a fraction and then use keep-change-flip to solve.

Now we can solve the problem by multiplying these two fractions together as follows:

• 9/1 x 4/3 = (9x4) / (1x3) = 36/3 = 12

Notice that the result, 36/3, can be reduced. Since both the numerator and denominator are divisible by 3, we can conclude that the final answer is 12/1 or just 12.

Final Answer: 12

The graphic in Figure 12 further illustrates how we solved this problem.

Figure 12: How to divide a whole number by a fraction using keep-change-flip

Now that you know how to divide a fraction by a whole number and how to divide a whole number by a fraction, let’s move onto the final section where you will learn how to divide mixed fractions.

---

How to Divide Mixed Fractions

The final section of the How to Divide Fractions guide will focus on how to divide mixed fractions and how to divide fractions with mixed numbers.

Again, we can solve all of these types of problems by using the keep-change-flip method (click here to revisit the previous section).

Let’s get started with our first example!

How to Divide Fractions with Mixed Numbers: Example #1

Example #1: 5/9 ÷ 3 1/3

Whenever you have to divide fractions with mixed numbers, you can use the keep-change-flip method to find the answer. However, before you can use keep-change-flip, you will have to convert the mixed number into an improper fraction first.

In this example, we have to convert the fraction 3 1/3 into an improper fraction (a fraction whose numerator is greater than its denominator).

We can rewrite 3 1/3 as 10/3 because:

• 3 1/3 = 3/3 + 3/3 + 3/3 + 1/3 = 10/3

If you need more help with converting mixed numbers into improper fractions, we highly recommend checking out this free guide before moving forward.

Now, we can rewrite the original problem as follows:

• 5/9 ÷ 3 1/3 → 5/9 ÷ 10/3

Both of these expressions are equivalent to each other. The key difference here is that the new expression allows us to use the keep-change-flip method to solve it as follows:

Step One: Keep the first fraction as is.

As always, simply keep the first fraction, 5/9, as is.

Step Two: Change the division sign to a multiplication sign.

Next, change the division sign (÷) to a multiplication sign (x) as follows:

• 5/9 ÷ → 5/9 x

Step Three: Switch the position of the numerator and the denominator in the second fraction. Then multiply the numerators together and the denominators together and simplify if possible.

Lastly, flip the positions of the numerator and the denominator of the second fraction as follows:

• 10/3 → 3/10

Why are we doing this? Remember that the keep-change-flip method allows us to transform the original division problem into an equivalent multiplication problem that will be much easier to solve. This process is highlighted in Figure 13 below.

Figure 13: How to Divide Fractions with Mixed Numbers: Rewrite the mixed number as an improper fraction and then use keep-change-flip to solve.

From here, we can solve by multiplying these two fractions together as follows:

• 5/9 x 3/10 = (5x3) / (9x10) = 15/90 = 1/6

Final Answer: 1/6

Figure 14 below illustrates how we were able to divide fractions with mixed numbers and solve this problem.

Figure 14: How to Divide Fractions with Mixed Numbers Explained

Now, let’s work through the final example of this guide where you will learn how to divide mixed fractions.

---

How to Divide Mixed Fractions: Example #2

Example #1: 6 1/2 ÷ 2 1/4

Just like the previous example, we can use the keep-change-flip method to solve this problem, but first we have to convert the mixed fractions in this problem into improper fractions.

In this case:

• 6 1/2 = 2/2 + 2/2 + 2/2 + 2/2 + 2/2 + 2/2 + 1/2 = 13/2

• 2 1/4 = 4/4 + 4/4 + 1/4 = 9/4

So, we can rewrite the original problem as:

• 6 1/2 ÷ 2 1/4 → 13/2 ÷ 9/4

Figure 14: How to Divide Mixed Fractions: Start by rewriting any mixed fractions as improper fractions.

Again, if you need to review how to convert a mixed numbers into an improper fraction, we highly recommend checking out this free step-by-step guide.

Now, we have a new, yet equivalent, expression to be solved using keep-change-flip:

• 13/2 ÷ 9/4

Step One: Keep the first fraction as is.

First, keep the first fraction, 13/2, as is.

Step Two: Change the division sign to a multiplication sign.

Second, change the division sign (÷) to a multiplication sign (x) as follows:

• 13/2 ÷ → 13/2 x

Step Three: Switch the position of the numerator and the denominator in the second fraction. Then multiply the numerators together and the denominators together and simplify if possible.

And finally, flip the numerator and the denominator positions of the second fraction:

• 9/4 → 4/9

Figure 15: How to Divide Mixed Fractions Explained

The last thing we have to do to solve this problem is multiply these two improper fractions together as follows:

• 13/2 x 4/9 = (13x4) / (2x9) = 26/9 or 2 8/9

For this example, we are left we the improper fraction 26/9, which can not be reduced any further. You can also convert 26/9 into a mixed number, which would be 2 8/9. Both of these answers are equivalent.

Final Answer: 26/9 or 2 8/9

Figure 16 below shows how we found this final answer.

Figure 16: You will likely have to convert your final answer into a mixed number.

---

Conclusion: How to Divide Fractions

Dividing fractions can be a conceptually challenging math task, but learning how to use the keep-change-flip method can make the process much easier.

Whether you are dividing fractions with other fractions, fractions with whole numbers, whole numbers with fractions, or fractions with mixed numbers, the keep-change-flip method provides a simple three-step process for solving these types of problems.

The keep-change-flip method for dividing fractions can be summarized as follows:

• Step One: Keep the first fraction as is.

• Step Two: Change the division sign to a multiplication sign.

• Step Three: Flip the position of the numerator and the denominator in the second fraction.

After you perform keep-change-flip, you can solve the problem by multiplying and reducing the result whenever possible.

How to Add Fractions in 3 Easy Steps

---

Search Tags: how to subtract fractions, subtracting fractions, how to subtract fractions with different denominators, fraction subtraction, subtracting fractions with unlike denominators, how do you subtract fractions?

Combining Like Terms Explained—Examples, Worksheet Included

Combining Like Terms Explained

How to Combine Like Terms Quickly and Easily (Worksheet Included)

Free Step-by-Step Guide: Combining Like Terms

Combining like terms is an important math skill that involves simplifying an expression by combining terms that have the same variables and/or exponents. You combine like terms when you want to make an expression simpler and easier to read and solve.

This free Step-by-Step Guide to Combining Like Terms will walk you through how to quickly and easily combine like terms and it includes examples and a free practice worksheet. This guide will cover the following topics:

• What are Terms in Math?

• What Does Combining Like Terms Mean?

• How to Combine Like Terms

• Combining Like Terms Examples

• Free Combining Like Terms Worksheet

Before we dive into how to combine like terms, lets do a quick review of some important math vocabulary related to combining like terms (this quick recap will help you to better understand the examples in this guide).

What are Terms in Math?

In math, a term is one single number or algebraic expression that is part of a larger expression or equation.

For example, the mathematical expression 6x + 8y - 4z + 5 has four terms:

• 6x

• 8y

• -4z

• 5

What are Terms in Math?

Each of the first three terms consists of a coefficient (a number in front of a variable) and variable letter (x, y, or z in this example). The fourth term is called a constant because it is a single number without a variable.

It is also important to note that a term can have multiple variables as well. For example, 6xy or 10xyz could be terms (more on this later on).

What are terms in math? The important thing to understand right now is that each individual part of an expression (separated by mathematical operation signs like + or -) is a term and, when terms are like, they can be combined to create a simpler result that is easier to work with.

---

Combining Like Terms Definition

Now that you understand what are terms in math, you are ready to learn how to combine like terms.

Combining Like Terms Definition: Combining like terms is the act of simplifying an algebraic expression by either adding or subtracting terms that have the same variables and/or exponents.

Before we look at a simple numerical example, lets take a look at the fruit expression in Figure 01 below.

Figure 01: The fruit expression has three terms: pomegranates, avocados, and lemons.

The fruit expression has three terms: pomegranates, avocados, and lemons.

You can read this expression verbally as: 14 pomegranates plus 8 lemons plus 5 avocados minus 6 pomegranates plus 4 avocados minus 2 lemons.

By looking at this expression, it should be clear that you can make it simpler by combining like fruits. We can easily do this by color coding the terms as follows:

• Highlight pomegranates in pink

• Highlight lemons in yellow

• Highlight avocados in green

Now, you can make the following combinations of like fruits:

• Pomegranates: 14 + - 6 = 8 pomegranates*

• Lemons: 8 + -2 = 6 lemons

• Avocados: 5 + 4 = 9 avocados

*Note that you simplify an expression like 8+-2 as 8-2.

So, after combining like terms, the new expression would be: 8 pomegranates plus 6 lemons plus 9 avocados

Figure 02 below illustrates how we just combined like terms (fruit) to simplify a complicated expression by using color-coding.

Figure 02: Combine Like Terms Using Color Coding

How to Combine Like Terms

The fruit expression is a good first introduction to combining like terms. Obviously, in math, you won’t be dealing with fruit, but variables like x, y, and z instead.

For example, consider the expression 3x + 2y + 5x -3z + 7y + z

To combine like terms, we can color coordinate the x-terms, y-terms, and z-terms and then combine them together group by group as follows:

• x-terms: 3x + 5x = 8x

• y-terms: 2y + 7y = 9y

• z-terms: -3z + z = -2z

After combining like terms, the new expression would be: 8x +9y - 2z.

This example is illustrated in Figure 03 below.

Figure 03: How to combine like terms.

Now, lets work through 3 more step-by-step examples of combining like terms.

---

Combining Like Terms Examples

Example #1: Combine Like Terms 5x + 9 + 2x

For this first example, you have to combine like terms 5x + 9 + 2x

This expression has 2 terms. To combine like terms, we can color coordinate the x-terms and constant terms, and then combine them together group by group as follows:

• x-terms: 5x + 2x = 7x

• constant terms: 9

After combining like terms, the new expression would be: 7x + 9

This example is illustrated in Figure 04 below.

Figure 04: Combine Like terms 5x + 9 + 2x

---

Example #2: Combine Like Terms: 7q + 5r -4 +3s -3q +5r - 3

This expression has 4 terms. To combine like terms, we can color coordinate the q-terms, r-terms, s-terms, and constant terms, and then combine them together group by group as follows:

• q-terms: 7q + (-3q) = 4q

• r-terms: 5r + 5r=10r

• s-terms: 3s

• constant terms: -4 + (-3) = -7

Notice that there is only one s term, so you can not combine it with another like term.

After combining like terms, the new expression would be: 4q+10r+3s-7

This example is illustrated in Figure 05 below.

Figure 05: How to Combine Like Terms

---

Example #3: Combine Like Terms: -5xy^2 +2x^2 + 4xy^2 - 2x^2 - y

This expression has 3 terms. To combine like terms, we can color coordinate the xy^2-terms, x^2 terms, and y-terms, and then combine them together group by group as follows:

• xy^2-terms: -5xy^2 + 4xy^2 = -1xy^2

• x^2-terms: 2x^2 + (-2x^2) = 0 (they cancel each other out)

• y-terms: -y

After combining like terms, the new expression would be: -xy^2 - y

This example is illustrated in Figure 06 below.

Figure 06: Combining Like Terms

---

Free Combining Like Terms Worksheet

Combining Like Terms Worksheet Preview

Are you looking for more practice with combining like terms?

Click the link below to download our free Combining Like Terms worksheet as a pdf file (full answer key included). We highly recommend using colored pencils or highlighters to color code the terms in each example.

→ Download your free Combining Like Terms Worksheet PDF File (with Answer Key)

Keep Learning:

---

How to Add Fractions in 3 Easy Steps

---

Search Tags: combining like terms, combine like terms, combining like terms worksheet, how to combine like terms, combine like terms worksheet, combine line terms 5x + 9 + 2x, combine like terms definition, combining like terms examples, combining like terms worksheet pdf

How to Subtract Fractions in 3 Easy Steps

How to Subtract Fractions in 3 Easy Steps

Math Skills: How do you subtract fractions with the same denominator and how to subtract fractions with different denominators

Free Step-by-Step Guide: How do you subtract fractions?

Having the knowledge of how to subtract fractions is a crucial and fundamental math skill that every student must learn, as it serves as a foundational element for comprehending more advanced math ideas that you may come across in the future.

(Looking to learn how to add fractions? Click here to access our free guide)

Fortunately, subtracting fractions, regardless of whether the denominators are the same (like) or different (unlike) can be done using a straightforward and uncomplicated three-step process.

This free Step-by-Step Guide on How to Subtract Fractions will walk you through the process of subtracting fractions with like and unlike denominators, including detailed examples for each scenario.

This step-by-step guide will focus on teaching you the following math skills:

• How to subtract fractions with the same denominator?

• Subtracting Fractions with Unlike Denominators: How to subtract fractions with different denominators?

Before we cover how to subtract fractions and work through a few examples, let’s do a fast recap of some key characteristics and vocabulary terms related to subtracting fractions.

Let’s get started!

Subtracting Fractions: Definitions and Vocabulary

Before you can learn how to subtract fractions, it’s important for you to know two key vocabulary terms (and the difference between them):

Definition: The top number of a fraction is called a numerator. For example, the fraction 5/6 has a numerator of 5.

Definition: The bottom number of a fraction is called a denominator. For example, the fraction 5/6 has a denominator of 6.

Again, the numerator is the top number of a fraction, and the denominator is the bottom number. These terms are further illustrated in Figure 01 below. While these two math vocabulary terms are simple, it is crucial that you are able to understand and correctly identify the numerator and denominator of fractions in order to master the skill of subtracting fractions.

Figure 01: Fraction Subtraction Key Terms: The numerator of a fraction is the top number, and the denominator is the bottom number.

Moving on, you are ready to take the next step towards mastering fraction subtraction. Next, you will need to be able to determine when a fraction subtraction problem falls into one of the following two categories:

• Subtracting Fractions with Like Denominator (the denominators equal the same number)

• Subtracting Fractions with Unlike Denominator (the denominators are different)

Fractions that have like denominators have bottom numbers that equal the same value.

• Example: 3/5 - 1/5 → This would be a case of subtracting fractions with like denominators since both fractions have a denominator of 5.

Alternatively, fractions that have unlike denominators have bottom numbers that do not equal the same value.

• Example: 1/2 - 3/7 →This would be a case of subtracting fractions with unlike denominators since both fractions have different bottom numbers (one has a denominator of 2 and the other has a denominator of 7).

Both examples (one with like denominators and one with unlike denominators) are illustrated in Figure 02 below.

Figure 02: How do you subtract fractions? The first step is being able to identify whether the fractions in question have like denominators or unlike denominators.

This concept may seem uncomplicated, but it is essential to refresh your understanding since you must be able to recognize if a problem involving subtracting fractions has like or unlike denominators in order to find a correct solution.

Now that you have the important foundation skills, you are ready to work through a few fraction subtraction problems.

---

How to Subtract Fractions with Like Denominators

How to Subtract Fractions with Like Denominators: Example #1

Example #1: 3/5 - 2/5

The first fraction subtraction example is pretty easy and straightforward, which is why it is a good place to start using our simple 3-step process for subtracting fractions. If you can learn to apply these three steps to easy examples, then you will be able to use the same process to solve more complex problems in the future (since the process works for any fraction subtraction problem).

How Do You Subtract Fractions in 3 Easy Steps?

• Step One: Identify whether the denominators are the same (like) or different (unlike).

• Step Two: If the example involves like denominators, move onto Step Three. If they are unlike denominators, find a common denominator.

• Step Three: Subtract the numerators and find the difference.

Now that you know the steps, let’s apply them to solving this first example:

• 3/5 - 1/5 = ?

Step One: Identify whether the denominators are the same (like) or different (unlike).

The fractions in this example have like denominators. They are both 5.

Step Two: If the example involves like denominators, move onto Step Three. If they are unlike denominators, find a common denominator.

Since the denominators are the same, you can skip ahead to the next step.

Step Three: Subtract the numerators and find the difference.

To complete this fraction subtraction problem, simply subtract the numerators and express the result as follows:

• 3/5 - 1/5 = (3-1)/5 = 2/5

Since 1/5 can not be reduced, you can conclude…

Final Answer: 2/5

Figure 03 below illustrates how you came to this conclusion.

Figure 03: How to Subtract Fractions with Like Denominators: Subtract the numerators and keep the denominator.

The first example shows you that subtracting fractions with like denominators is pretty easy.

To subtract fractions with the same denominator, subtract the numerators and keep the same denominator.

Now, let’s move onto one more example of subtracting fractions with like denominators before moving onto examples of how to subtract fractions with different denominators.

---

How to Subtract Fractions with Like Denominators: Example #2

Example #2: 8/9 - 5/9

For the next example, you will be applying the same 3-step method that you used in Example #1 as follows:

Step One: Identify whether the denominators are the same (like) or different (unlike).

In this example, the fractions have like denominators. They are both 9.

Step Two: If the example involves like denominators, move onto Step Three. If they are unlike denominators, find a common denominator.

Again, since the denominators are the same, you can skip ahead to Step Three.

Step Three: Subtract the numerators and find the difference.

To complete this fraction subtraction problem, subtract the numerators as follows:

• 8/9 - 5/9 = (8-5)/9 = 3/9

In this case, 3/9 is the correct answer, but this fraction can be simplified. Since both 3 and 9 are divisible by 3, 3/9 can be simplified as 1/3.

Final Answer: 1/3

Figure 04 below illustrates how you just solved Example #2.

Figure 04: Subtracting Fractions: 3/9 can be simplified to 1/3

Now, let’s move onto learning how to subtract fractions with different denominators.

---

How to Subtract Fractions with Different Denominators

How to Subtract Fractions with Different Denominators: Example #1

Example #1: 1/2 - 3/7

Step One: Identify whether the denominators are the same (like) or different (unlike).

In this example, the fractions have unlike denominators (they are different). The first fraction’s denominator is 2 and the other’s is 7.

Step Two: If the example involves like denominators, move onto Step Three. If they are unlike denominators, find a common denominator.

Since the fractions have unlike denominators, you can not skip ahead.

Before you can move onto Step Three, you have to find a number that both denominators can divide into evenly. This is called a common denominator.

A very easy and effective way to find a common denominator between two fractions is by multiplying the denominators together (i.e. multiplying the denominator of the first fraction by the second fraction and multiplying the denominator of the second fraction by the first fraction.

• 1/2 - 3/7 (1x7)/(2x7) - (3x2)/(7x2) = 7/14 - 6/14

This fraction subtraction process is illustrated in Figure 05 below.

Figure 05: How to Subtract Fractions with Different Denominators: Find a common denominator by multiplying the denominators together.

(Looking for some extra help with multiplying fractions, click here to access our free student guide).

Now that Step Two is complete, you can see that the original question has been transformed and you are now working with equivalent fractions that have common denominators, which means that the heavy lifting has been done and you can now solve the problem by subtracting the numerators and keep the same denominator:

• 7/14 - 6/14 = (7-6)/14 = 1/14

Since 1/14 can not be reduced further, you have solved the problem…

Final Answer: 1/14

Figure 06: Once you have common denominators, simply subtract the numerators keep the same denominator.

Are you ready for one more practice problem for how to subtract fractions with unlike denominators?

---

How to Subtract Fractions with Different Denominators: Example #2

Example #1: 2/3 - 8/15

For this final example of subtracting fractions, you will again be using the 3-step method as follows:

Step One: Identify whether the denominators are the same (like) or different (unlike).

The fractions have unlike denominators (one is 3 and the other is 5).

Step Two: If the example involves like denominators, move onto Step Three. If they are unlike denominators, find a common denominator.

Just like last example, you will have to find a common denominator since the fractions have unlike denominators. You can find a common denominator by multiplying the denominators together as follows:

• (2x15)/(3x15) - (8x3)/(15x3) = 30/45 - 24/45

This process is shown in Figure 07 below.

Figure 07: How to Subtract Fractions with Different Denominators

Now that the fractions share a common denominator, you can solve the fraction subtraction problem as follows:

• 30/45 - 24/45 = (30-24)/45 = 6/45

Since both 6 and 45 are divisible by 3, you can simplify this fraction as…

Final Answer: 2/15

Figure 08: Subtracting Fractions with Unlike Denominators

Conclusion: How to Subtract Fractions

Subtracting fractions with like denominators involves simply finding the difference of the numerators (top values) and not changing the denominator (bottom value).

Subtracting fractions with unlike denominators requires you to find a common denominator, which is a value that both denominators divide evenly into.

How do you subtract fractions? You can solve any fraction subtraction problem by applying the following three step method:

• Step One: Identify whether the denominators are the same (like) or different (unlike).

• Step Two: If the example involves like denominators, move onto Step Three. If they are unlike denominators, find a common denominator.

• Step Three: Subtract the numerators and find the difference.

Keep Learning:

How to Add Fractions in 3 Easy Steps

---

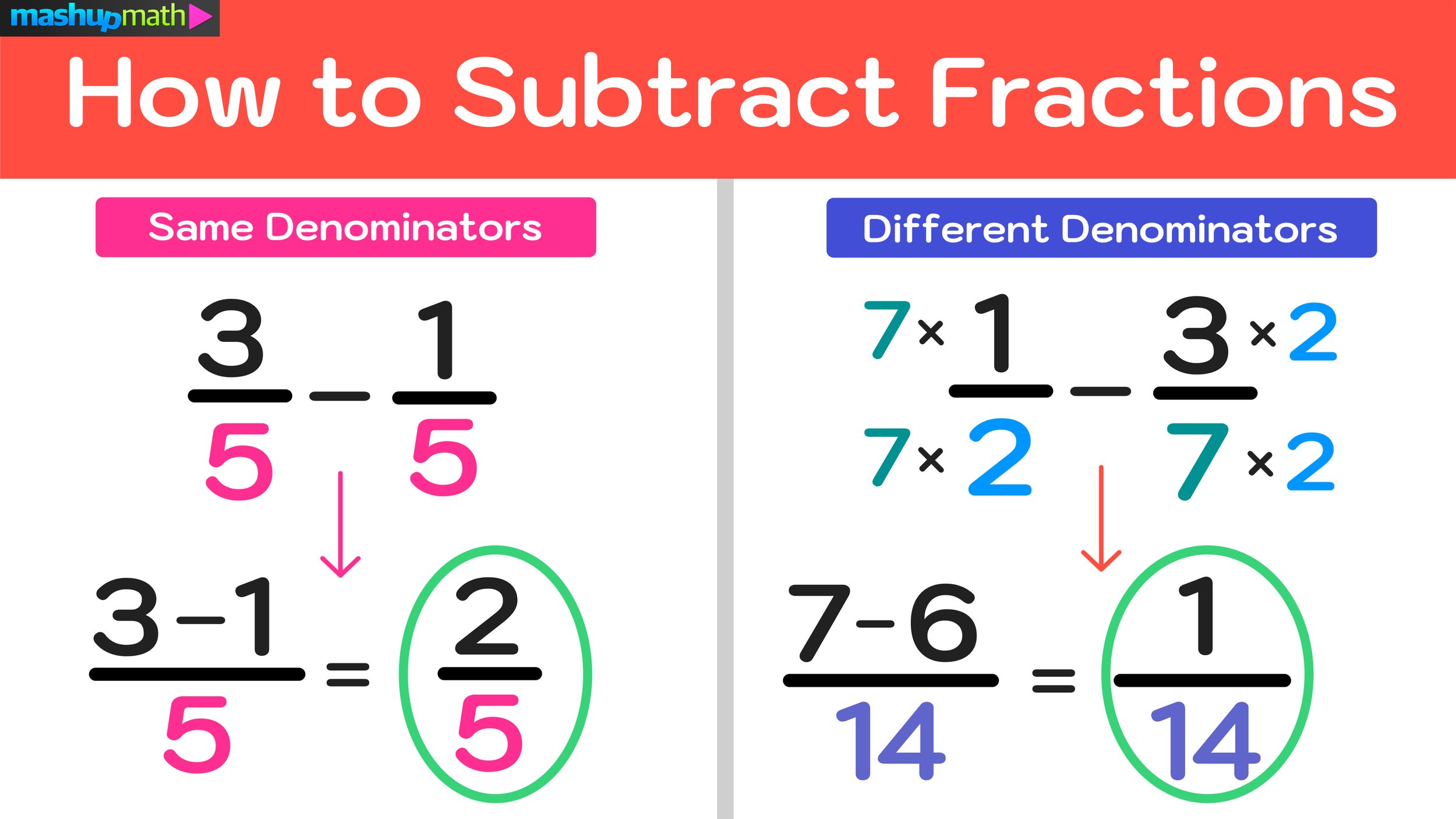
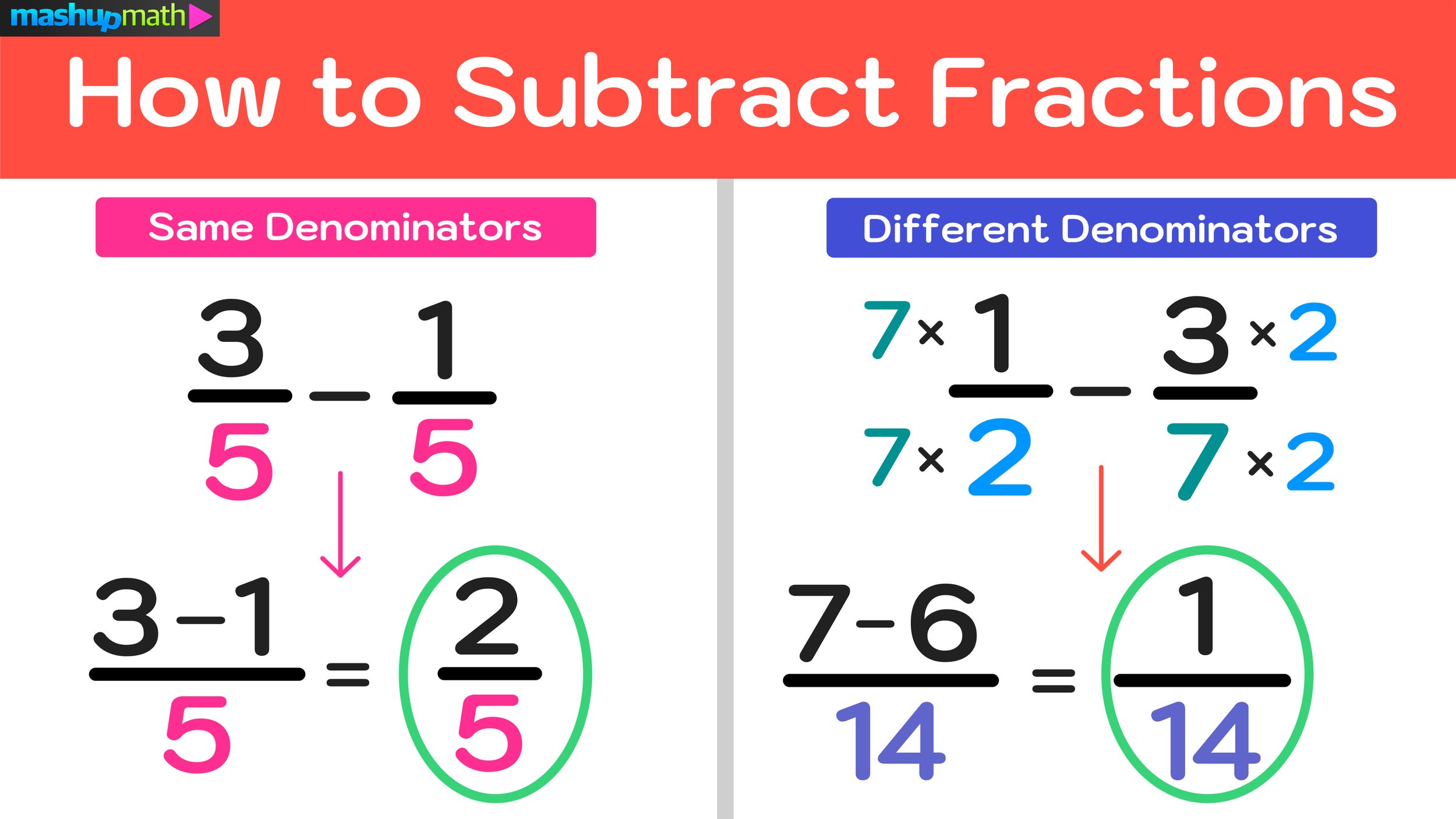
Search Tags: how to subtract fractions, subtracting fractions, how to subtract fractions with different denominators, fraction subtraction, subtracting fractions with unlike denominators, how do you subtract fractions?