Factors of 147

What are the factors of 147? What is the factorization of 147?

The factors of 147 are all of the whole numbers (both positive and negative) that can be evenly divided into the number 147.

Below is a quick reference to the factors of 147, and you can continue reading for a more in-depth explanation of the factors of 147 as well as the prime factorization of 147.

▶ Factors of 147: 1, 3, 7, 21, 49, 147

▶ Prime Factorization of 147: 3 × 7 × 7

What are the factors of 147?

In math, a factor is a number that divides into another number evenly, meaning that there is no remainder.

For example,

4 is a factor of 8 because 8 ÷ 4 = 2 → no remainder
5 is not a factor of 8 because 8 ÷ 5 = 1.6 → there is a remainder

When you want to find the factors of a number (like the factors of 147), you will simply use division to find all of the numbers that divide evenly into 147 without any remainder.

This process of searching for the factors of a number is shown in Figure 01 below.

**Figure 01: Factors of 147:** To find the factors of a number, divide that number by whole numbers to find the ones that divide into evenly (without any remainder). If the result does have a remainder, then the value is not a factor.

For example,

3 is a factor of 147 because 147 ÷ 3 = 49 → no remainder

6 is not a factor of 147 because 147 ÷ 6 = 24.5 → there is a remainder

Finding All of the Factors of 147

To find all of the factors of 147, you will have to use division to find all of the whole numbers that divide evenly into 147 without leaving a remainder.

The best strategy for finding all of the factors of 147 is to begin dividing by the number 1 and then continue with 2, 3, 4, 5, etc. You can typically stop around half of whatever number you are working with, so, in the case of 147, you could go all the way up to 75.

Note that 1 will always be a factor of any given number (since 1 divides evenly into every number).

Also, note that the number itself (147 in this case) will always be a factor, since every number divides evenly into itself.

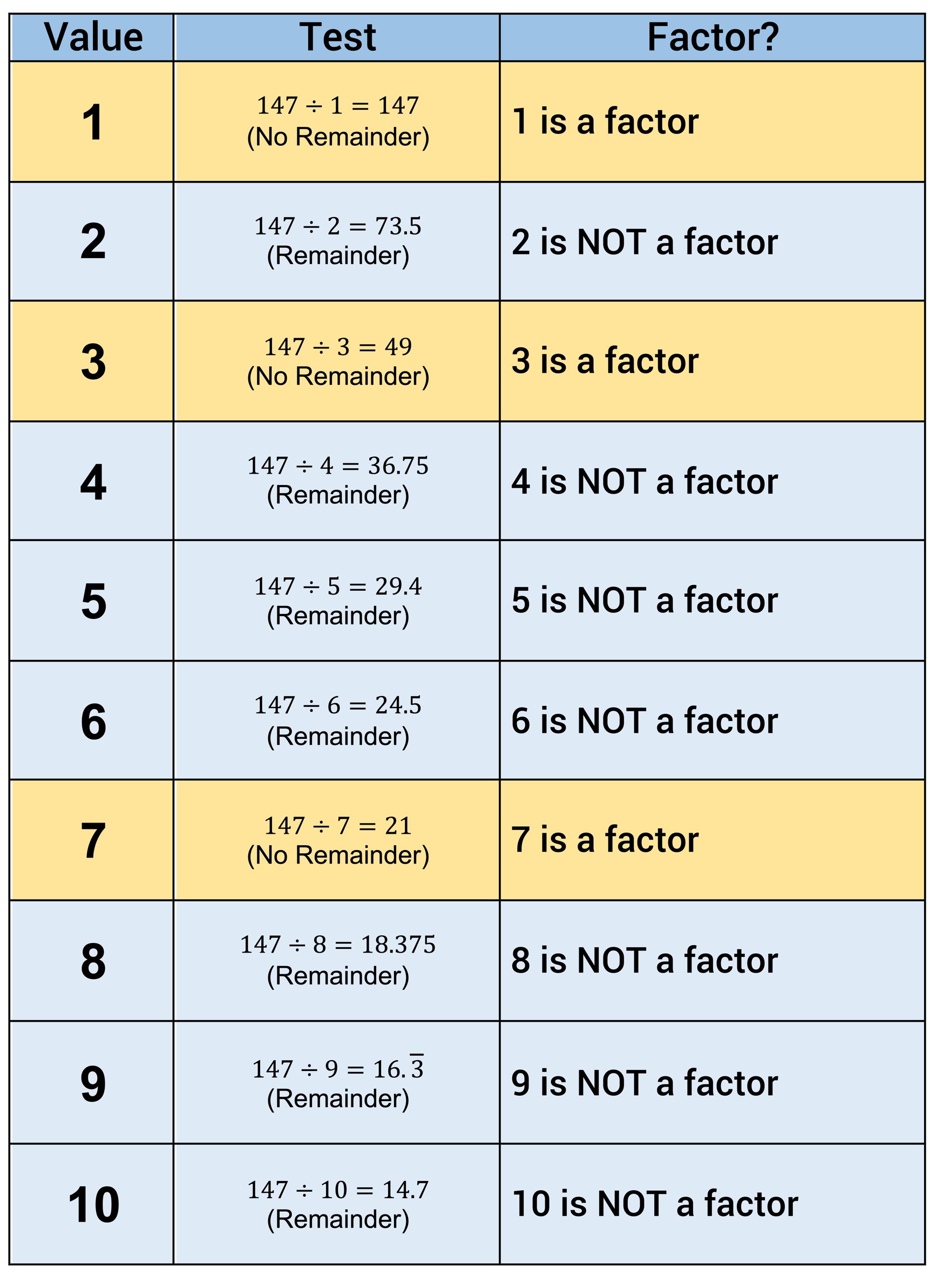
The table in Figure 02 below shows what this process would look like for checking if the numbers 1-10 are factors of 147.

Notice that the only whole numbers from 1-10 that divide evenly into 147 without a remainder are 1, 3, and 7, so they are all factors of 147.

By continuing this process, you will find three additional factors of 147:

21 is a factor of 147 because 147 ÷ 21 = 7 → no remainder
49 is a factor of 147 because 147 ÷ 49 = 3 → no remainder
147 is a factor of 147 because 147 ÷ 147 = 1 → no remainder

So, we can conclude that the factors of 147 are 1, 3, 7, 21, 49, and 147

**Figure 03:** The factors of 147 are 1,3,7,21,49,147

Prime Factorization of 147

In math, prime factorization is when you find the prime numbers that multiply together to form the original number. The process involves expressing the original number as the product of its prime factors.

Note that prime factors refer to numbers that are factors of a number AND also prime.

For your reference, the prime numbers up to 50 are shown in Figure 04 below.

**Figure 04: Factorization of 147:** The prime numbers up to 50.

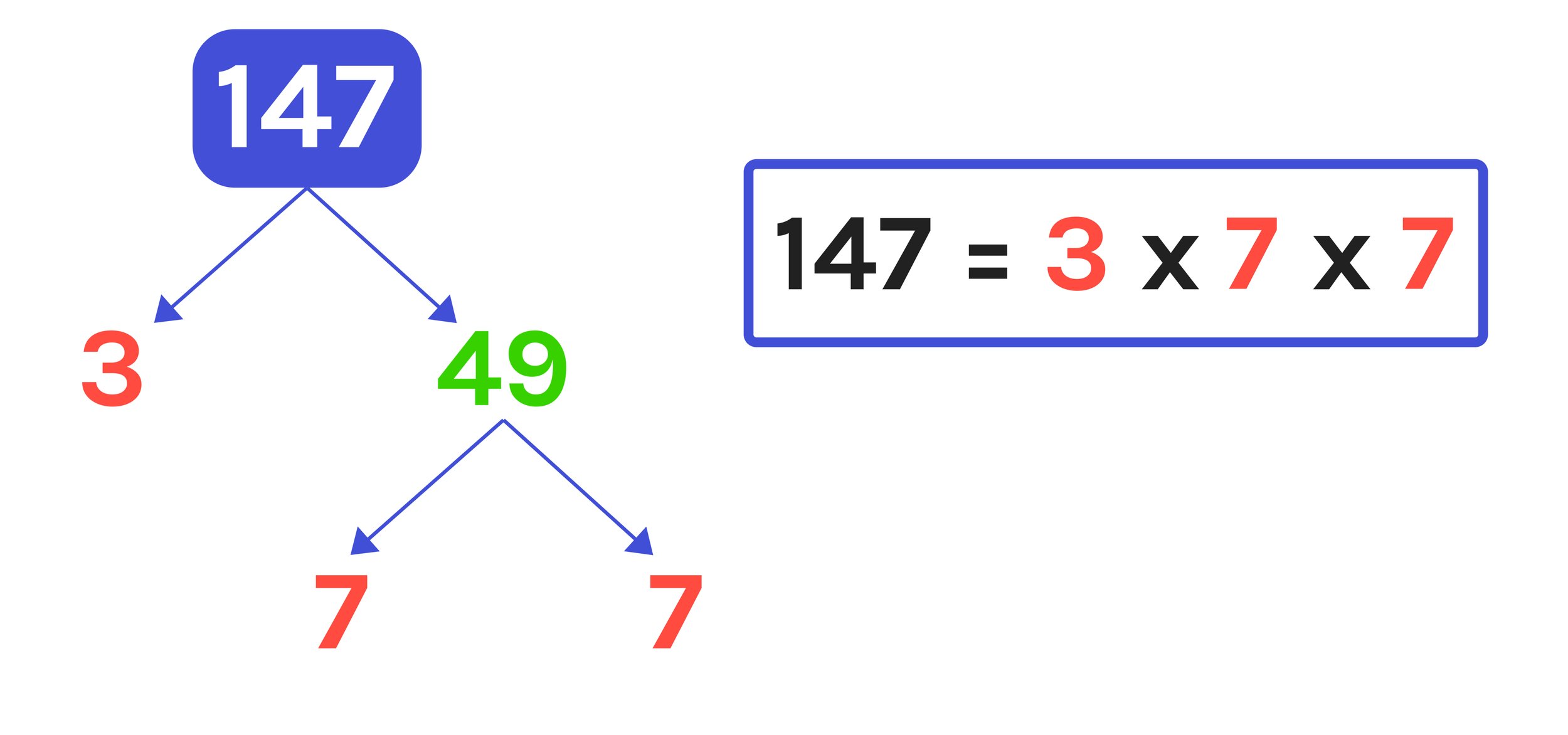
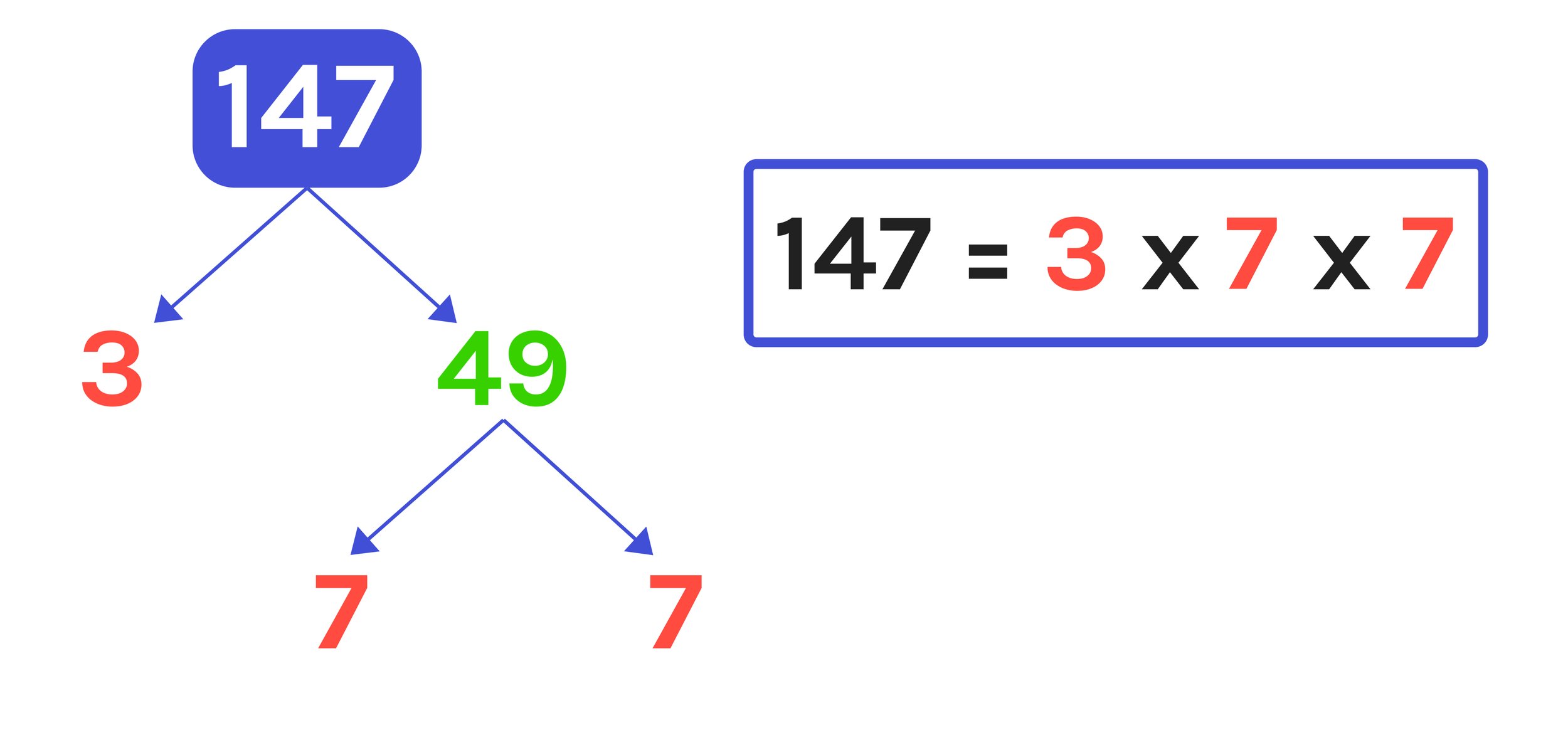
The easiest way of finding the prime factorization of 147 is to construct a factor tree as illustrated in Figure 05 below:

**Figure 05: Factorization of 147:** The prime factorization of 147 is 3 x 7 x 7

Therefore, we can conclude that the prime factorization of 147 is:

147 = 3 × 7 × 7

And now we have concluded determining both the factors of 147 and the prime factorization of 147. Being able to find the factors of a number is an incredibly useful math skill that has applications in elementary mathematics, algebra, and far beyond.