Factors of 63

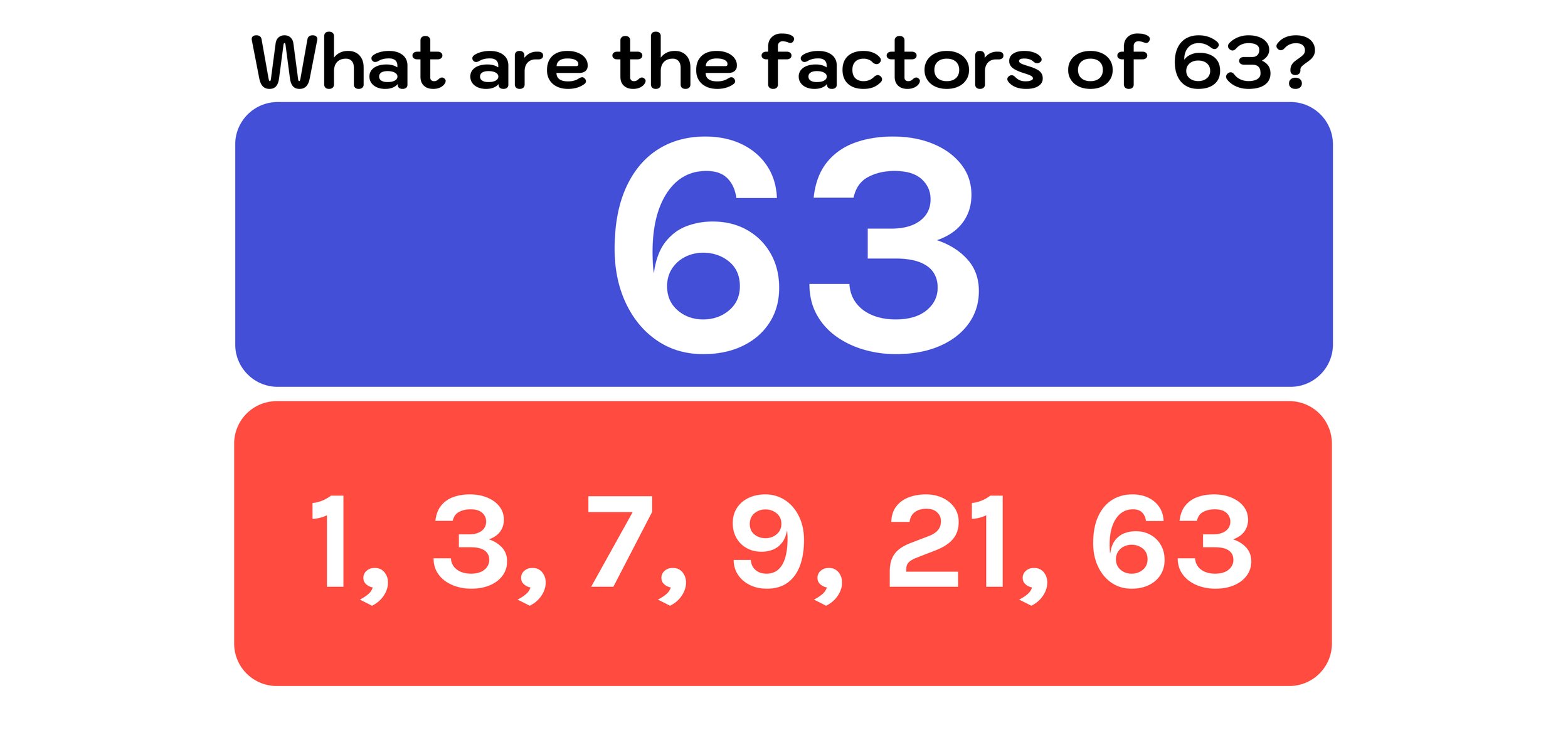
What are the factors of 63? What is the factorization of 63?

What are the factors of 63?

In math, a number is considered a factor of another number if it can be divided into it evenly without any remainder (i.e. the result is a whole number and not a fraction/decimal).

For example, if you were looking to determine whether or not 5 and 6 are factors of 20:

• 5 is a factor of 20 because 20 ÷ 5 = 4 → the result is a whole number with no remainder

• 6 is not a factor of 20 because 20 ÷ 6 = 3.3 → the result is not a whole number (it’s a decimal)

Whenever you have to find the factors of a number, you just have to use division to figure out all of the numbers that can be divided evenly into 63 that result in a whole number with no remainder.

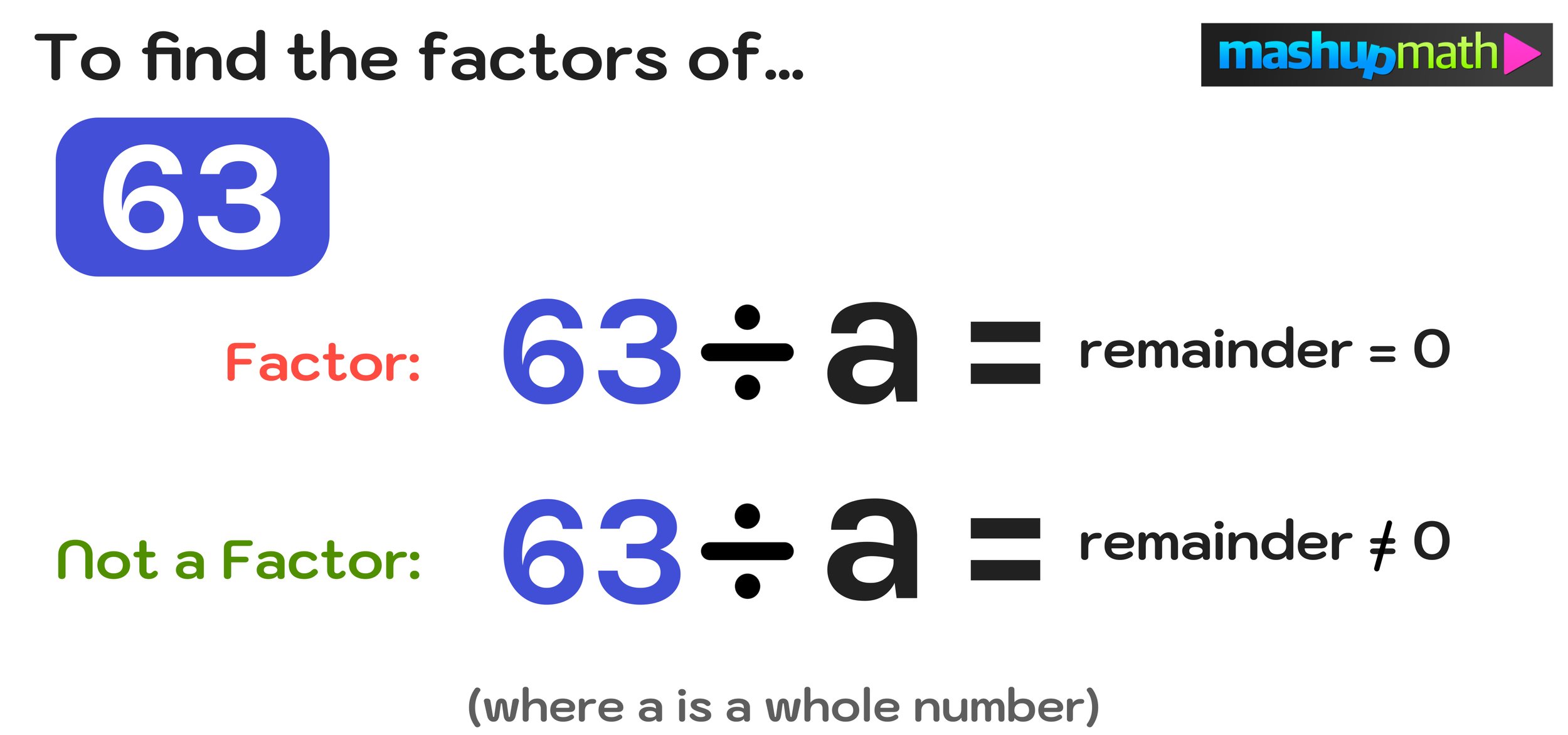
How can you figure out whether or not a number is on the list of factors of 63? Simply divide and see if the result has a remainder of not. If the remainder is zero, the number is a factor. If the remainder is not zero, the number is not a factor.

This process is further demonstrated below in Figure 01.

For example,

• 3 is a factor of 63 because 63 ÷ 3 = 21 → the result is a whole number with no remainder

• 10 is not a factor of 63 because 63 ÷ 10 = 6.3 → the result is not a whole number (it’s a decimal)

How to Find All of the Factors of 63

If you want to find all the factors of 63, you need to use division to find all of the whole numbers that can be divided into 63 without leaving a remainder.

Here's a simple way to find the factors of 63: start by dividing 63 by 1, then 2, 3, 4, 5, 6, 7, and so on. You can usually stop around the number that is equal to half of 63, which is about 32.

Also, note that 1 is always a factor of any number because it divides into every number evenly with zero remainder.

And, the number itself is always a factor because every number divides evenly into itself, i.e. 63/63=1, which is a whole number with zero remainder.

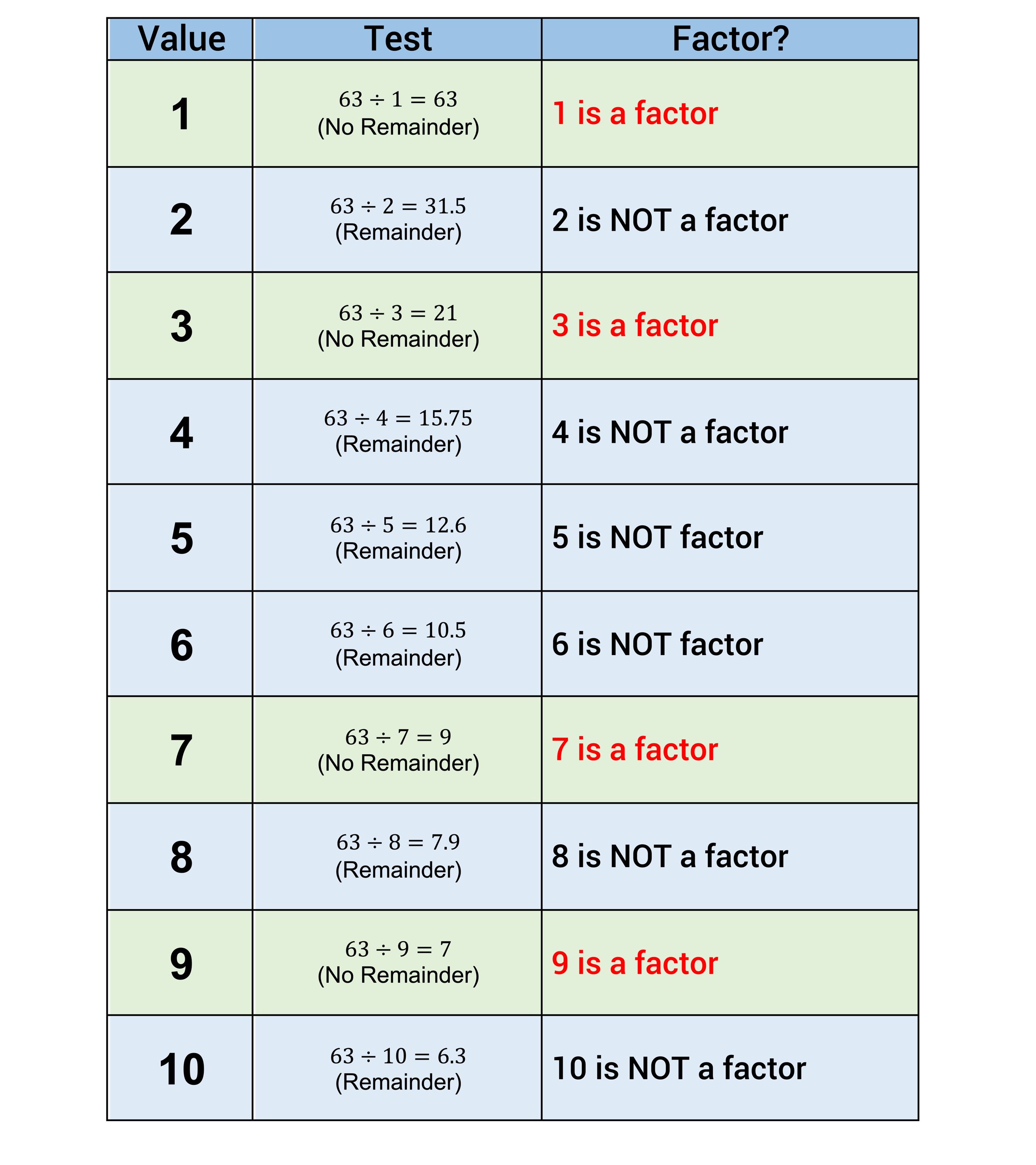
Figure 02 shows a table that details this process for determining whether or not the numbers from 1 through 10 are factors of 63.

By looking at the table, you can see that the only whole numbers between one and ten that divide evenly into 63 with a zero remainder are 1, 3, 7, and 9 so they are all factors of 63.

Continuing along with this process, you should find two more factors of 63:

• 21 is a factor of 63 because 63 ÷ 21 = 2 → zero remainder

• 63 is a factor of 63 because 63 ÷ 63 = 1 → zero remainder

Now we can say that all factors of 63 are 1, 3, 7, 9, 21, and 63

Prime Factorization of 63

In simple terms, prime factorization refers to finding the prime numbers that you can multiply together to get the original number that you started with.

To find the prime factorization of 63, you have to express 63 as the product of its prime factors. This process is similar to finding the factors of 63, but the prime factors must not only be factors, but prime as well.

As a quick reference, all of the prime numbers up to fifty are illustrated below in Figure 04.

The simplest way to find the prime factorization of 63 is to make a factor tree like the one shown in Figure 05 below:

Now we can see that the prime factorization of 63 is:

• 63 = 3 × 3 × 7

That’s it! We've just finished finding both the factors of 63 and the prime factorization of 63.

Knowing how to find the factors of a number is an important and incredibly useful math skill that can help you in elementary math, algebra, and beyond, so make sure that you feel comfortable with finding the factors of a number before moving onto another topic.

Keep Learning:

• What are the factors of 18?

• What are the factors of 27?

• What are the factors of 30?

• What are the factors of 147?

Search Tags: factors of 63, prime factorization of 63, what are the factors of 63, factor of 63, prime factors of 63, all factors of 63, what is the prime factorization of 63, what are factors of 63