How to Add Fractions in 3 Easy Steps
Math Skills: How to add fractions with the same denominator and how to add fractions with different denominators
Knowing how to add fractions is an important and fundamental math skill.
Since fractions are a critically important math topic, understanding how to add fractions is a fundamental building block for mastering more complex math concepts that you will encounter in the future.
(Looking to learn how to subtract fractions? Click here to access our free guide)
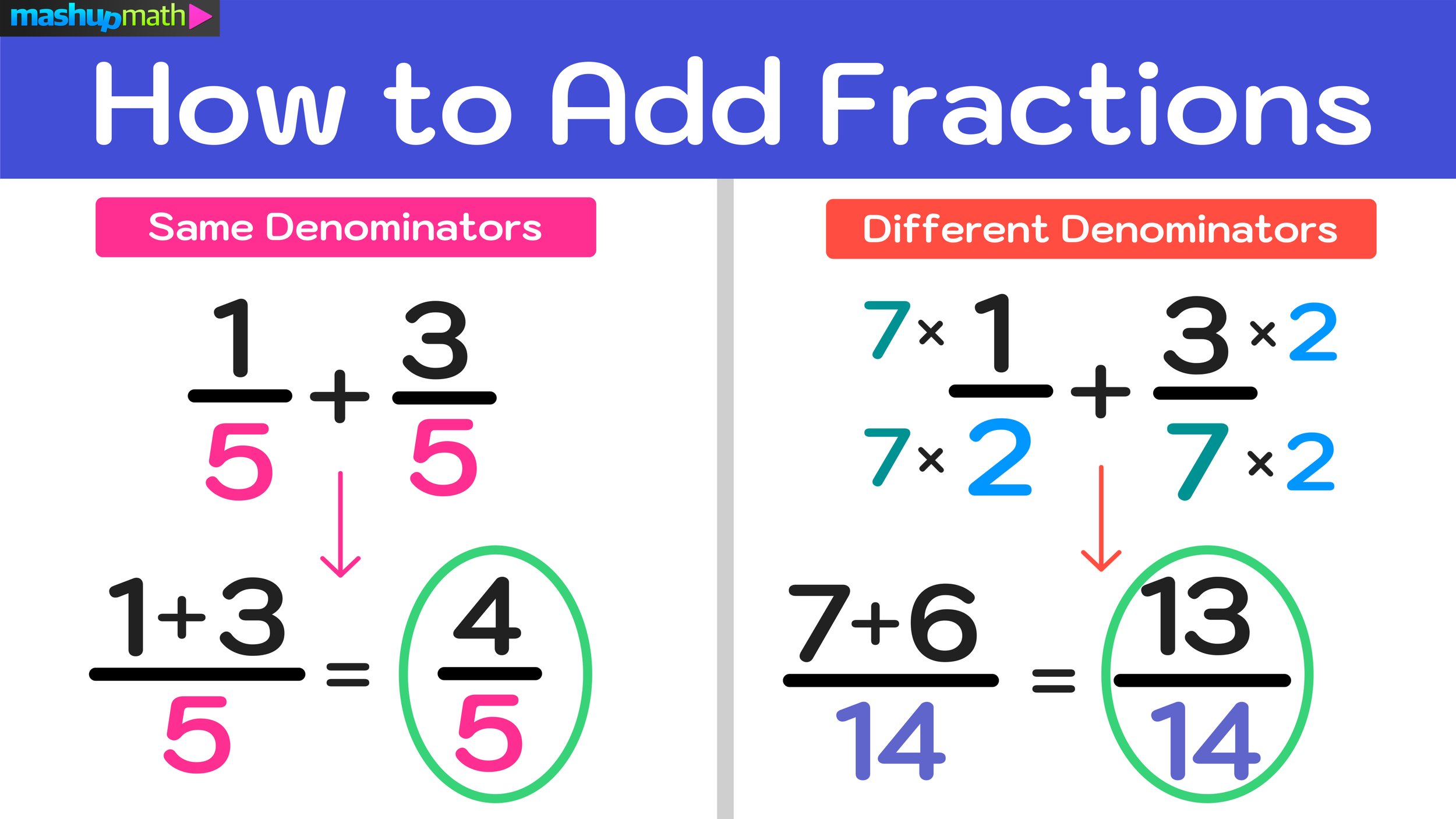
Luckily, learning how to add fractions with like and unlike (different) denominators is a relatively simple process. The free How to Add Fractions Step-by-Step Guide will teach you how to add fractions when the denominators are the same and how to add fractions with different denominators using a simple and easy 3-step process.
This guide will teach you the following skills (examples included):
But, before you learn how to add fractions, let’s do a quick review of some key characteristics and vocabulary terms related to fractions before we move onto a few step-by-step examples of how to add fractions.
Are you ready to get started?
How to Add Fractions: Definitions and Vocabulary
In order to learn how to add fractions, it is imperative that you understand the difference between a numerator and a denominator.
Definition: The numerator of a fraction is the top number in the fraction. For example, in the fraction 3/4, the numerator is 3.
Definition: The denominator of a fraction is the bottom number in the fraction. For example, in the fraction 3/4, the denominator is 4.
Pretty simple, right? These terms are visually represented in Figure 01 below. Make sure that you understand the difference between the numerator and the denominator of a fraction before moving forward in this guide. If you mix them up, you will not learn how to add fractions correctly.
Figure 01: The numerator is the top number of a fraction, and the denominator is the bottom number of a fraction.
Now that you know the difference between the numerator and the denominator of a fraction, you are ready to learn how to identify whether or not a given problem involving adding fractions falls into which of the following categories:
Like Denominators (the denominators are the same)
Unlike Denominators (the denominators are different)
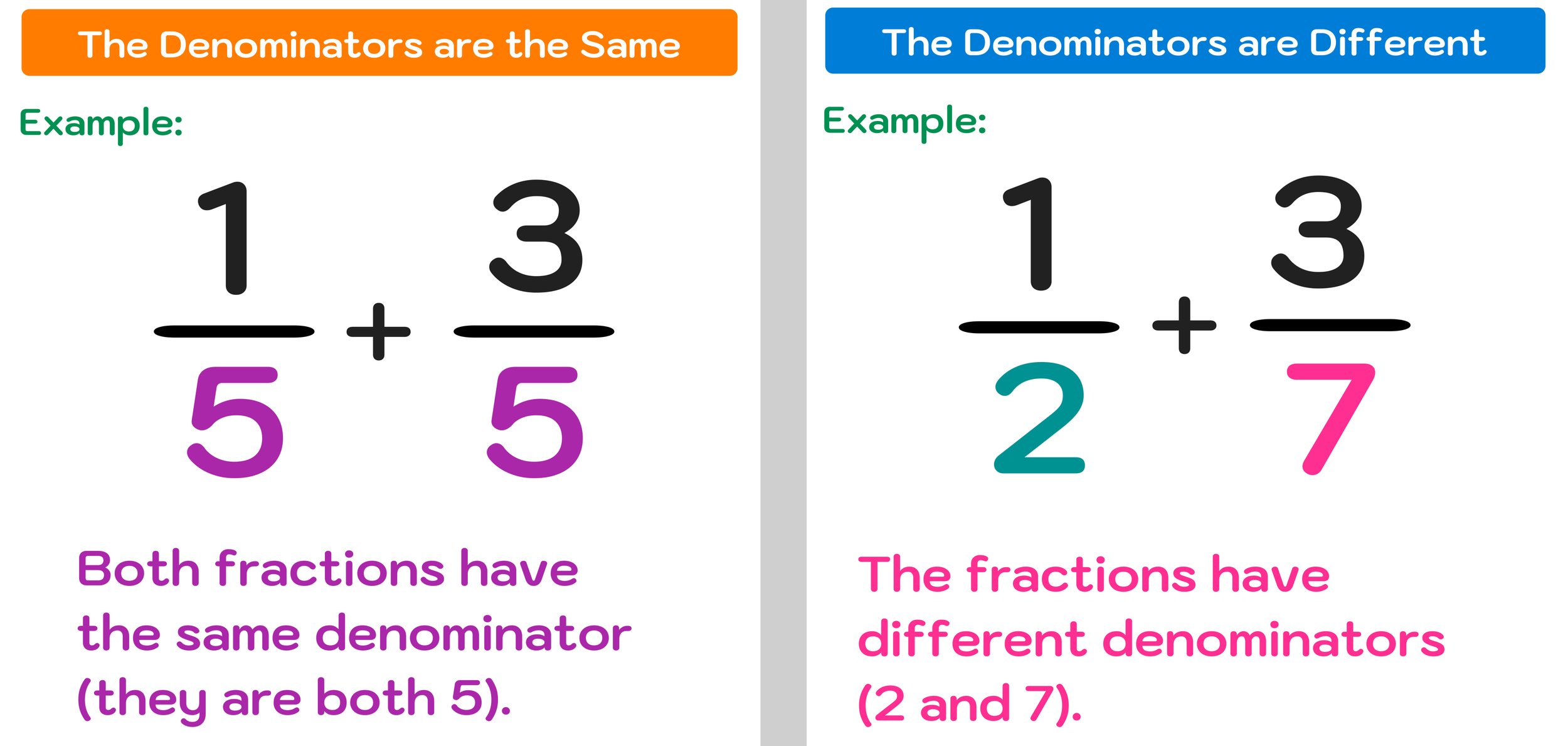
Fractions with like denominators have bottom numbers that equal the same value. For example, in the case of 1/5 + 3/5, you would be adding fractions with like denominators since both fractions have a bottom number of 5.
Conversely, fractions with different (or unlike) denominators have bottom numbers that do not equal the same value. For example, in the case of 1/2 + 3/7, you would be adding fractions with different denominators since the fractions do not share a common denominator (one has a denominator of 2 and the other has a denominator of 7).
These examples are featured in Figure 02 below.
Figure 02: In order to learn how to add fractions, you must be able to identify when the fractions have denominators that are the same and when they have different denominators.
Again, this concept should be simple, but a quick review was required because you will need to be able to identify whether or not a fractions addition problem involves like or unlike denominators in order to solve it correctly.
Now, let’s move onto a few examples.
How to Add Fractions with Like Denominators
How to Add Fractions with Like Denominators: Example #1
Example #1: 1/4 + 2/4
Our first example is rather simple, but it is perfect for learning how to use our easy 3-step process, which you can use to solve any problem that involves adding fractions:
Step One: Identify whether the denominators are the same or different.
Step Two: If they are the same, move onto Step Three. If they are different, find a common denominator.
Step Three: Add the numerators and find the sum.
Okay, let’s take our first attempt at using these steps to solve the first example: 1/4 + 2/4 = ?
Step One: Identify whether the denominators are the same or different.
Clearly, the denominators are the same since they both equal 4.
Step Two: If they are the same, move onto Step Three. If they are different, find a common denominator.
Since the denominators are the same, you can move onto Step Three.
Step Three: Add the numerators and find the sum.
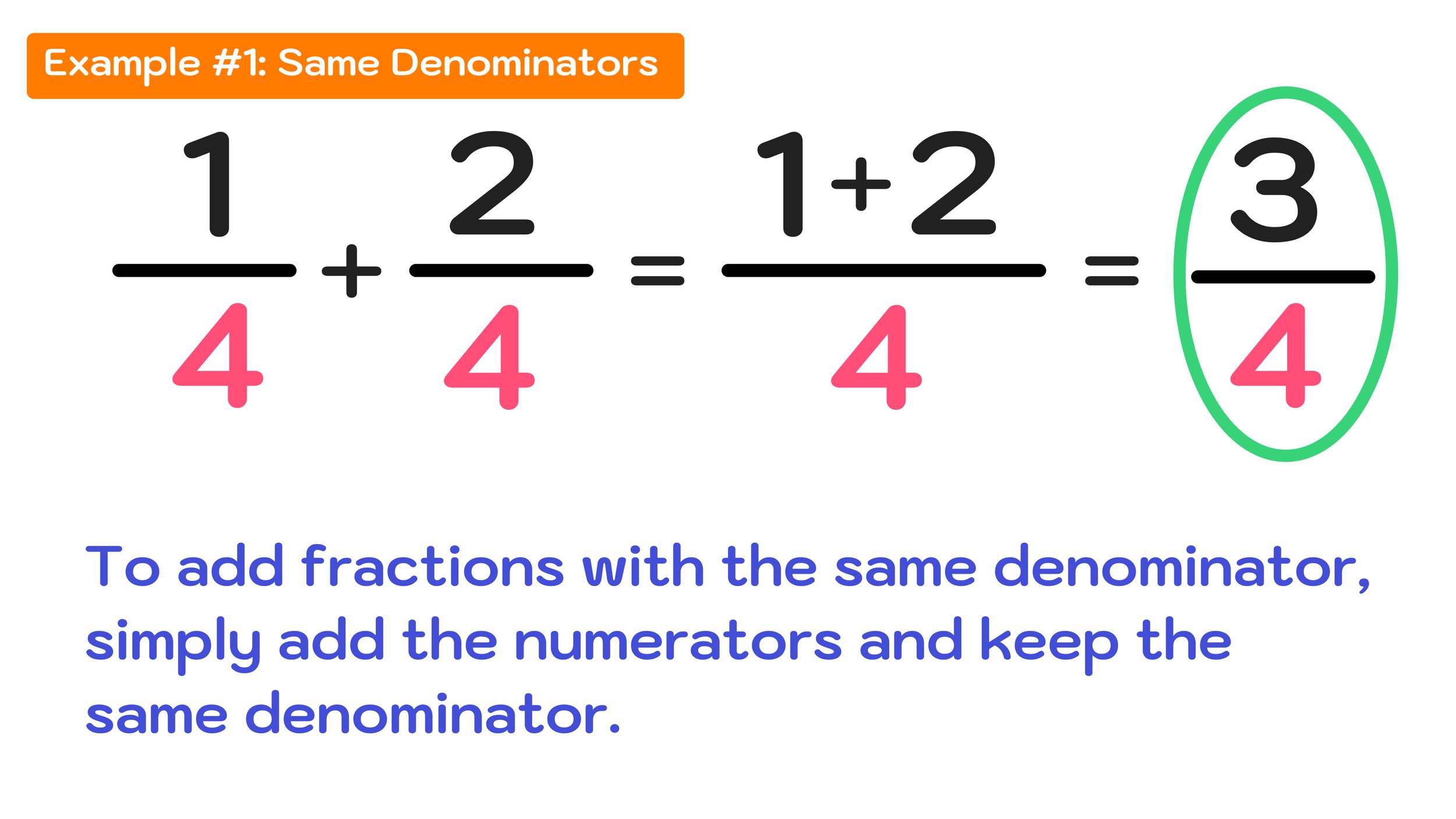
To complete this first example, simply add the numerators together and express the result as one single fraction with the same denominator as follows:
1/4 + 2/4 = (1+2)/4 = 3/4
Since 3/4 can not be simplified further, you can conclude that…
Final Answer: 3/4
This process is summarized in Figure 03 below.
Figure 03: How to Add Fractions: The process is relatively simple when the denominators are the same.
As you can see from this first example, learning how to add fractions when the denominators are the same is very simple.
To add fractions with the same denominator, simply add the numerators and keep the same denominator.
Let’s take a look at one more example of adding fractions when the denominators are the same before you learn how to add fractions with different denominators.
How to Add Fractions with Like Denominators: Example #2
Example #2: 2/9 + 4/9
To solve this second example, let’s apply the 3-step process like we did in the previous example as follows:
Step One: Identify whether the denominators are the same or different.
The denominators in this example are the same since they both equal 9.
Step Two: If they are the same, move onto Step Three. If they are different, find a common denominator.
Again, you can skip the second step because the denominators are the same.
Step Three: Add the numerators and find the sum.
The final step is to add the numerators and keep the denominator the same:
2/9 + 4/9 = (2+4)/9 = 6/9
In this case, 6/9 is the correct answer, but this fraction can actually be reduced. Since both 6 and 9 are divisible by 3, 6/9 can be reduced to 2/3.
Final Answer: 2/3
This process is summarized in Figure 04 below.
Figure 04: How to Add Fractions: 6/9 can be reduced to 2/3
Next, let’s learn how to add fractions with different denominators.
How to Add Fractions with Different Denominators
How to Add Fractions with Different Denominators: Example #1
Example #1: 1/3 + 1/4
Step One: Identify whether the denominators are the same or different.
In this case, the denominators are different (one is 3 and the other is 4)
Step Two: If they are the same, move onto Step Three. If they are different, find a common denominator.
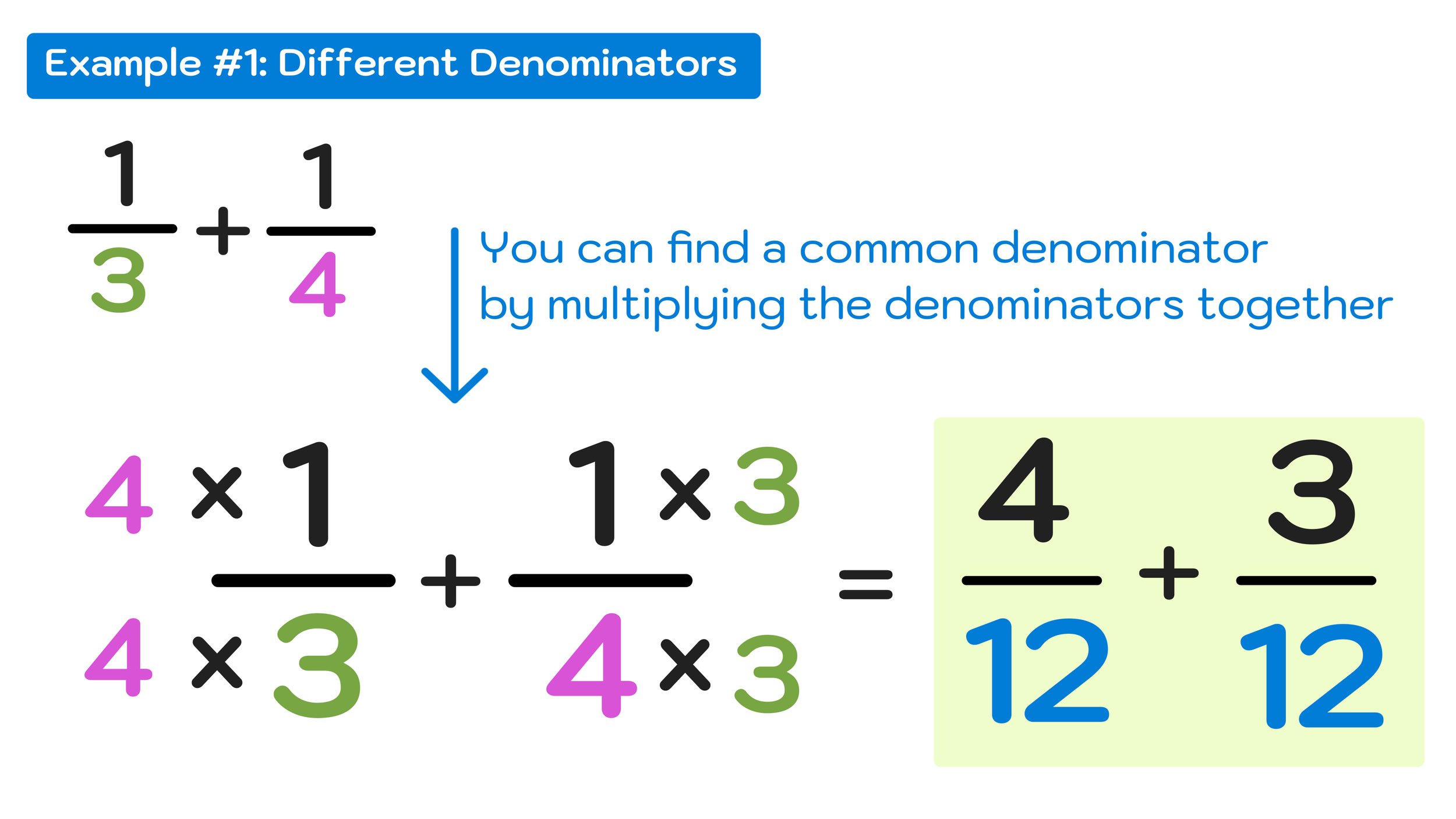
For this example, you can not skip the second step. Before you can continue on, you will need to find a common denominator—a number that both denominators can divide into evenly.
The easier way to do this is to multiply the denominator of the first fraction by the second fraction and the denominator of the second fraction by the first fraction (i.e. multiply the denominators together).
1/3 + 1/4 (4x1)/(4x3) + (3x1)/(3x4) = 4/12 + 3/12
This process is shown in Figure 05 below.
Figure 05: How to Add Fractions with Different Denominators: Get a common denominator by multiplying the denominators together.
(If you need some help with multiplying fractions, click here to access our free guide).
Now, we have transformed the original question into a scenario involving adding two fractions that do have common denominators, which means that the hard work is over and we can solve by adding the numerators and keep the same denominator:
4/12 + 3/12 = (4+3)/12 = 7/12
Since 7/12 can not be simplified further, you can conclude that…
Final Answer: 7/12
Figure 06: Once you have common denominators, you can simply add the numerators together and keep the same denominator.
Now, let’s work through one final example of adding fractions with unlike denominators.
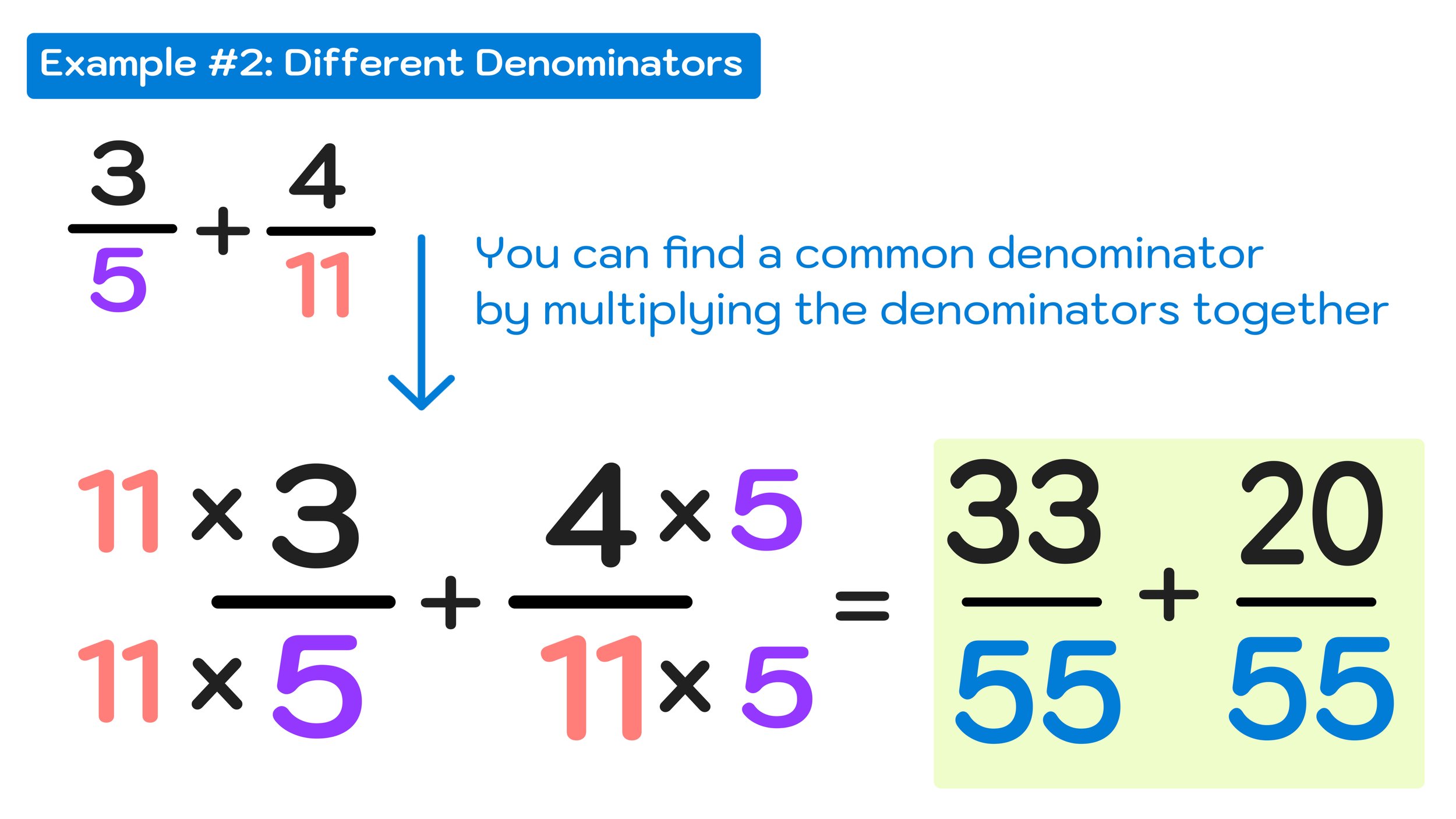
How to Add Fractions with Different Denominators: Example #2
Example #1: 3/5 + 4/11
For this last example, let’s again apply the 3-step process:
Step One: Identify whether the denominators are the same or different.
The denominators are clearly different (one is 5 and the other is 11)
Step Two: If they are the same, move onto Step Three. If they are different, find a common denominator.
Just like the last example, the second step is to find a common denominator by multiplying the denominators together as follows:
3/5 + 4/11 (11x3)/(11x5) + (5x4)/(5x11) = 33/55 + 20/55 = 53/55
This process is shown in Figure 07 below.
Figure 07: How to Add Fractions with Different Denominators: Get a common denominator by multiplying the denominators together.
Finally, now that you have common denominators, you can solve the problem as follows:
33/55 + 20/55 = (33+20)/55 = 53/55
Since there is no value that divides evenly into both 53 and 55, you can not simplify the fraction further.
Final Answer: 53/55
Figure 08: How to Add Fractions with Different Denominators: 53/55 can not be simplified further.
Conclusion: How to Add Fractions
To add fractions with the same denominator, simply add the numerators (top values) and keep the same denominator (bottom value).
To add fractions with different denominators, you need to find a common denominator. A common denominator is a number that both denominators can divide into evenly.
You can solve problems involving adding fractions for either scenario by applying the following 3-step process:
Step One: Identify whether the denominators are the same or different.
Step Two: If they are the same, move onto Step Three. If they are different, find a common denominator.
Step Three: Add the numerators and find the sum.
Keep Learning:
Search Tags: how to add fractions, how to add fractions with different denominators, how to add fraction , how to add fractions with unlike denominators, how to. add fractions, how to add fractions with, how to add a fraction