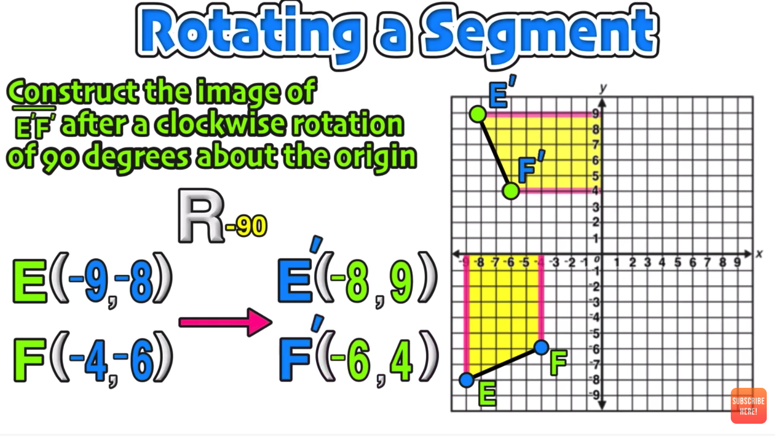
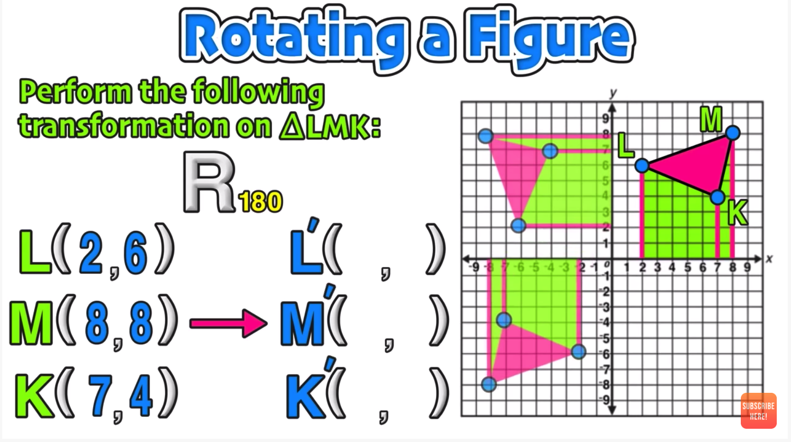
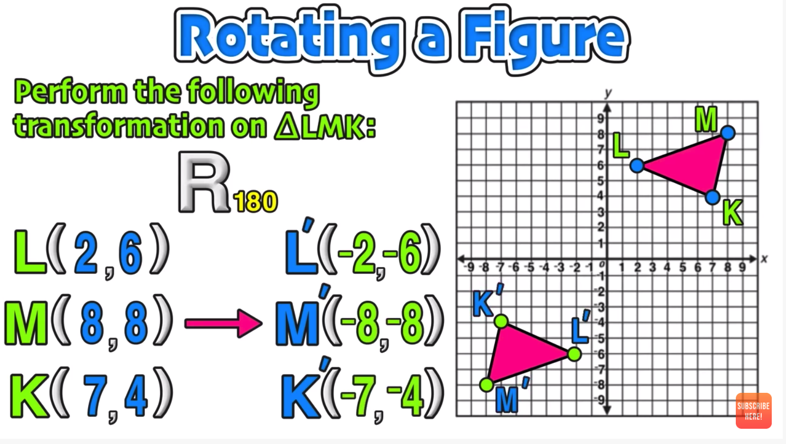
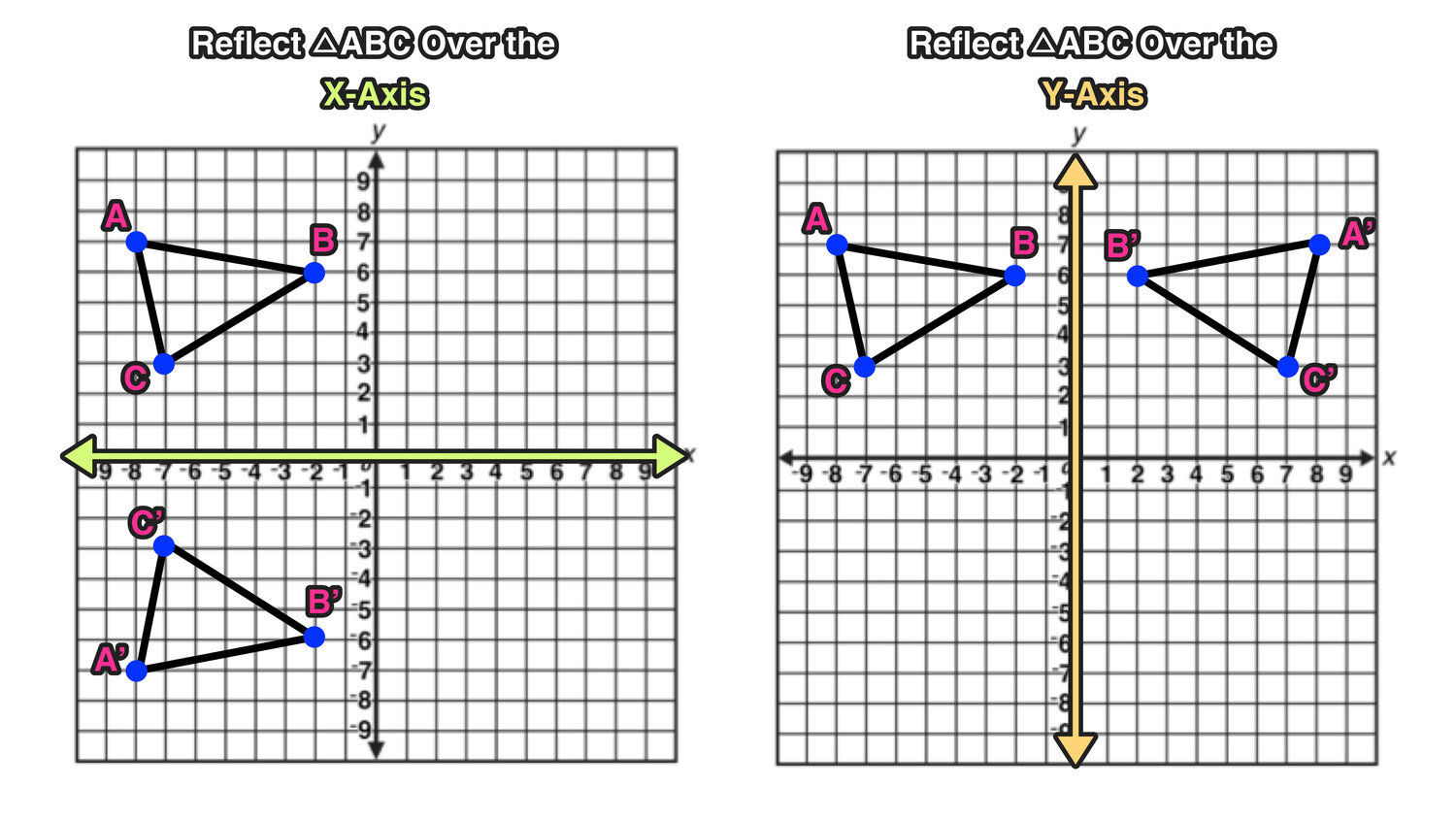
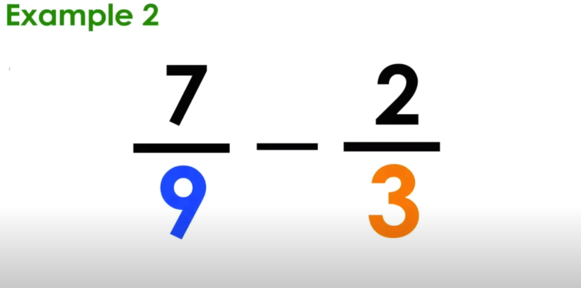Free Math Sheets for 4th Grade! (Easy to Print)

May 1, 2020

Free Math Sheets for 4th Grade!

Are you looking for the best free math sheets for 4th grade students?

The following shares over 100 completely free math sheets for 4th grade. They are easy to print and share at home or in the classroom, and they all include answer keys!

Enjoy!

Want free 4th grade math resources in your inbox every week? Join our free mailing list to get yours!

1.) Math Sheets for 4th Grade: PDF Worksheets and Answer Keys

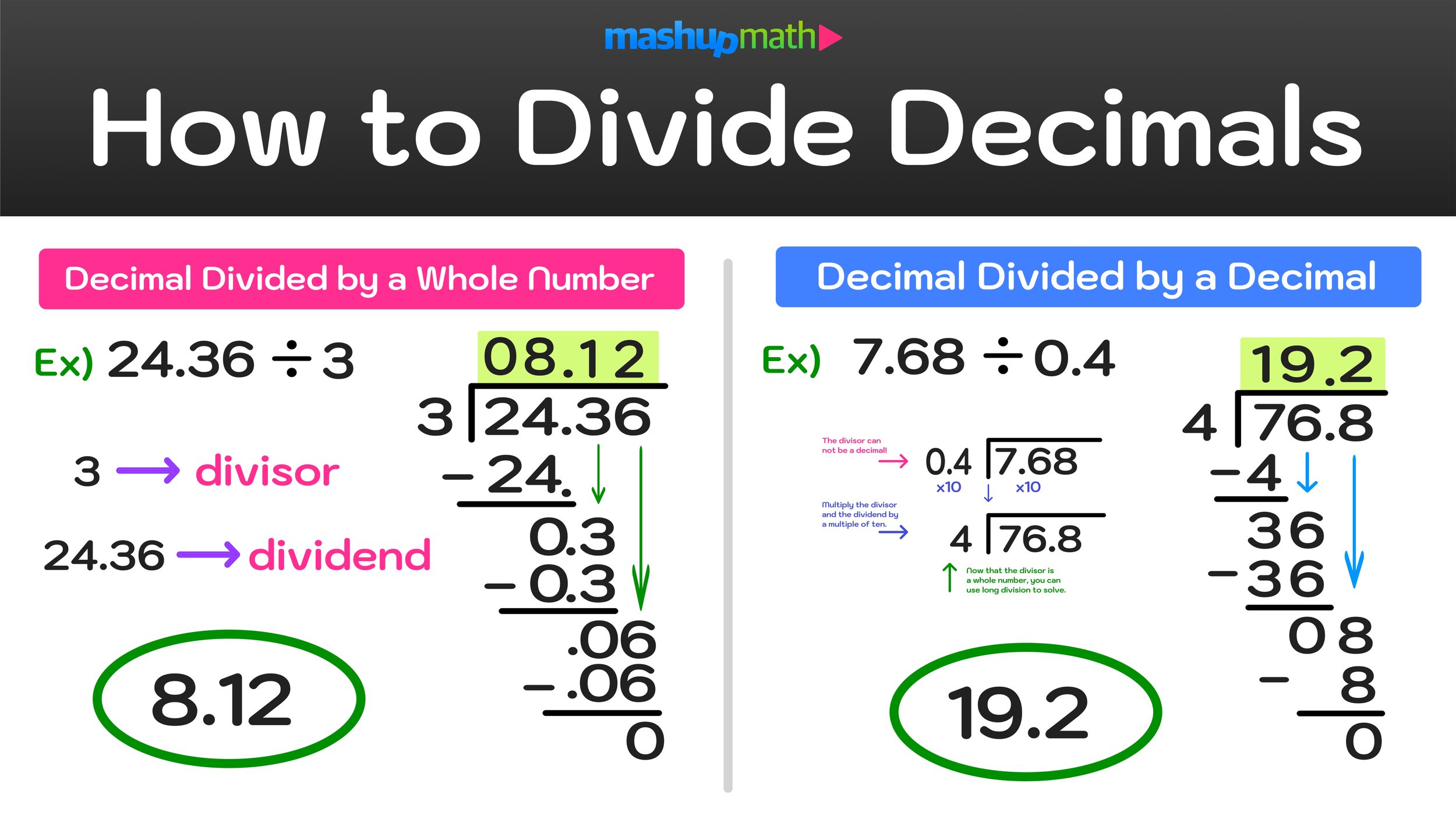
The following collection shares over 100 free and easy to print math sheets for 4th grade on topics including fractions, place value, unit conversion, multiplication, division, and more! Plus every worksheet includes a free answer key.

Just scroll through the list of topics, select any one that you want, and click on it to download the pdf file and answer key (yes, it’s that easy!).

Click here to access the free library of 4th grade math sheets.

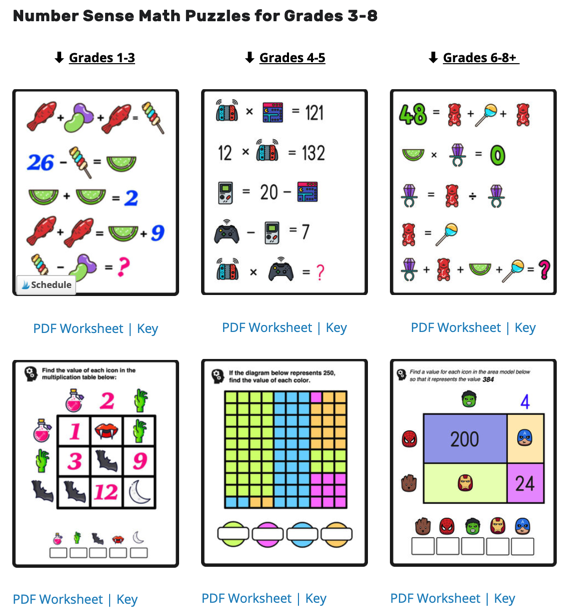
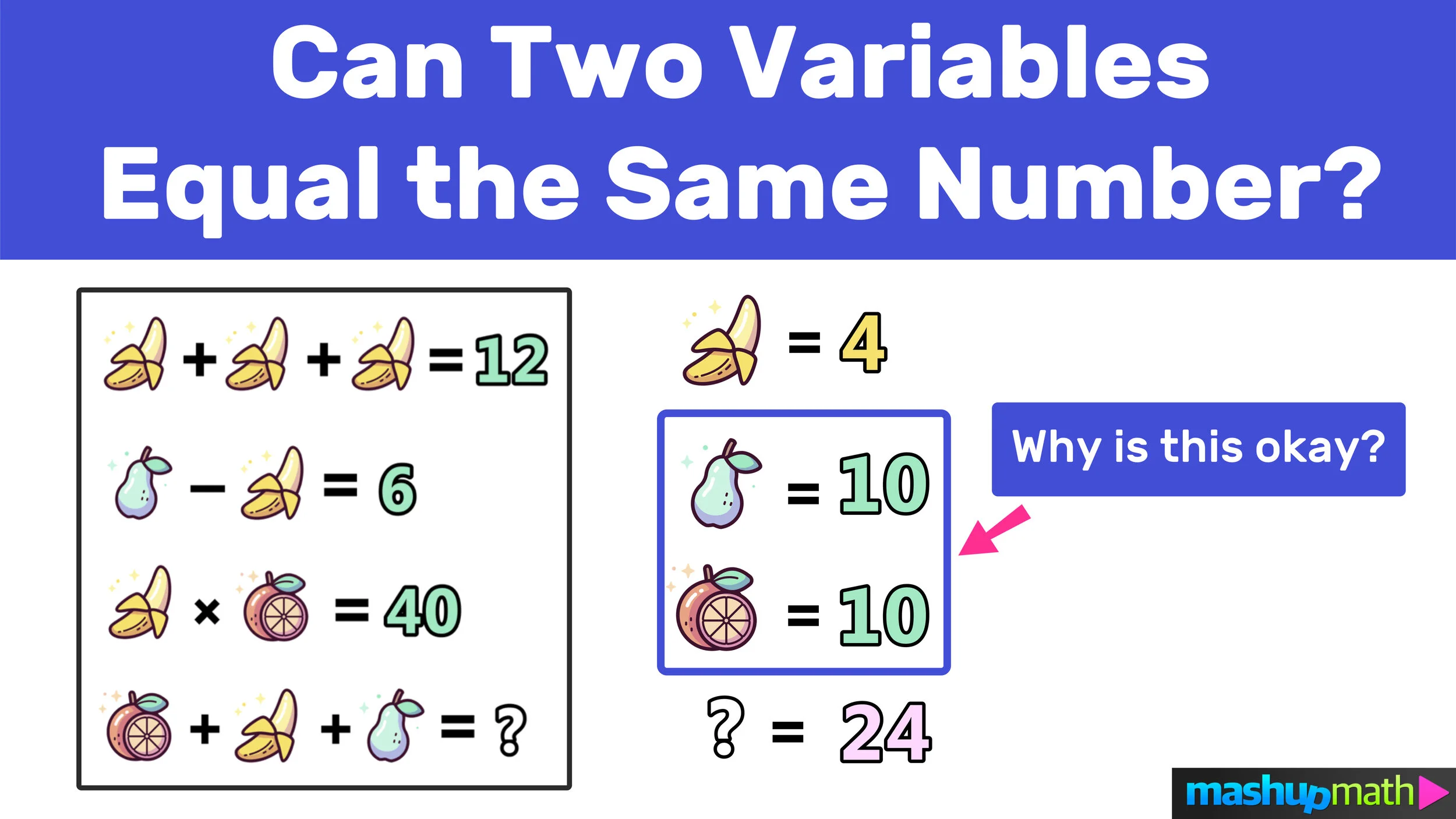
2.) Math Sheets for 4th Grade: Puzzles, Riddles, and Brain Teasers

Typical math sheets are great for giving students extra practice, but they can get pretty boring and repetitive after a while. If you’re looking to mix up your lesson plans every now and then, check out this collection of super fun math sheets for 4th grade that include math puzzles, riddles, and brain teasers!

Trust me, your kids will love them!

Click here to get your math puzzles, riddles, and brain teasers for 4th grade math students!

3.) Math Sheets for 4th Grade: Word Problems

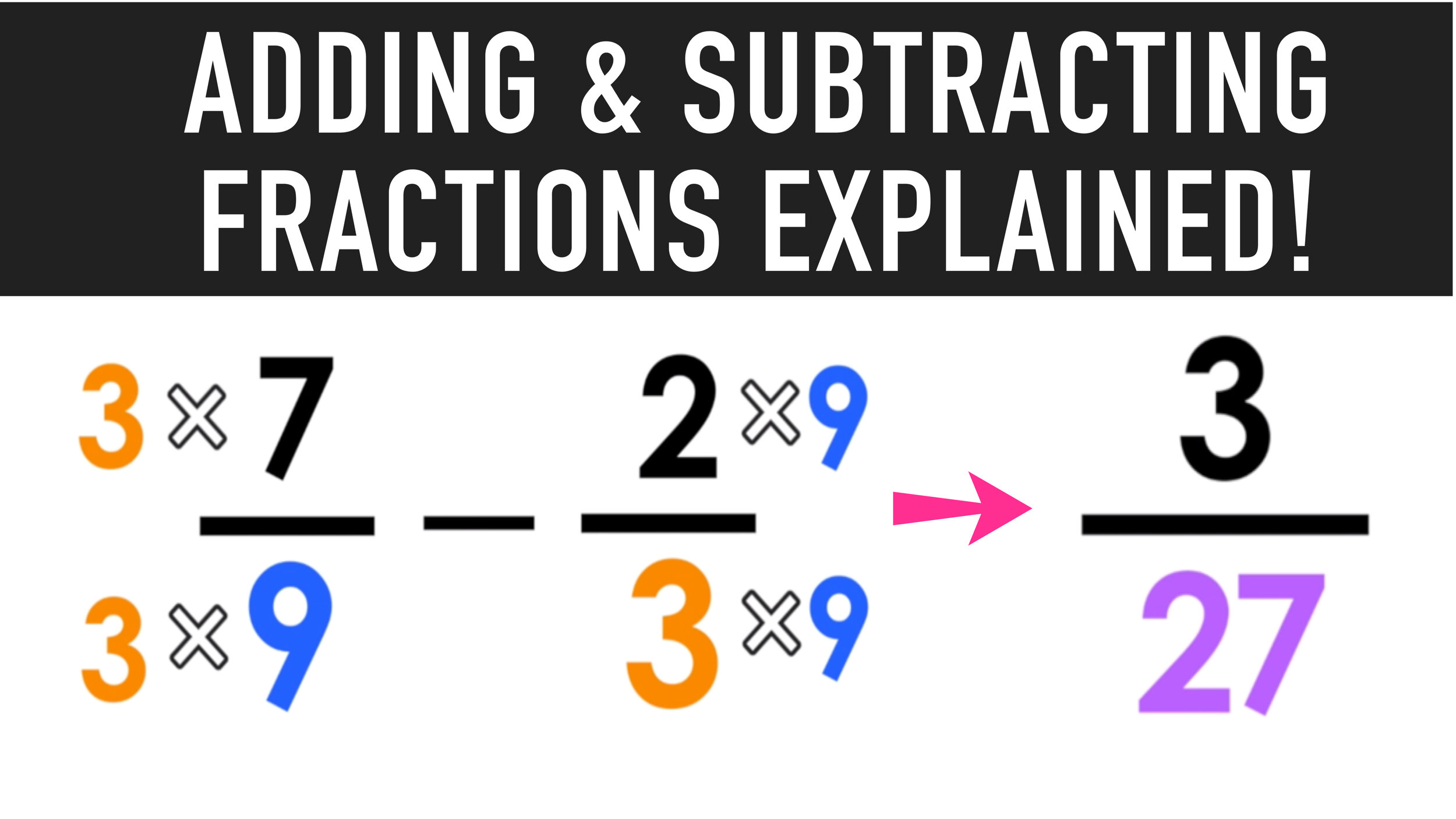
Are you looking for engaging multi-step math sheets for 4th grade problems with answers to add to your upcoming lesson plans? The following collection of free 4th grade maths word problems worksheets cover topics including addition, subtraction, multiplication, division, mixed operations, fractions, and decimals.

Students need to gain a strong understanding of place value in order to understand the relationship between digits and how these relationships apply to operations including addition, subtraction, multiplication, division, and more.

Click here to get your 4th grade math word problems

Looking for more math activities and resources other than math sheets for 4th grade?

Here are some more 4th-grade math resources that you will love!

Featured

Free Printable Math Puzzles for Middle School Students

How to Divide Decimals Explained—Step-by-Step Examples and Tutorial

Are You Using This Genius Strategy for Math Writing?

10 Free Counting Worksheets for Kindergarten

Can Different Variables Have the Same Value?

5 Logical Puzzles That Will Bend Your Brain

10 Free Kindergarten Math Worksheets (PDF Downloads)

10 Free Maths Puzzles with Answers!

7 Super Fun Math Logic Puzzles for Kids!

What Math Teaching Strategies Work Best? 16 Math Education Experts Share Their Suggestions.

10 Super Fun Math Riddles for Kids! (with Answers)

Share your ideas, questions, and comments below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

By Anthony Persico

Anthony is the content crafter and head educator for YouTube's MashUp Math . You can often find me happily developing animated math lessons to share on my YouTube channel . Or spending way too much time at the gym or playing on my phone.

Comment

3 Comments

Free Decimal to Fraction Chart (Printable PDF)

May 1, 2020

Free Decimal to Fraction Conversion Chart

Are you looking for an easy reference chart for making decimal to fraction conversions?

Use the link below to download your free decimal to fraction chart as an easy-to-print PDF file.

Looking to learn how to convert decimals to fractions without a chart? Check out this free converting decimal to fraction in 3 easy steps lesson guide!

Click here to download your free Decimal to Fraction Conversion Chart

Learn How to Convert Decimal to Fraction

The following video lesson shares an easy 3-step method for converting a decimal to a fraction without a decimal to fraction chart!

If you like the video, please give it a thumbs up and leave a comment!

Need help with decimal to fraction conversions with rulers and measurement?

In addition to today’s free decimal to fraction chart, here is a free tutorial on how to read a ruler:

Decimal to Fraction Chart Uses

You can use the decimal and fraction chart above as a reference to quickly make conversions between decimals and fractions.

Be sure to print it out and keep it close by when you are working on problems that require you to convert decimals to fractions or vice versa.

Tags: math,maths,converting decimals to fractions,convert decimal to fraction,decimal to fraction,decimal to fraction in simplest form,decimal to fraction calculator,decimal to fraction math antics,decimal to fraction conversion,decimal to fraction khan academy,decimal to fraction thousandths,decimal to fraction mixed number,decimal to fraction chart,fractions and decimals,improper fractions,simplest form,how to convert decimals to fractions,decimal to fraction ti 84

More Free Decimals and Fractions Resources

How to Add and Subtract Fractions (Step-by-Step)

April 30, 2020

How to Add and Subtract Fractions Explained!

How can you add and subtract fractions with different denominators?

Learn how to solve these kinds of problems.

Welcome to this free lesson guide that accompanies this Adding and Subtracting Denominators with Unlike Denominators video lesson, where you will learn how to add and subtract fractions:

Adding Fractions
Subtracting Fractions
Unlike Denominators
How to add and subtract fractions with the same denominator
How to add and subtract fractions with different denominators

This How to Add and Subtract Fractions with Unlike Denominators: Complete Guide includes several examples, a step-by-step tutorial, an animated video mini-lesson, and a free worksheet and answer key.

*This lesson guide accompanies our animated Adding and Subtracting Fractions with Unlike Denominators on YouTube.

Want more free math lesson guides and videos? Subscribe to our channel for free!

Before you learn how to add and subtract fractions you need to understand some key vocabulary first.

Adding and Subtracting Fractions with the Same Denominator

Let’s start by reviewing the difference between a numerator and a denominator:

How to Add and Subtract Fractions with Like Denominators

In the above example, 1/5 and 3/5 have common denominators (both equal 5). To add them together, you just have to add the numerators together and leave the denominator alone as follows:

Since 4/5 can not be simplified any further, you can conclude that:

1/5 + 3/5 = 4/5

But what about when the denominators are not the same?

Adding and Subtracting Fractions with Unlike Denominators

How do you add and subtract fractions when the denominators are different?

You can use the following 3-step process for adding and subtracting fractions (with and without common denominators).

Subtracting Fractions with Unlike Denominators Example

STEP ONE: Get a common denominator.

STEP TWO: Add or subtract the numerators.

STEP THREE: Simplify the result if needed.

Notice that 3/27 can be simplified, since the numerator and denominator are both divisible by 3.

And that’s all there is to it!

Final Answer:

Adding and Subtracting Fractions with Unlike Denominators: Video Tutorial

Still confused? Check out the animated video lesson below:

Check out the video lesson below to learn more about adding and subtracting fractions and for more free practice problems:

Keep Learning with More Free Lesson Guides:

Featured

Log Rules Explained! (Free Chart)

Exponent Rules Explained! (Free Chart)

How to Factor a Trinomial in 3 Easy Steps

Calculating Percent Change in 3 Easy Steps

What is Point-Slope Form in Math?

5 Point-Slope Form Examples with Simple Explanations

Calculating Percent Decrease in 3 Easy Steps

Where is the hundredths place value in math?

How to Solve Compound Inequalities (in 3 Easy Steps)

Parent Functions and Parent Graphs Explained!

Have thoughts? Share your thoughts in the comments section below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

By Anthony Persico

Anthony is the content crafter and head educator for YouTube's MashUp Math. You can often find me happily developing animated math lessons to share on my YouTube channel . Or spending way too much time at the gym or playing on my phone.

6 Comments

Comment

Geometry Transformations: Rotations 90, 180, 270, and 360 Degrees!

April 30, 2020

Performing Geometry Rotations: Your Complete Guide

The following step-by-step guide will show you how to perform geometry rotations of figures 90, 180, 270, and 360 degrees clockwise and counterclockwise and the definition of geometry rotations in math! (Free PDF Lesson Guide Included!)

Welcome to this free lesson guide that accompanies this Geometry Rotations Explained Video Tutorial where you will learn the answers to the following key questions and information:

What is the geometry rotation definition and what is the definition of rotation in math?
How to perform clockwise and counterclockwise rotations
How can you rotate a triangle about the origin?
Several geometry rotation examples

This Complete Guide to Geometry Rotations includes several examples, a step-by-step tutorial, a PDF lesson guide, and an animated video tutorial.

*This lesson guide accompanies our animated Geometry Transformations: Rotations Explained! video.

Want more free math lesson guides and videos? Subscribe to our channel for free!

Rotation Geometry Definition

Before you learn how to perform rotations, let’s quickly review the definition of rotations in math terms.

Rotation Geometry Definition: A rotation is a change in orientation based on the following possible rotations:

90 degrees clockwise rotation
90 degrees counterclockwise rotation
180 degree rotation
270 degrees clockwise rotation
270 degrees counterclockwise rotation
360 degree rotation

Note that a geometry rotation does not result in a change or size and is not the same as a reflection!

Clockwise vs. Counterclockwise Rotations

There are two different directions of rotations, clockwise and counterclockwise:

Clockwise Rotations (CW) follow the path of the hands of a clock. These rotations are denoted by negative numbers.

Counterclockwise Rotations (CCW) follow the path in the opposite direction of the hands of a clock. These rotations are denoted by positive numbers.

Clockwise rotations are denoted by negative numbers.

Counterclockwise rotations are denoted by positive numbers.

Note that the direction of rotation (CW or CCW) doesn’t matter for 180 and 360-degree rotations, since they will both bring you to the same spot (more on this later).

Geometry Rotation Notation

Note that the following notation is used to show what kind of rotation is being performed.

For example, Figure 1 is a rotation of -270 degrees (which is a CW rotation).

Now you are ready to try a few geometry rotation examples!

Geometry Counterclockwise Rotation Examples

Example 01: 90 Degrees Counterclockwise About the Origin

Since 90 is positive, this will be a counterclockwise rotation.

In this example, you have to rotate Point C positive 90 degrees, which is a one quarter turn counterclockwise.

Point C lays in the 1st quadrant.

To perform the 90-degree counterclockwise rotation, imagine rotating the entire quadrant one-quarter turn in a counterclockwise direction.

Note the location of Point C’, the image of Point C after a 90-degree rotation.

And this process could be repeated if you wanted to rotation Point C 180 degrees or 270 degrees counterclockwise:

This example should help you to visually understand the concept of counterclockwise geometry rotations. Next, you will learn the rules for performing counterclockwise rotations.

>>> Before you move on, take some time to visualize what rotations look like on the coordinate plane.

<><><>

Counterclockwise Rotation Rules

You can use the following rules when performing any counterclockwise rotation.

By applying these rules to Point C (3,6) in the last example (Figure 2), you can see how applying the rule creates points that correspond with the graph!

Left: replace x with 3. Right: replace x with -9

These points should look familiar! They are the points that you plotted in the last example!

Geometry Clockwise Rotation Examples

Example 01: 90 Degrees Clockwise About the Origin

Since the rotation is 90 degrees, you will be rotating the point in a clockwise direction.

Now imagine rotating the entire 4th quadrant one-quarter turn in a clockwise direction:

Note the location of Point D’, the image of Point D after a -90-degree rotation.

And this process could be repeated if you wanted to rotation Point D -180 degrees or -270 degrees counterclockwise:

This example should help you to visually understand the concept of clockwise geometry rotations. Next, you will learn the rules for performing clockwise rotations.

>>> Before you move on, take some time to visualize what rotations look like on the coordinate plane.

<><><>

Clockwise Rotation Rules

You can use the following rules when performing any clockwise rotation.

By applying these rules to Point D (5,-8) in the last example (Figure 3), you can see how applying the rule creates points that correspond with the graph!

These points should look familiar! They are the points that you plotted in the last example!

More Geometry Rotations Examples

Example 01: Rotate a Line Segment 90 Degrees Clockwise

You can perform this rotation by using the rules or by doing a visual rotation as follows:

Example 02: Rotate a Triangle 180 Degrees

Note that it doesn’t matter which direction go (CW or CCW) for 180 degrees rotations, since you will end up in the same position either way!

You can perform this rotation by using the rules or by doing a visual rotation as follows:

Free Geometry Rotations Lesson Guide

Looking for more help with geometry rotations?

Click the link below to download your free PDF lesson guide that corresponds with the video lesson below!

Click here to download the Your Free PDF Lesson Guide

Still Confused?

Check out this animated video tutorial on geometry rotations:

Looking for more practice with Geometry Transformations?

Check out the following free resources:

Reflections Over the X- and Y-Axis: Complete Guide

Dilations and Scale Factor: Complete Guide

Keep Learning with More Free Lesson Guides:

Featured

Log Rules Explained! (Free Chart)

Exponent Rules Explained! (Free Chart)

How to Factor a Trinomial in 3 Easy Steps

Calculating Percent Change in 3 Easy Steps

What is Point-Slope Form in Math?

5 Point-Slope Form Examples with Simple Explanations

Calculating Percent Decrease in 3 Easy Steps

Where is the hundredths place value in math?

How to Solve Compound Inequalities (in 3 Easy Steps)

Parent Functions and Parent Graphs Explained!

Have thoughts? Share your thoughts in the comments section below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

By Anthony Persico

Comment

Multiplying Polynomials: The Complete Guide

April 28, 2020

The Best Method for Multiplying Polynomials

The following step-by-step guide will show you how to multiply polynomials using the distributive method and includes 3 examples!

Welcome to this free lesson guide that accompanies this Multiplying Polynomials Made Easy! video tutorial where you will learn the answers to the following key questions and information:

What is the best method for multiplying polynomials?
How to perform multiplication of polynomials
Multiplication of monomials, binomials, and trinomials
Multiplying Polynomials Using the Distributive Property

This Complete Guide to Multiplying Polynomials includes several examples, a step-by-step tutorial and an animated video tutorial.

*This lesson guide accompanies our animated Multiplying Polynomials Made Easy! video.

Want more free math lesson guides and videos? Subscribe to our channel for free!

Multiplying Polynomials Explained!

Before you learn how to multiply polynomials, let’s quickly review some important information:

The Distributive Property

Definition: The distributive property allows for you to multiply a sum by multiplying each term separately and then add all of the products together.

If you understand the distributive property, you will be able to multiply polynomials with ease.

Multiplying Polynomials Examples

Multiplying Polynomials Example 1: Multiplying by a Monomial

In this first example, you will be multiplying a monomial by a trinomial. You can think of this as multiplying one “thing” by “another thing” as follows:

This is where using the distributive property (or distributive method) will help you!

To multiply these polynomials, start by taking the first polynomial (the purple monomial) and multiplying it by each term in the second polynomial (the green trinomial).

This can be done by multiplying 4x^2 by the first term of the green trinomial (Figure 1), then by the second term of the green trinomial (Figure 2) and finally by the third term of the green trinomial (Figure 3).