How to Subtract Fractions with Different Denominators (Step-by-Step)

How to Subtract Fractions with Different Denominators

Math Skills: How to Subtract Fractions with Unlike Denominators in 3 Easy Steps

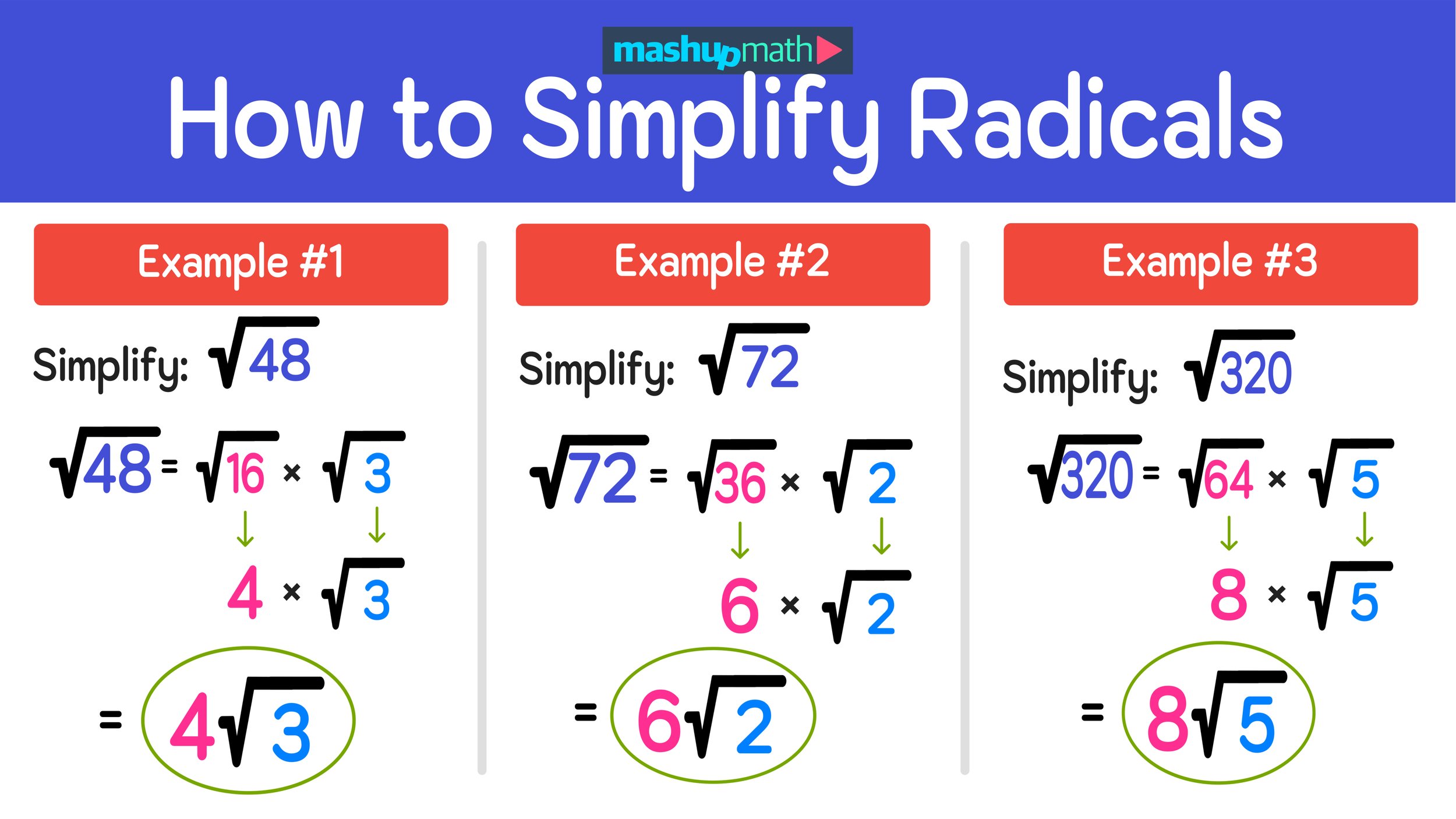
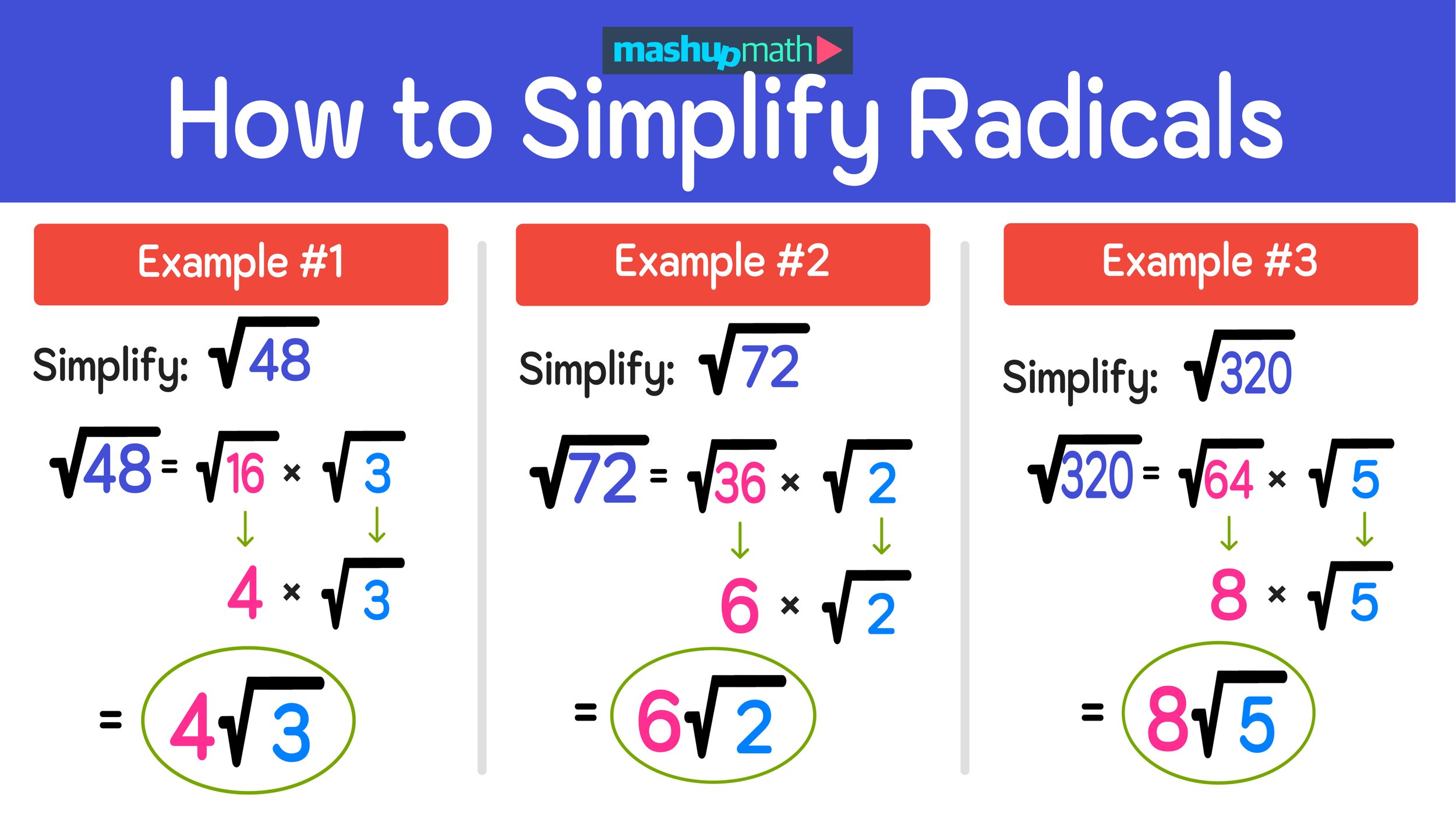
Subtracting Fractions with Different Denominators

Learning how to add and subtract fractions with different denominators is an important math skill that requires a strong understanding of fractions overall. After you learn how to subtract fractions with the same denominator, the next step is to learn how to subtract fractions with different denominators (i.e. how to subtract fractions with unlike denominators).

Subtracting fractions with the same denominator is a pretty straightforward task, but problems involving subtracting fractions with unlike denominators can be more challenging. However, the overall process for subtracting fractions with unlike denominators is a skill that can be mastered by learning a few simple steps and by working through practice problems (and this is exactly what this step-by-step guide will entail).

This free guide on How to Add Subtract with Different Denominators will teach you everything you need to know about subtracting fractions. Together, we will learn a simple 3-step strategy for subtracting fractions that you will be able to use to solve any subtracting fractions with unlike denominators problem.

While we highly recommend that you follow each section of the guide in order, you can click on any of the quick-links below to jump to a specific section.

• Review: Subtracting Fractions with the Same Denominator

• How to Subtract Fractions with Different Denominators: 3-Step Strategy

• How to Subtract Fractions with Different Denominators Example #1

• How to Subtract Fractions with Different Denominators Example #2

• How to Subtract Fractions with Different Denominators Example #3

Before we get started with learning the 3-step strategy or working on the practice problems, we will do a quick recap of fractions and what it means to subtract fractions with unlike denominators.

Recap: Subtracting Fractions with the Same Denominator

Let’s start by recapping two important vocabulary terms related to fractions:

Definition: The numerator of a fraction is the top number. For example, 2/3 has a numerator of 2.

Definition: The denominator of a fraction is the bottom number. For example, 2/3 has a denominator of 3.

Throughout this tutorial, we will make several references to the numerator and denominator of a fraction, so be sure that you have a firm understanding of these two vocabulary terms before going any further.

Figure 01: Every fraction has a top number (numerator) and a bottom number (denominator).

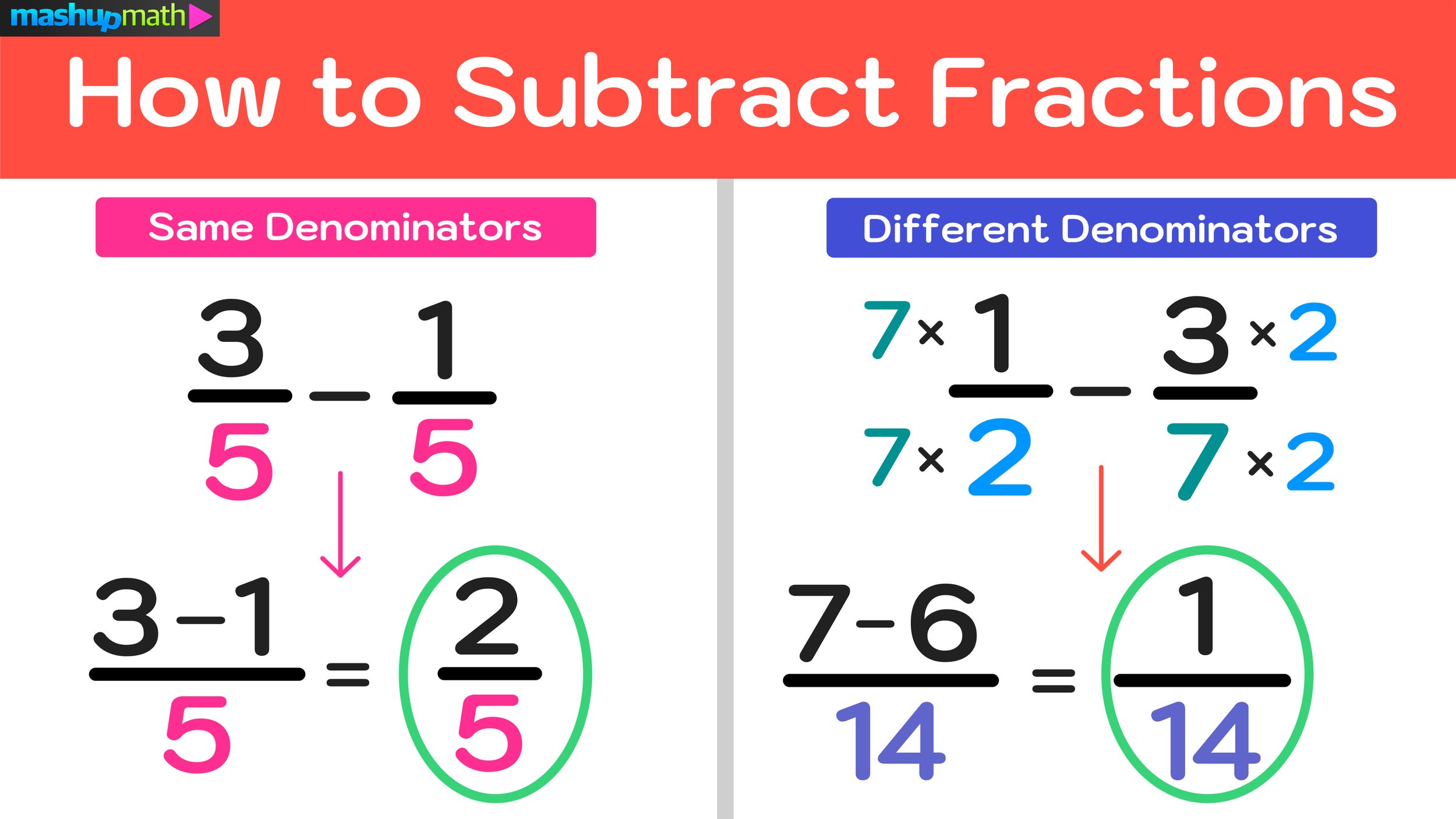
Subtracting fractions with the same denominator is a relatively simple task.

For example, consider the following problem where you have to subtract one fraction from another:

• 3/4 - 1/4 = ?

Both of the fractions, 3/4 and 1/4, have the same denominator (i.e. both fractions have 4 as the denominator).

For examples such as this, if both fractions have a common denominator, all you have to do to solve the problem is to subtract the second numerator from the first numerator and keep the denominator the same. This can be done as follows:

• 3/4 - 1/4 = (3-1)/4 = 2/4

Notice that our result, 2/4, can be simplified to 1/2 (since both the numerator and the denominator share a greatest common factor of 2, we can reduce by dividing both numbers by 2).

So, we can conclude that:

• Final Answer: 3/4 - 1/4 = 1/2

Figure 02 below illustrates how we solved this problem. For a deeper dive into subtracting fractions with the same denominator, we suggest reviewing our free How to Subtract Fractions Student Guide before going any further.

The key takeaway from this review of how to subtract fractions with the same denominator is that whenever you are subtracting fractions with the same denominator, find the difference of the numerators and leave the denominator the same.

Figure 02: Subtracting fractions with the same denominator.

Now we are ready to move onto the next section, which will focus on how to subtract fractions with unlike denominators using a 3-step strategy.

---

How to Subtract Fractions with Unlike Denominators Step-by-Step

First, let’s recall the sample problem from the previous section when we reviewed how to subtract fractions with a common denominator:

• 3/4 - 1/4 = ?

For this problem, we concluded that the answer is 1/2. Now, let’s look at another practice problem where the fractions have unlike denominators:

• 3/4 - 2/8 = ?

Notice that, in this problem, one fraction has a denominator of 4 and the other has a denominator of 8 (i.e. the denominators are different).

However, if we compare both problems, we should notice that they are equivalent to each other. Namely, the second fraction in both problems represent “one-quarter” since 1/4 and 2/8 are equivalent fractions.

What does this mean? Both problems represent the difference of three-quarters and one quarter. However, the first problem has common denominators and the section problem does not.

With this difference in mind, we will go ahead and learn how to use a 3-step strategy for subtracting fractions with unlike denominators to solve this problem to see if we get the same result (i.e. the final answer is 1/2).

Figure 03: The expressions 3/4 - 1/4 and 3/4 - 2/8 are equivalent (i.e. they both represent “three-quarters minus one-quarter.”

How to Subtract Fractions with Unlike Denominators:

• Step One: Find a common denominator by multiplying each fraction by the opposite fraction’s denominator.

• Step Two: Subtract the second numerator from the first numerator and keep the denominator.

• Step Three: Reduce the result if possible.

Now that you know the 3-step strategy for subtracting fractions with unlike denominators, let’s apply them to solving the problem 3/4 - 2/8 = ?.

Step One: Find a common denominator by multiplying each fraction by the opposite fraction’s denominator.

For step one, we have to find a common denominator.

Definition: In math, a common denominator is a common value that the denominators of both fractions can be divided into evenly.

While there are a few ways to find a common denominator between two fractions, the simplest way is to multiply the denominator of the first fraction by the second fraction and vice versa (i.e. multiply the denominator of the second fraction by the first fraction).

For the problem 3/4 - 2/8 = ?, we can use this approach to finding a common denominator as follows:

• 3/4 - 2/8 = (3x8)/(4x8) - (2x4)/(8x4) = 24/32 - 8/32

Now we have a new equivalent expression, 24/32 - 8/32, where the denominators are the same. And, since the denominators of this new equivalent expression are the same, we can say that we have found a common denominator and we can move onto step two.

Figure 04: How to find a common denominator between two fractions.

Step Two: Subtract the second numerator from the first numerator and keep the denominator.

For the second step, we will focus on our new expression where the fractions share a common denominator of 32:

• 3/4 - 2/8 → 24/32 - 8/32

From here, we can solve 24/32 - 8/32 by finding the difference of the numerators and leaving the denominator the same:

• 24/32 - 8/32 = (24-8)/32 = 16/32

Step Three: Reduce the result if possible.

Lastly, we have to check and see whether or not our result, 13/15, can be simplified. Since both 16 and 32 share a greatest common factor of 16, we can divide both the numerator, 16, and the denominator, 32, by 16 to get an answer in reduced form:

• (16÷16)/(32÷16) = 1/2

So, we can conclude that:

Final Answer: 3/4 - 2/8 = 1/2

Figure 05: How to Subtract Fractions with Different Denominators in 3 Steps.

Our final answer should look familiar. Remember that we already knew that our answer would be 1/2 since we know that three-quarters minus one-quarter equals one-half. The key takeaway here is understanding that our 3-step strategy will allow us to correctly solve any problem where you are tasked with subtracting fractions with the same denominator.

Now, let’s go ahead and get some more practice using the 3-step strategy for subtracting fractions with unlike denominators.

---

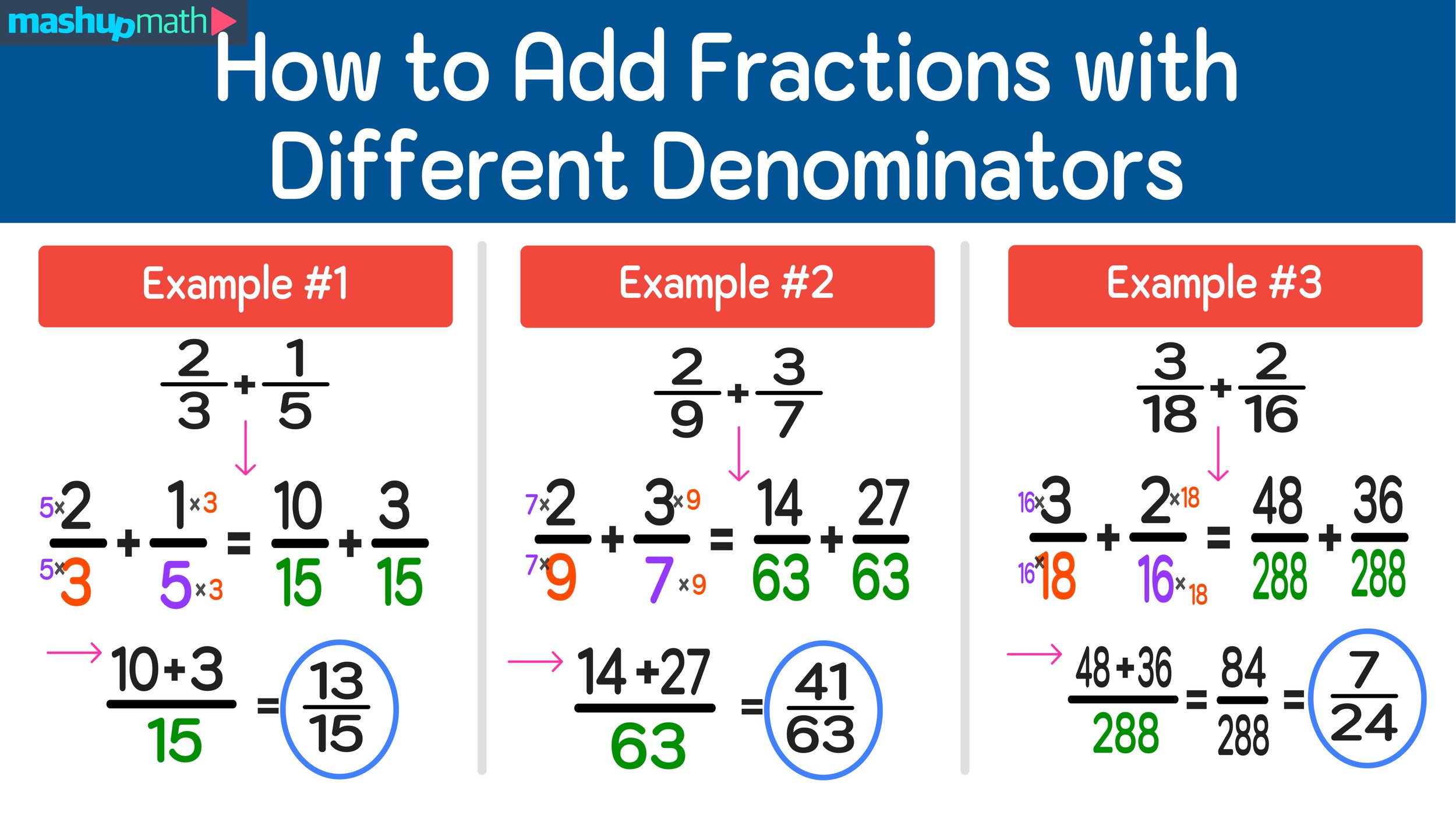
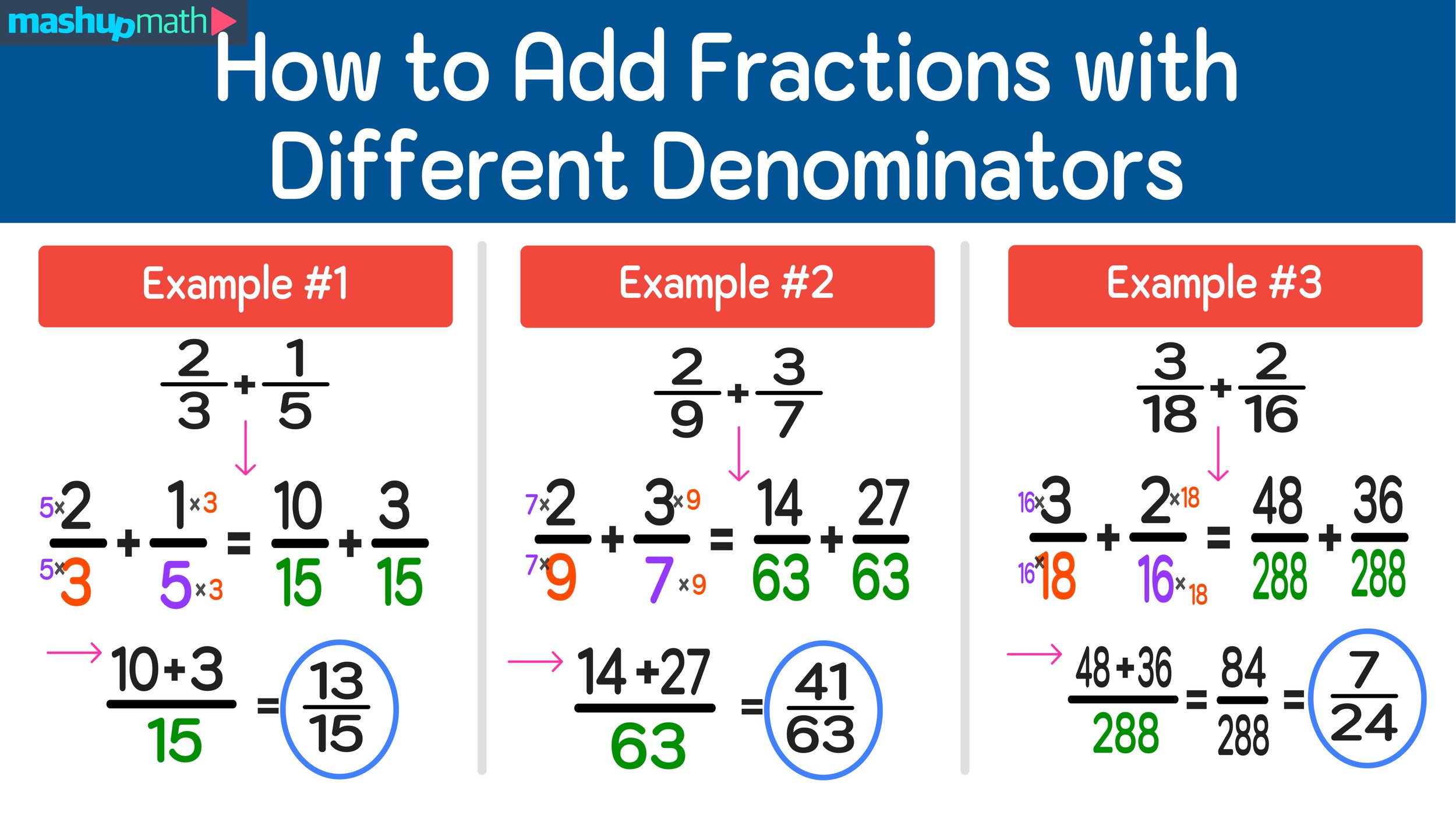
How to Subtract Fractions with Different Denominators Example #1

Example #1: 4/5 - 2/3

For this first example, we want to find the difference between four-fifths (4.5) and two-thirds (2/3). To solve this problem, we can use of 3-step strategy as follows:

Step One: Find a common denominator by multiplying each fraction by the opposite fraction’s denominator.

First, we have to find a common denominator by multiplying the denominator of the first fraction (5) by the second fraction (2/3) and then by multiplying the denominator of the second fraction (3) by the first fraction (4/5):

• 4/5 - 2/3 = (3x4)/(3x5) - (5x2)/(5x3) = 12/15 - 10/15

Notice that we now have a new equivalent expression with common denominators (i.e. both fractions have the same denominator, which, in this case, is 15).

• 4/5 - 2/3 → 12/15 - 10/15

Step Two: Subtract the second numerator from the first numerator and keep the denominator.

For the second step, we can solve 12/15 - 10/15 = ? as follows:

• 12/15 - 10/15 = (12-10)/15 = 2/15

Step Three: Reduce the result if possible.

And now, for the final step, we just have to see if the result, 2/15, can be simplified. Since 2 and 15 do not have any factors in common (other than 1), this fraction is already in reduced form and can not be simplified further. Therefore…

Final Answer: 4/5 - 2/3 = 2/15

The entire 3-step process for solving this first example is illustrated in Figure 06 below:

Figure 06: How to Subtract Fractions with Unlike Denominators

---

How to Subtract Fractions with Unlike Denominators Example #2

Example #2: 3/7 - 2/9

Just like the last example, we can use our 3-step strategy to solve this problem.

Step One: Find a common denominator by multiplying each fraction by the opposite fraction’s denominator.

To find a common denominator, we can multiply the first fraction, 3/7, by the denominator of the second fraction, 9, and then multiply the second fraction, 2/9, by the denominator of the first fraction, 7, as follows:

• 3/7 - 2/9 = (9x3)/(9x7)-(7x2)/(7x9) = 27/63 - 14/63

Now we have found an expression that is equivalent to the original problem:

• 3/7 - 2/9 → 27/63 - 14/63

Step Two: Subtract the second numerator from the first numerator and keep the denominator.

Next, we can go ahead and solve 27/63 - 14/63 as follows:

• 27/63 - 14/63 = (27-14)/63 = 13/63

Step Three: Reduce the result if possible.

For the very last step, we just have to see if our result, 13/63, can be simplified any further. Notice that 13 and 63 do not share any common factors besides 1, so we can conclude that 13/63 can not be simplified and:

Final Answer: 3/7 - 2/9 = 13/63

All of the steps for solving this second example are detailed in Figure 07 below.

Figure 07: Subtracting Fractions Explained!

Are you feeling more confident with using the 3-step strategy to subtract fractions with unlike denominators? If not, let’s go ahead and gain some more experience by working through one more practice problem.

---

How to Subtract Fractions with Different Denominators Example #3

Example #3: 7/8 - 4/24

For this last example, we can again use the 3-step strategy to solve it:

Step One: Find a common denominator by multiplying each fraction by the opposite fraction’s denominator.

First, we can find a common denominator by taking each fraction and multiplying it by the denominator of the opposite fraction:

• 7/8 - 4/24 = (24x7)/(24x8) - (8x4)/(8x24) = 168/192 - 32/192

Now we have a new expression where both fractions share a common denominator:

• 7/8 - 4/24 → 168/192 - 32/192

Step Two: Subtract the second numerator from the first numerator and keep the denominator.

Next, we have to solve:

• 168/192 - 32/192 = (168-32)/192 = 136/192

Step Three: Reduce the result if possible.

One more step! To wrap this problem up, we have to try and simplify 136/192. We know that this fraction can be reduced since both 136 and 192 share 2 as a common factor. However, they actually share a greater common factor, which is 8. So, after we divide the numerator, 136, and the denominator, 192, by 8, we are left with 17/24, and we can conclude that:

Final Answer: 7/8 - 4/24 = 17/24

The complete step-by-step process of solving Example #3 is illustrated in Figure 08 below.

Figure 08: How to Subtract Fractions with Different Denominators

---

Conclusion: How to Subtract Fractions with Different Denominators

Subtracting fractions with unlike denominators is a foundational math skill that every student must master when learning how to perform operations with fractions.

This step-by-step tutorial on subtracting fractions focused on teaching you how to find the difference between two fractions that do not have the same denominator.

To solve problems where you have to subtract fractions with unlike denominators, we used the following 3-step strategy:

• Step One: Find a common denominator by multiplying each fraction by the opposite fraction’s denominator.

• Step Two: Subtract the second numerator from the first numerator and keep the denominator.

• Step Three: Simplify the result if possible.

These three steps were used to solve the three example problems in this guide. They can also be used to solve any subtracting fractions problems that you may come across, so be sure to keep them in your notes and practice using them as often as you can!

Keep Learning:

How to Add Fractions with Different Denominators in 3 Easy Steps

---

Why Every Teacher Should Say "No" More Often

Why Every Teacher Should Say “No” More Often

The importance of setting boundaries and avoiding teacher burnout.

However, as a teacher, you have the right to say '“no” to unreasonable requests without feeling guilty. (Image: Mashup Math MJ)

Dedicated teachers are in short supply and students, parents and administrators are masters of—often unfairly— squeezing every last drop of energy from them.

Teaching is an important and rewarding profession, but the job is also incredibly demanding. Teachers of all grade levels are saddled with an overwhelming amount of daily responsibilities that extend way beyond their duties inside of the classroom. In an effort to be a team player (and often in fear of not letting others down), many teachers struggle with setting healthy boundaries, which often leads to intense exhaustion and burnout before the school year is over.

However, as a teacher, you have the right to say '“no” to unreasonable requests without feeling guilty.

Setting Boundaries

While it’s true that teaching is an inherently selfless profession and many teachers view themselves as servants to their students, schools, and communities, it is also true that well-balanced students have well-balanced teachers. 

It can be hard to say to taking on more responsibilities, but one of the best reasons for learning to say “no” more often is to conserve and prioritize your energy. Your energy is a finite resource and the mental and emotional demands of teaching are taxing enough. Every time to agree to take on an additional responsibility or task, you are giving away more and more of your precious energy, leaving your students with a lesser and more exhausted version of you.

When you learn to set healthy boundaries and decline any requests for commitments that will leave you overextended, you will have more time and energy to focus on your primary task—teaching your students.

When the demands of being a teacher prevent you from setting healthy boundaries, you are at increased risk of reaching a point of burnout. ((Image: Mashup Math MJ)

Burnout and Selective Engagement

What happens to teachers who never learn how to set healthy boundaries? Many of them experience teacher burnout—a persistent state of stress causing feelings of cynicism, detachment, and both mental and physical exhaustion. Teacher burnout leads many teachers to feel helpless, ineffective, and incapable of being an effective educator.

When the demands of being a teacher prevent you from setting healthy boundaries, you are at increased risk of reaching a point of burnout. However, learning how to say “no” and set personal and professional boundaries will allow you to reach a healthy work-life balance where you don’t constantly feel like you are overcommitted or stretched too thin.

The best way to maintain such a work-life balance and healthy boundaries is through something called selective engagement.

Selective engagement is when a teacher chooses to focus on the roles and responsibilities that have the greatest impact on their students and their school community. This doesn’t mean that a teacher should say no to every request that does directly relate to her classroom teaching responsibilities. However, it does mean that teachers must be realistic about how much time and energy that have to give and that they should thoughtfully choose to give their attention to responsibilities that will not lead them to teacher burnout.

One major benefit of setting boundaries is that your students will receive higher quality classroom instruction and lesson planning from a teacher who is able to be energized, present, and focused. (Image: Mashup Math MJ)

Strategies for Saying “No”

Saying “no” can be difficult, which is why many consider it an artform in itself.

In Kenny Nguyen's TED Talk, The Art of Saying No, the practice of declining certain requests is the key to saying yes to others. If you have set priorities for your students and yourself and expect to get through the school year with your stamina and sanity intact, then you simply can not say 'yes' to every request. 

Whenever you say “yes” to one thing, you are saying “no” to everything else. And, conversely, whenever you say “no” to one thing, you are effectively saying “yes” to your other commitments. In essence, the less commitments that you make allows you to give more of yourself to the commitments that you currently have.

Need some help with setting healthy boundaries and learning how to say “no” more often? Here are some helpful strategies for saying “no” without feeling guilty:

• Be Direct: Whenever saying “no”, it’s important to be clear and concise in your response. Never half-commit or leave any room for misinterpretation.

• Decline with Grace: Always express gratitude for whatever opportunity you are declining and be respectful.

• Don’t Over-Explain: Never feel like you have to give a detailed explanation justifying why you can’t commit to something if you don’t want to.

• Share Alternatives: If possible, you can make suggestions for other solutions or staff members who may be available to help.

Setting Boundaries Will Make You a More Effective Teacher

When you learn to set healthy and effective boundaries, the positives greatly outweigh the negatives. One major benefit is that your students will receive higher quality classroom instruction and lesson planning from a teacher who is able to be energized, present, and focused.

Great teachers, of course, are team players, and saying 'no' sometimes won't change that. Understanding where your limits are and never feeling guilty about putting a cap on how far you're willing to extend yourself is the key to being a healthy and effective educator.

Otherwise, like many teachers often do, you can fall victim to exhaustion and burnout towards the middle/end of the school year, leaving you feeling overwhelmed, unhappy, and ineffective. You just may find out that saying 'no' more often prepares you for the perfect times to say 'yes'!

Do you think it's important for teachers to learn to say 'no' more often? Share your thoughts in the comments section below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

More Education Insights You Will Love:

How to Add Fractions with Different Denominators (Step-by-Step)

How to Add Fractions with Different Denominators

Math Skills: How to Add Fractions with Unlike Denominators in 3 Easy Steps

Ready to learn how to add fractions with unlike denominators?

When it comes to working with fractions and performing operations on them, you will typically first learn how to add fractions. Once you master how to add fractions with the same denominator, it’s time to advance to a more challenging task—learning how to add fractions with different denominators (or unlike denominators).

While adding fractions with a common denominator is relatively simple and straightforward, things get a bit trickier when you are tasked with adding fractions that do not have the same denominator. However, learning how to add fractions with unlike denominators is a skill that every math student can learn by becoming familiar with a few simple steps and by working on a sufficient amount of practice problems (which is exactly what we will be doing in this guide).

This free How to Add Fractions with Different Denominators guide is your step-by-step tutorial to learning how to add fractions with uncommon denominators together. In this guide, we will use an easy 3-step method for adding fractions that you can use to solve any problem where you have to find the sum of two fractions that do not share the same denominator.

You can follow this guide in order (recommended if you are new to adding fractions) or by using the quick-links below to jump to a specific section or example.

• Quick Review: Adding Fractions with the Same Denominator

• How to Add Fractions with Different Denominators: The 3-Step Method

• How to Add Fractions with Different Denominators Example #1

• How to Add Fractions with Different Denominators Example #2

• How to Add Fractions with Different Denominators Example #3

Are you ready to get started?

Before we start working on any practice problems, let’s do a quick review of some key vocabulary as well as how to add fractions with unlike denominators.

Review: Adding Fractions with the Same Denominator

First, let’s make sure that we understand the difference between the numerator and the denominator of a fraction.

Definition: The top number of any fraction is called the numerator. For example, the fraction 5/7 has a numerator of 5.

Definition: The bottom number of any fraction is called the denominator. For example, the fraction 5/7 has a denominator of 7.

In this guide, we will be making reference to numerators and denominators often, so make sure that you understand what these terms mean before moving on.

Figure 01: In any fraction, the numerator is the top number and the denominator is the bottom number.

When it comes to adding fractions, the process is relatively simple when the denominators are the same.

For example, what if you wanted to solve the following problem:

• 1/4 + 2/4 = ?

Notice that the fractions 1/4 and 2/4 have the same denominator (i.e. they both have a denominator of 4).

In cases like this, when both fractions have the same denominator, you can simply add the numerators together and keep the denominator the same as follows:

• 1/4 + 2/4 = (1+2)/4 = 3/4

And, since 3/4 can not be simplified any further, we can say that:

• Final Answer: 1/4 + 2/4 = 3/4

The process for solving this problem is illustrated in Figure 02 below. If you need a more in-depth review of how to add fractions with the same denominator, we recommend checking out our free guide to adding fractions before moving on.

Key Takeaway: When adding fractions with the same denominator, you can simply add the numerators together and keep the same denominator.

Figure 02: When adding fractions with the same denominator, you can simply add the numerators together and keep the same denominator.

Now that we have recapped how to add fractions when the denominators are the same, we are ready to learn to how to add fractions with unlike denominators.

---

How to Add Fractions with Unlike Denominators in 3-Easy Steps

Now, let’s revisit the practice problem from the previous section where we had to add two fractions with the same denominator:

• 1/4 + 2/4 = ?

We already solved this problem and determined that the answer is 3/4. Now, let’s consider another problem where the denominators are different:

• 1/4 + 1/2 = ?

In this new problem, the first fraction has a denominator of 4 and the second fraction has a denominator of 2 (i.e. the fractions have unlike denominators).

However, notice that the second fraction in the first problem, 2/4, and the second fraction in the second problem, 1/2, are equivalent since they both represent one-half.

This means that both problems mean the same thing (i.e. find the sum of one-quarter and one-half) and that they will both have the same answer: 3/4.

With this in mind, let’s learn a 3-step method for adding fractions with unlike denominators and apply it to the problem 1/4 + 1/2 = ? to see if it gives us a result of 3/4.

Figure 03: Two different ways to write the expression “one-quarter plus one-half.” One has like denominators and the other has different denominators.

How to Add Fractions with Unlike Denominators:

• Step One: Get a common denominator by multiplying each fraction by the other’s fraction’s denominator (top and bottom).

• Step Two: Add the numerators together and keep the denominator.

• Step Three: Simplify the result if possible.

That’s all there is to it! Now, let’s go ahead and apply these three steps to 1/4 + 1/2 = ?

Step One: Get a common denominator by multiplying each fraction by the other’s fraction’s denominator (top and bottom).

For this initial step, you have to find a common denominator—a value that the denominators of both fractions divide evenly into.

The easiest way to find a common denominator is to multiply the denominator of the first fraction by the second fraction and then multiply denominator of the second fraction by the first fraction as follows:

• 1/4 + 1/2 = (2x1)/(2x4) + (4x1)/(4x2) = 2/8 + 4/8

Doing this gives us a new equivalent expression that now has a common denominator (both fractions have a denominator of 8). The actions taken to complete this first step are illustrated in Figure 04 below.

Figure 04: How to Add Fractions with Unlike Denominators: You can find a common denominator by multiplying each fraction by the other fraction’s denominator.

Step Two: Add the numerators together and keep the denominator.

Now we have a new expression where both fractions share a common denominator:

• 1/4 + 1/2 → 2/8 + 4/8

Next, we have to add the numerators together and keep the denominator as follows:

• 2/8 + 4/8 = (2+4)/8 = 6/8

Step Three: Simplify the result if possible.

Finally, we are left with the fraction 6/8 and we only have to see if the fraction can be simplified. Since 6 and 8 share a greatest common factor of 2, we can divide both 6 and 8 by 2 to get 3/4 (i.e. the fraction 6/8 simplifies to 3/4) and we can conclude that:

Final Answer: 1/4 + 1/2 = 3/4

Figure 05: How to Add Fractions with Different Denominators in 3 Easy Steps.

This answer should make sense sill we already knew that the end result was going to be 3/4. Now that you are familiar with the 3-step method for adding fractions with different denominators, let’s gain some practice applying them to three different practice problems.

---

How to Add Fractions with Different Denominators Example #1

Example #1: 2/3 + 1/5

For our first example, we have to find the sum of two-thirds (2/3) and one-fifth (1/5). Let’s go ahead and use our 3-step method to add fractions with unlike denominators:

Step One: Get a common denominator by multiplying each fraction by the other’s fraction’s denominator (top and bottom).

Let’s start by finding a common denominator by multiplying the denominator of the first fraction (3) by the second fraction (1/5) and then multiply denominator of the second fraction (5) by the first fraction (2/3) as follows:

• 2/3 + 1/5 = (5x2)/(5x3) + (3x1)/(3x5) = 10/15 + 3/15

Now we have a new expression that is equivalent to the original, except the fractions now have the same denominator:

• 2/3 + 1/5 → 10/15 + 3/15

Step Two: Add the numerators together and keep the denominator.

Next, we can perform 10/15 + 3/15 as follows:

• 10/15 + 3/15 = (10+3)/15 = 13/15

Step Three: Simplify the result if possible.

For the third and final step, we have to see if we can simplify the result (13/15). Since 13 and 15 do not share any common factors other than 1, we can not simplify this fraction any further and we can conclude that:

Final Answer: 2/3 + 1/5 = 13/15

Figure 06 below shows how we determined that 2/3 + 1/5 = 13/15 using our 3-step method.

Figure 06: How to Add Fractions with Unlike Denominators

---

How to Add Fractions with Unlike Denominators Example #2

Example #2: 2/9 + 3/7

We can go ahead and solve this next example by using our 3-step method just like we did for Example #1:

Step One: Get a common denominator by multiplying each fraction by the other’s fraction’s denominator (top and bottom).

We can find a common denominator by multiplying the first fraction (2/9) by 7 and the second fraction (3/7) by 9 as follows:

• 2/9 + 3/7 = (7x2)/(7x9) + (9x3)/(9x7) = 14/63 + 27/63

From here, we have a new equivalent expression to what we started with:

• 2/9 + 3/7 → 14/63 + 27/63

Step Two: Add the numerators together and keep the denominator.

For the second step, we can find the solve of 14/63 + 27/63 as follows:

• 14/63 + 26/63 = (14+27)/63 = 41/63

Step Three: Simplify the result if possible.

Lastly, we have to determine whether or not the result, 41/63, can be simplified. Since 41 and 63 do not share a greatest common factor other than 1, we know that the fraction can not be reduced and:

Final Answer: 2/9 + 3/7 = 41/63

The entire process for solving Example #2 is displayed in Figure 07 below.

Figure 07: Adding Fractions Explained!

By now, you should be starting to feel a little more comfortable with using our 3-step method for solving problems where you have to add fractions with unlike denominators. Let’s gain some more experience by working through one more example.

---

How to Add Fractions with Different Denominators Example #3

Example #3: 3/18 + 2/16

We can solve this final example using our 3-step method as follows:

Step One: Get a common denominator by multiplying each fraction by the other’s fraction’s denominator (top and bottom).

We can find a common denominator by multiplying each fraction by the other fraction’s denominator:

• 3/18 + 2/16 = (16x3)/(16x18) + (18x2)/(18x16) = 48/288 + 36/288

By the end of step one, we are left with a new equivalent expression:

• 3/18 + 2/16 → 48/288 + 36/288

Step Two: Add the numerators together and keep the denominator.

Continue by solving 48/288 + 36/288:

• 48/288 + 36/288 = (48+36)/288 = 84/288

Step Three: Simplify the result if possible.

We’re almost finished. For the third and last step, we have to see if 84/288 can be simplified. Since 84 and 288 share a greatest common factor of 12, we can divide both the numerator, 84, and the denominator, 288, by 12 to get an equivalent reduced fraction, 7/24.

Final Answer: 3/18 + 2/16 = 7/24

Our entire step-by-step approach to solving this last example where we had to add fractions with unlike denominators is shown in Figure 08 below.

Figure 08: How to Add Fractions with Different Denominators

---

Conclusion: How to Add Fractions with Different Denominators in 3 Easy Steps

Understanding how to add fractions is an important math skill that every student must learn. When it comes to adding fractions, there are two common scenarios that you must be familiar with:

• Adding Fractions with Like Denominators

• Adding Fractions with Unlike Denominators

The focus of this guide is on teaching you how to deal with problems related to the second scenario: how to add fractions with unlike denominators.

To solve problems where you have to add fractions with unlike denominators, we learned to use the following 3-step method for how to add fractions with unlike denominators:

• Step One: Get a common denominator by multiplying each fraction by the other’s fraction’s denominator (top and bottom).

• Step Two: Add the numerators together and keep the denominator.

• Step Three: Simplify the result if possible.

By working through three practice problems, we gained experience with adding fractions with unlike denominators to find correct answers that are in simplified form. Since this method can be used to solve any problem where you have to add fractions with unlike denominators, you can use it solve any math problems resembling the ones covered in this guide!

Keep Learning:

How to Subtract Fractions in 3 Easy Steps

---

Are Timed Math Tests Harmful to Students?

Are Timed Math Tests Harmful to Students?

How Timed Math Tests Lead to Math Anxiety and Poor Results

Students with math anxiety are often affected by feelings of tension, apprehension, or fear, which interfere with learning or remembering math facts and skills. (Image: Mashup Math MJ)

Question: Which of these statements best describes an exceptional math student?

• She performs computations faster than her classmates.

• She has memorized lots of facts, formulas, and procedures.

• She scores high grades on exams and works well under pressure.

• She understands number relationships and how to solve complex problems.

If you chose one of the first three statements, then your beliefs about the essence of math understanding may be rooted in misconceptions.

People often allow the prevalence of high-stakes exams to frame mathematics education into a practice in rote memorization and uninspired computations.

As a result, many students lose interest in learning math at a young age.

Large populations of students believing that they can't understand mathematics only breeds more misconceptions, such as the idea that only certain individuals are capable of understanding math.

However, we now know that the idea that only certain people are capable of understanding math is a myth. According to a recent report, The Myth of 'I'm Bad at Math', by The Atlantic, math ability can be improved through effort and learning with a growth mindset.

The truth is that, under the right conditions, anyone can develop math skills.

Are timed math tests harmful to students? (Image: Mashup Math FP)

Where Does the "Math Person" Myth Come From?

A large part of the answer lies in how schools use testing.

The demands of high-stakes exams, which often overpower curriculums, can be felt in math classrooms across the country.

According to a recent report on standardized exams by the Washington Post:

The average student in America’s big-city public schools takes some 112 mandatory standardized tests between pre-kindergarten and the end of 12th grade — an average of about eight a year, the study says. That eats up between 20 and 25 hours every school year.

The frequency of testing is only part of the problem.

Teachers are confined by strict curriculum schedules that force the pace of instruction and assessment.

Under these conditions, teachers are forced to give timed tests that emphasize speed and computation over deep mathematical thinking.

Image: Mashup Math MJ

What are the Consequences of Time Pressure?

Our time-bound approach to testing often leads to math anxiety.

Students with math anxiety are affected by feelings of tension, apprehension, or fear, which interfere with learning or remembering math facts and skills.

And, the problem is only becoming worse.

According to a recent study by the University of Chicago, math anxiety has now been documented in children as young as five, and timed tests are a key cause of this weakening, often lifelong condition.

Timed tests elicit such powerful emotions that students believe that being fast with math facts is the heart of the subject.

The misconception that speed and memorization are the keys to understanding math has resulted in high numbers of students dropping out of math and the depressed numbers of women in STEM-based college majors.

The negative impact of math anxiety is holding back crowds of students in the United States, which continues to be outpaced by other countries.

According to a recent report on global math and science rankings by NPR:

In mathematics, 29 nations and other jurisdictions outperformed the United States by a statistically significant margin, up from 23 three years ago.

Math students in the U.S. can't compete with their global counterparts until they are freed from the debilitating effects of math anxiety.

Image: Mashup Math FP

What Does Math Anxiety Do to the Brain?

Stanford researcher and math education expert, Jo Boaler, has shed much-needed light on the consequences of timed testing in her reports on YouCubed.org, a Stanford-funded organization that focuses on, according to their website, transforming the latest research on math learning into accessible and practical forms.

Boaler points to brain science research suggesting that speed and time pressure blocks working memory, which is where math facts are stored in the brain.

When the working memory is blocked, students become unable to retrieve what they already know.

This inability to recall information under pressure is the hallmark of math anxiety.

According to Boaler's Report on Time Pressure Blocking Working Memory:

Conservative estimates suggest that at least a third of students experience extreme stress related to timed tests, and these are not students from any particular achievement group or economic background. When we put students through this anxiety-provoking experience, they distance themselves from mathematics.

If we continue to assess mathematical understanding using timed tests, then we will continue to turn students away and perpetuate misconceptions.

Image: Mashup Math MJ

How Can We Help Our Students?

The best way to learn math facts is through mathematical activities that focus on understanding number relationships.

This authentic understanding is difficult to achieve in a time-bound environment.

Yet, many people believe that mathematics is only about calculating and recalling math facts -- and that the best mathematical thinkers are those who can calculate the quickest.

In truth, skilled mathematicians are often slow with performing math, because they take the time to think carefully and deeply about mathematics.

If we want our students to become powerful thinkers--ones who can make connections, think logically, and solve complex problems--then systemic changes must be made.

You can take action today by removing or, at the very least, reducing timed tests from your classroom and providing ample opportunities for students to engage in deep mathematical thinking.

You can also keep this conversation going.

In your school. In your classroom. And in your home.

Math education is evolving and the movement towards removing timed testing is building momentum, but it will take a group effort to make real change.

More Math Education Resources You Will Love:

How to Simplify Radicals in 3 Easy Steps

How to Simplify Radicals in 3 Easy Steps

Math Skills: How to Simplify a Radical Using a 3-Step Strategy

Every math student must learn how to work with numbers inside of a radical (√) at some point. While working with perfect squares inside of radicals can be quite easy, the task becomes a bit trickier when non-perfect squares are involved and you have to simplify.

While learning how to simplify a radical of a non-perfect square may seem challenging, it’s actually a relatively easy math skill to learn as long as you have a strong understanding of perfect squares and factoring (we will review both of these topics in this guide).

This free How to Simplify Radicals Step-by-Step Guide will teach you how to simplify a radical when the number inside of it is not a perfect square using a simple 3-step strategy that you can use to solve any problem involving simplifying radicals.

The following topics and examples will be covered in this guide:

• What is the difference between a perfect square and a non-perfect square?

• How to Simplify Radicals: 3-Step Strategy Explained

• How to Simply a Radical Example #1

• How to Simply a Radical Example #2

• How to Simply a Radical Example #3

You can use the quick-links above to jump to any section of this guide. However, we highly recommend that you work through each section in order, including the following review section where we will quickly recap some important features of perfect squares, non-perfect squares, factors, and the properties of radicals. This review will recap some important vocabulary terms and prerequisite math skills that you will need to be successful with this new math skill.

But, before you learn how to add fractions, let’s do a quick review of some key characteristics and vocabulary terms related to fractions before we move onto a few step-by-step examples of how to add fractions.

Let’s get started!

Radicals, Perfect Squares, and Non-Perfect Squares

Before you learn how to simplify a radical, it’s important that you understand what a radical is and what the difference between a perfect square and a non-perfect square is.

Definition: In math, a radical (√) is a symbol that is associated with the operation of finding the square root of a number.

Finding the square root of a number means finding an answer to the question: “What number times itself results in the original number?” For example, the square root of 25 (or √25) is equal to 5 because 5 times 5 equals 25.

Definition: In math, a perfect square is a number that is the square of any integer. This means that a perfect square is a number that can be represented as the product of an integer squared (or an integer multiplied by itself). For example, 36 is a perfect square because 6 times 6 equals 36.

Note that perfect squares are unique and there are not that many of them. In fact, most numbers are considered non-perfect squares because they can not be represented as an integer times itself.

Figure 01: What is a perfect square?

Definition: In math, a non-perfect square is a number that is not the square of any integer. This means that a non-perfect square is a number that can’t be represented as the product of an integer squared (or an integer multiplied by itself). For example, 20 is not a non-perfect square because it is impossible to take an integer and multiply by itself to get a result of 20.

Again, most numbers are non-perfect squares. When we see these types of numbers inside of a radical, they can be simplified or expressed as decimal numbers, but never as an integer.

The chart in Figure 02 below shows all of the perfect squares and non-perfect squares up to 144. Note that the numbers highlighted in orange are all perfect squares. For example:

• 49 is a perfect square (because 7 x 7 = 49)

• 8 is a non-perfect square

Figure 02: Before you learn how to simplify a radical, you must be familiar with the perfect squares.

For quick reference, here is a list of the perfect squares up to 144:

• 1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144

Now that you are familiar with radicals, perfect squares, and non-perfect squares, you are ready to learn how to simplify radicals.

---

How to Simplify Radicals in 3-Easy Steps

Now you are ready to learn how to simplify a radical using our easy 3-step radical.

Let’s start by considering a problem where you are asked to simplify c. You should notice that 16 is a perfect square, so you can easily conclude that √16 = 4 (because 4 x 4 =16).

This kind of problem is pretty easy when the number inside of the radical is a perfect square, but what happens when it is not a perfect square? For example, what if you were dealing with a non-perfect square like 12 inside of the radical? How can you simplify √12? This guide will teach you how to do just that (i.e. how to simplify a radical containing a non-perfect square).

To simplify radicals like √12, we will use the following 3-step strategy:

• Step One: List all of the factors of the number inside of the radical

• Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.

• Step Three: Separate the original radical into two radicals and simplify.

Does this sound confusing? The process gets easier with practice. Let’s go ahead and apply these three steps to √12 as follows:

Step One: List all of the factors of the number inside of the radical

First, we will list all of the factors of 12:

• Factors of 12: 1, 2, 3, 4, 6, 12

Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.

From the list of factors above, we can see that only 4 is a perfect square and it is the only perfect square that is a factor of 12. This is important because we will need to find at least one factor that is a perfect square in order to simplify a radical of a non-perfect square.

• 4 is a factor of 12 and a perfect square

• 4 x 3 = 12

Figure 03: How to split up a radical.

Step Three: Split the original radical into two radicals and simplify.

For the final step, what do we mean by “split up the radical”?

Figure 03 above illustrates the following property of radicals:

• √(ab) = √a x √b (as long as a>0 and b>0)

This means that you can separate a radical into the radicals of two of its factors. In the case of √12, we can rewrite it as follows:

• √12 = √(4 x 3) = √4 x √3

Now, notice that √4 is equal to 2 (because 4 is a perfect square), so we can rewrite the result as follows:

• √12 = = √4 x √3 = 2 x √3

From here, we can not simplify this radical any further, so we can conclude that:

Final Answer: √12 = 2√3

Figure 04 below illustrates how we solved this problem using our 3-step strategy:

Figure 04: How to Simplify Radicals in 3 Easy Steps.

Now that you have learned the 3-step strategy for how to simplify radicals, let’s gain some experience using this strategy to solve a few practice problems.

---

How to Simplify Radicals Example #1

Example #1: Simplify √72

For this first example (and all of the examples in this guide) we will use our 3-step strategy to simplify the given radical as follows:

Step One: List all of the factors of the number inside of the radical

For the first step, let’s list all of the factors of 72:

• Factors of 72: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72

Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.

Next, take a look at the list of factors of 72. Notice that 72 has three facts that are perfect squares other than 1: 4, 9, and 36. In cases like this, you have to choose the largest perfect square, which is 36 for this example.

• 36 is a factor of 72 and 36 is a perfect square

• 36 x 2 = 72

Step Three: Split the original radical into two radicals and simplify.

Finally, we can split the radical into the radicals of two of its factors (namely 36 and 2) as follows:

• √72 = √(36 x 2) = √36 x √2

Since 36 is a perfect square (36 = 6x6), we know that √36=6, so we can say that:

• √72 = = √36 x √2 = 6 x √2

Now we can make the following conclusion:

Final Answer: √72 = 6√2

Figure 05 below illustrates how we simplified √72 for this first example.

Figure 05: How to Simplify Radicals Explained

Let’s continue onto another practice problem!

---

How to Simplify Radicals Example #2

Example #2: Simplify √48

Just as we did in the previous example, we can use our 3-step strategy to simplify √48 as follows:

Step One: List all of the factors of the number inside of the radical

Let’s start by listing all of the factors of 48:

• Factors of 48: 1, 2, 3, 4, 6, 8, 12, 16, 24, 48

Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.

For the next step, notice that 48 has two perfect square factors other than one: 4 and 16. Remember that, if we want to simplify completely, we must choose the largest perfect square factor, which, in this case, is 16.

• 16 is a factor of 48 and 16 is a perfect square

• 16 x 3 = 48

Step Three: Split the original radical into two radicals and simplify.

Lastly, we have to split the radical into the radicals of two of its factors where one of them is a perfect square (in this example, the perfect square factor is 16 and the non-perfect square factor is 3).

• √48 = √(16 x 3) = √16 x √3

Since 16 is a perfect square (16 = 4x4), we can rewrite √16 as 4:

• √48 = √16 x √3 = 4 x √3

And now we have our final answer:

Final Answer: √48 = 4√3

Figure 06 below shows how we used our 3-step strategy to simplify the radical √48.

Figure 06: How to Simply a Radical: √48 = 4√3

Are you feeling better about using our 3-step strategy to simplify radicals? Let’s move on and work through one more example.

---

How to Simplify Radicals Example #3

Example #3: Simplify √320

For this final example, we have to simplify a radical with a triple-digit non-perfect square inside of it: √320. We can do just that by using our 3-step strategy:

Step One: List all of the factors of the number inside of the radical

Start by listing all of the factors of the number inside of the radical, which, in this case, is 320:

• Factors of 48: 1, 2, 4, 5, 8, 10, 16, 20, 32, 40, 64, 80, 160, 320

Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.

Next, identify all of the perfect square factors of 320 other than 1. In this case, we have 4, 16, and 64. The largest of these three perfect square factors is 64.

• 64 is a factor of 320 and 64 is a perfect square

• 64 x 5 = 320

Step Three: Split the original radical into two radicals and simplify.

For the very last step, we must split the radical √320 into the radicals of two of its factors. For this third example, the perfect square factor is 64 and the non-perfect square factor is 5.

• √320 = √(64 x 5) = √64 x √5

Since 64 is a perfect square (64 = 8x9), we can rewrite √64 as 8:

• √320 = √64 x √5 = 8 x √5

All that we left to do is conclude that:

Final Answer: √320 = 8√5

The graphic in Figure 07 below illustrates how we solved this problem using our 3-step strategy.

Figure 07: How to Simply Radicals: √320 = 8√5

---

Conclusion: How to Simplify Radicals in 3 Easy Steps

Whenever you have to simplify a radical with a non-perfect square inside, you have to find two factors of that number, one of which must be a perfect square. Once you find two factors that meet these conditions, you can simplify the perfect square and rewrite the radical in simplified form.

The process of simplifying radicals of non-perfect squares can done by following these three steps:

• Step One: List all of the factors of the number inside of the radical

• Step Two: Determine if any of the factors are perfect squares (not including 1). If there are multiple perfect squares, choose the largest one.

• Step Three: Separate the original radical into two radicals and simplify.

As long as you can follow these steps, you can learn how to simplify radicals and you can solve a variety of problems.

Keep Learning:

How to Subtract Fractions in 3 Easy Steps

---