The Vertical Line Test Explained in 3 Easy Steps

What is the Vertical Line Test in Math?

Key Question: What is the vertical line test function and how can it be used?

Learning how to identify whether or not a given relation is a function is an extremely important math skill (most notably in pre-algebra and algebra). Luckily, there is a simple and handy tool called the vertical line test that you can always use to see if a relation can be considered a function or not just by looking at its graph.

This free Vertical Line Test step-by-step guide will teach you how to figure out whether or not a relation is or is not a function by looking at its graph and using a simple mathematical tool called the vertical line test.

Before you learn how to use the vertical line test, we will do a quick review of some very important vocabulary and definitions related to functions and relations.

Are you ready to get started?

Vertical Line Test Definitions and Vocabulary

What is a relation?

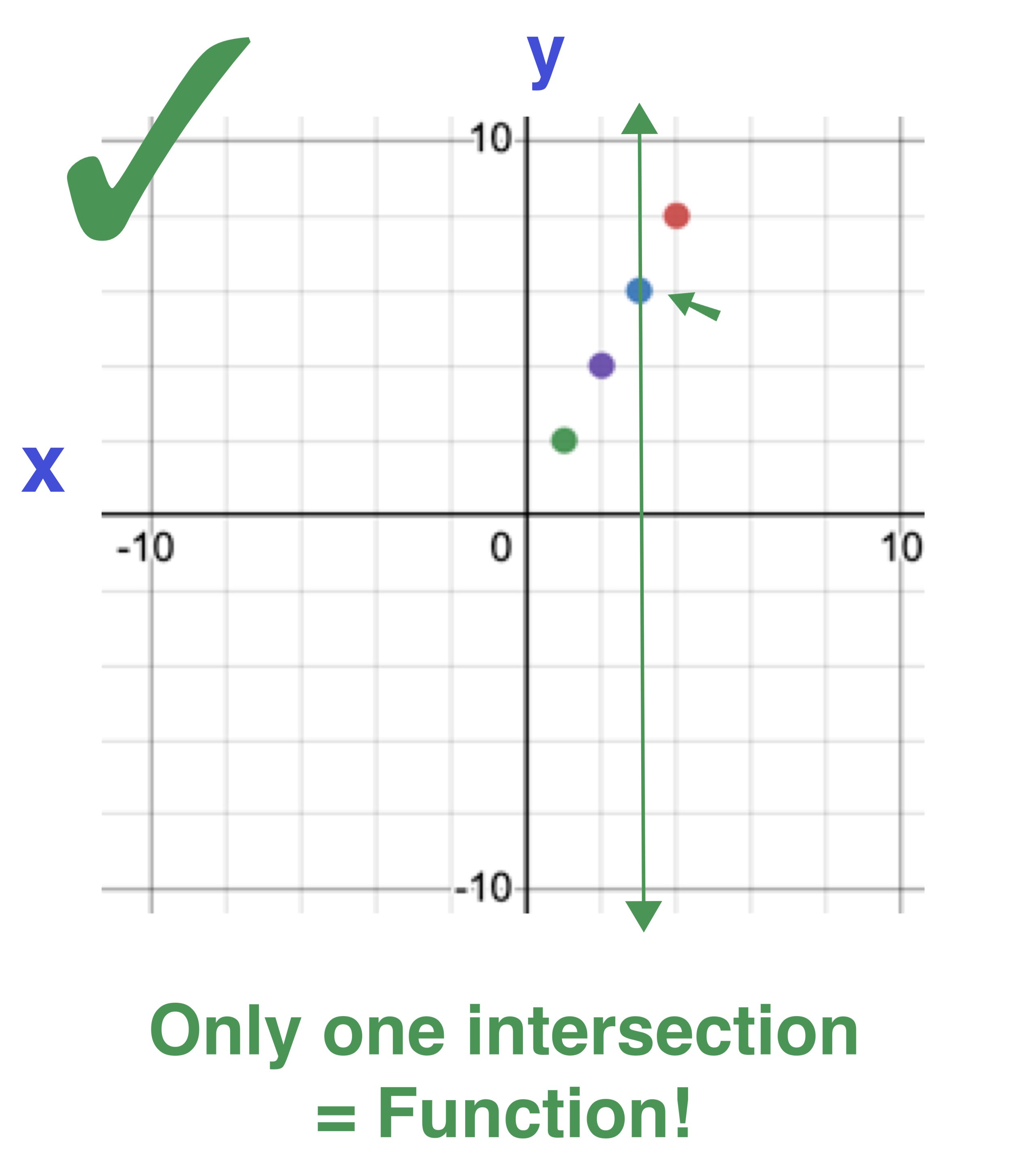
Definition: A relation is how a set of inputs and outputs of a system are related to each other. Mappings and graphs are the most common ways of representing relations. The figures below show a graphic and map representation of the relation between a set of numbers and the square of those numbers.

Figure 01: Relation Mapping

Figure 02: The graph representing the relation in Figure 01.

What is a function?

Definition: A function is a relation between a set of inputs and outputs where, for every input, there is one (and only one) output. In other words, a function is a relation where every input has only one output.

If this is confusing, observe the mappings in Figure 03 and Figure 04 below for more insight.

Figure 03: The relation is a function.

Figure 04: The relation is NOT a function.

The relation mapping in Figure 03 represents a function because every input (or x-value) has only one unique output (or y-value).

The relation mapping in Figure 04 does not represent function because at least one of the inputs (in this case 6) has more than one output. If you look at the mapping, you will notice that 6 has two outputs: 1 and 3. Thus, the relation in Figure 04, by definition, is not function.

Keep this information in mind as we move on and extend your understanding of relations and functions to looking at graphs and learning how to apply the vertical line test.

What is the difference between a relation and a function?

Functions are a subset of relations. Simply put, all functions are relations, but not all relations are functions.

In other words, a relation represents the relationship between an input and an output, while a function—more specifically—is a type of relation where there is only one output for each input.

What is the Vertical Line Test Function?

Now that you understand how to determine whether or not a relation is a function by looking at a mapping, you are ready to learn to make this same determination just by looking at a relation’s graph and using a tool called the vertical line test.

The Vertical Line Test helps you to determine if a given relation is a function. Now, let's find out how the vertical line test works with realistic examples.

Again, for a relation to be a function, the relation must have exactly one output for a given input. Let's revisit the relations shown in Figure 03 and Figure 04, but this time we will represent them graphically:

Figure 05: The graph of the relation in Figure 03, which was, by definition, a function.

Figure 06: The graph of the relation in Figure 04, which was, by definition, not a function.

We already know that the graph in Figure 05 represents a function and that the graph in Figure 06 does not represent a function because we already applied the definition of a function to the corresponding mappings.

But what if we had to make this determination using only the graph without the mappings? This is where the vertical line test comes into play.

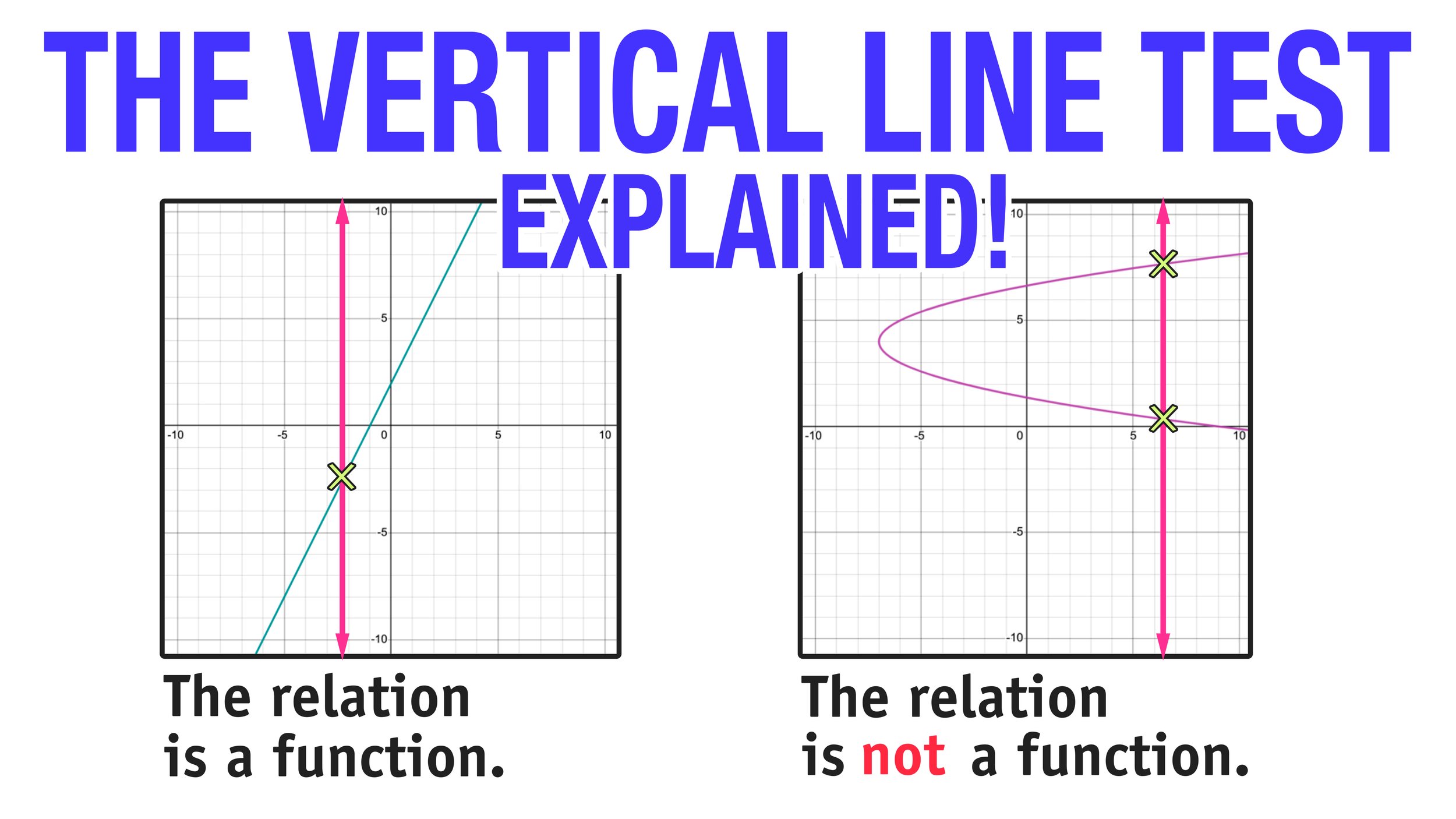
The Vertical Line Test Explained in 3 Easy Steps

The vertical line test is a simple and straightforward tool for determining whether not the graph of a relation represents a function or not.

Applying the vertical line test is as easy as following these 3 easy steps:

Step One: Observe the given graph and draw one or multiple vertical lines through the graph.

Step Two: Note how many times the vertical line(s) that you drew intersected the graph (either coordinate points or a line/curve).

Step Three: Based on the number of intersections, determine whether or not the relation is a function.

• If your vertical line intersects the graph only once, then the relation is a function.

• If your vertical line intersects the graph more than once at any point, the relation is not a function.

We can apply the vertical line test to the graphs from Figure 05 and Figure 06 below to confirm what we already knew (that one graph represents a function and the other does not).

Figure 07: The vertical line test confirms that the relation is a function because there is only one intersection point.

Figure 08: The vertical line test confirms that the relation is a not function because there are multiple intersection points.

Notice how the vertical line drawn on the graph in Figure 07 only has one intersection point. Furthermore, there are no other areas on the graph where you could draw a vertical line where there would be more than one intersection point. Therefore, the graph represents a function.

Notice how the vertical line drawn on the graph in Figure 08 has multiple intersection points (namely through (6,1) and (6,3)), therefore this graph does not represent a function.

Now that you understand how the vertical line test works, let’s apply it to three more examples…

Vertical Line Test Examples

Directions: Use the vertical line test to determine whether or not the graph of each relation is a function.

Example A: Is the relation a function?

We can determine whether or not this graph represents a function by drawing one (or multiple vertical lines) through the graph to see if there is any point where the line intersects the graph more than once (if it does, then the relation is not a function).

The vertical line test shows…

Here we can clearly see that the relation fails the vertical line test and that the graph does not represent a function because there are multiple intersection points.

Final Answer: The relation is not a function because it fails the vertical line test.

Example B: Is the relation a function?

Again, you can use the vertical line test to determine whether or not the graph above represents a function.

Here we can clearly see that the relation passes the vertical line test and that the graph represents a function because there is only one intersection point.

Final Answer: The relation is a function because it passes the vertical line test.

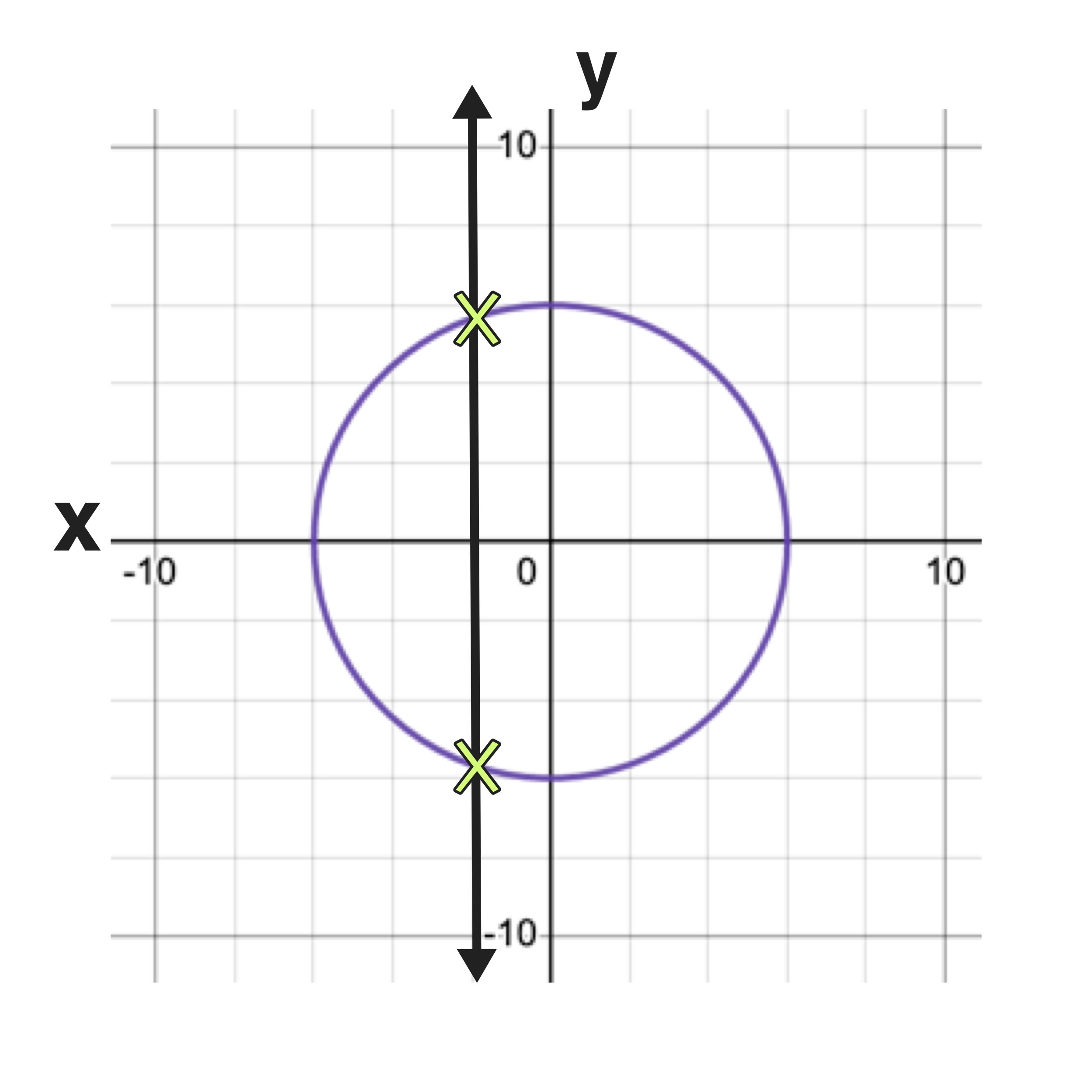
Example C: Is the relation a function?

Are you starting to get the hang of it? Go ahead and apply the vertical line test to see if the graph of the circle represents a function.

Here we can clearly see that the relation fails the vertical line test and that the graph does not represent a function.

Final Answer: The relation is not a function because it fails the vertical line test.

(This will always be the case with circles)

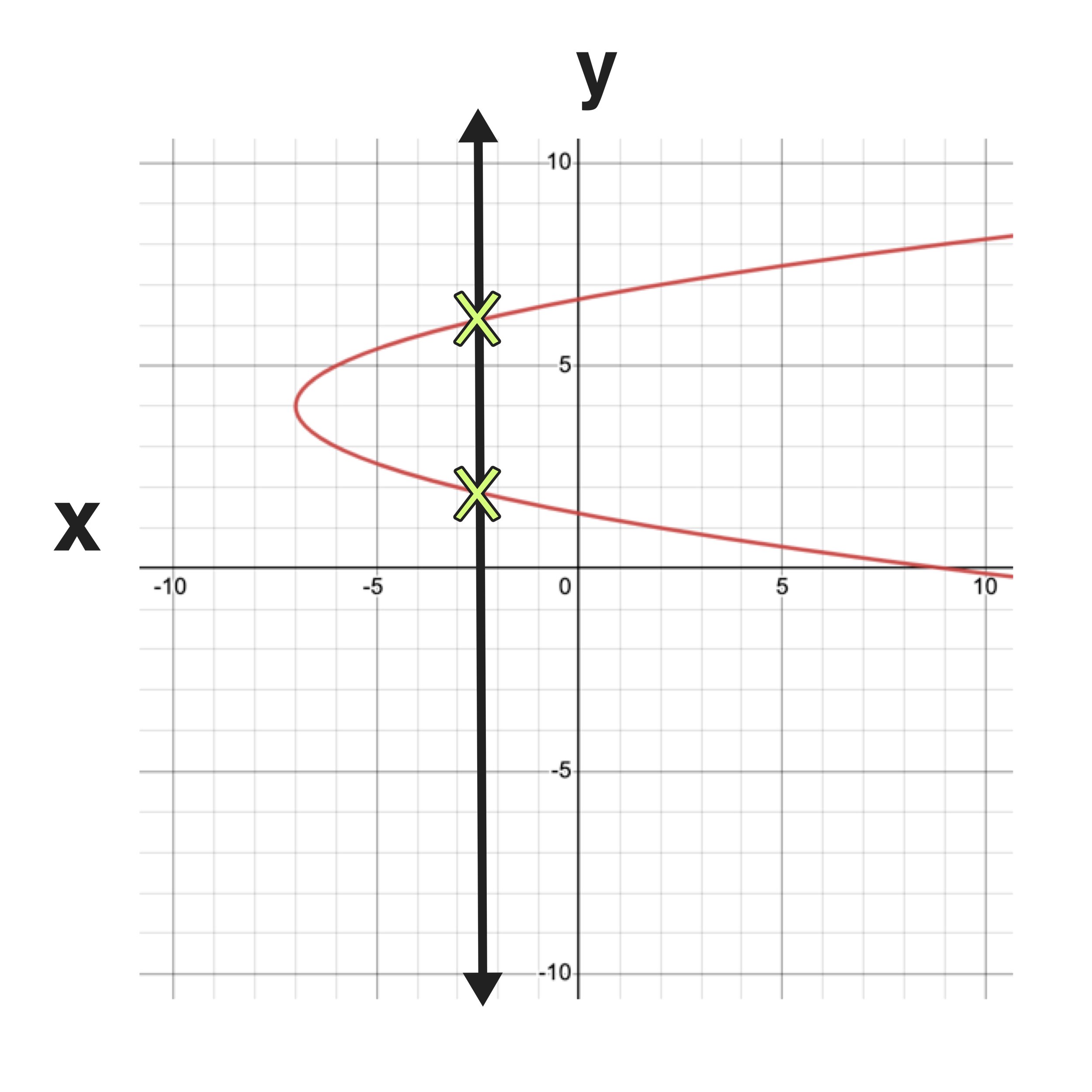
Example D: Is the relation a function?

Remember that the graph of a relation has to pass the vertical line test everywhere in order to confirm that the relation is a function.

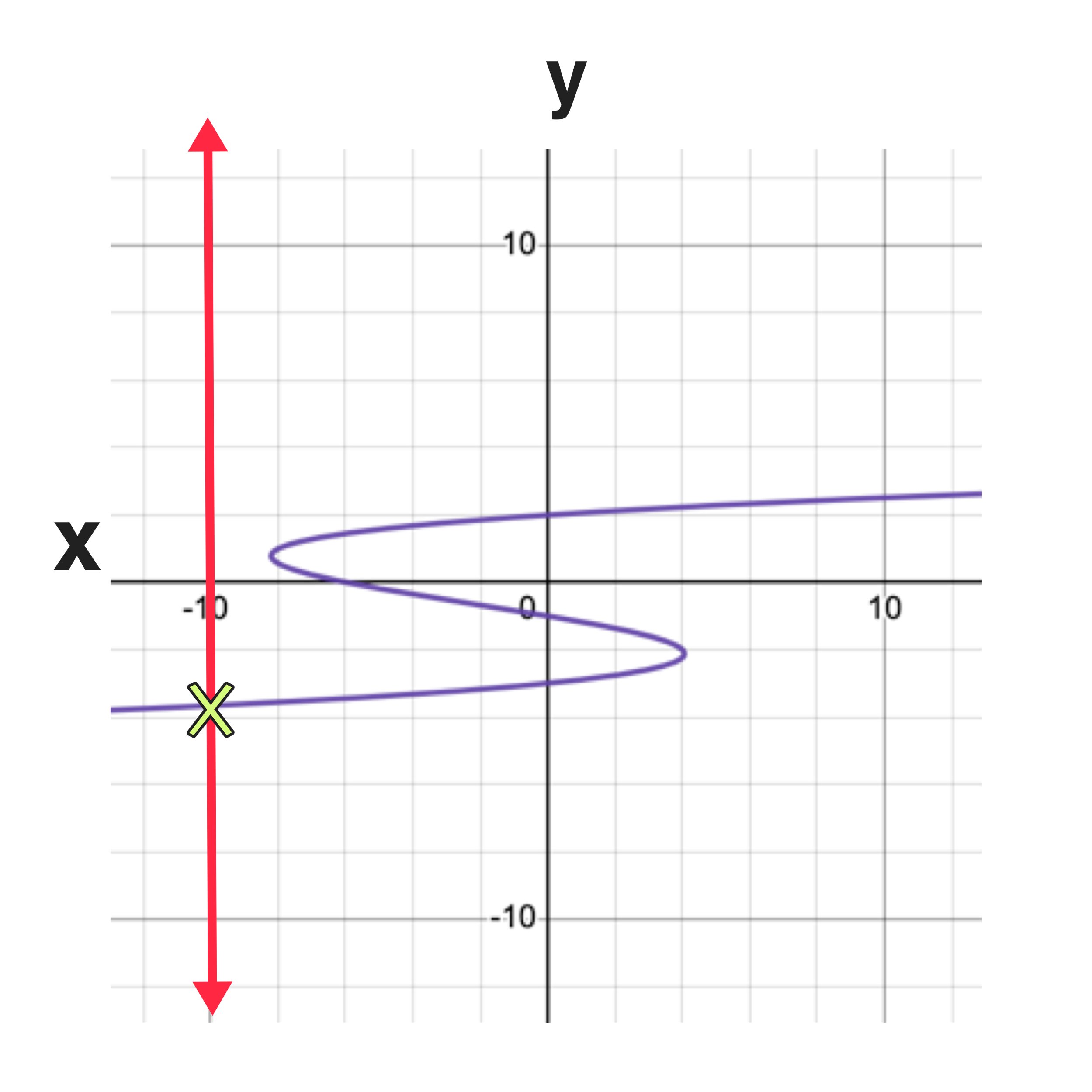
For example, if you drew your vertical line at x=-10 as in Figure 09 below, you might incorrectly conclude that the graph passes the vertical line test (since there is only one intersection point).

But, if you draw multiple vertical lines, such as the one through x=2 in Figure 10 below, you will see that this graph actually fails the vertical line test.

Figure 09: Does the vertical line test pass everywhere?

Figure 10: If the vertical line test fails anywhere on the graph, then the relation is not a function.

Final Answer: The relation is not a function because it fails the vertical line test.

Conclusion/Recap:

The vertical line test is a simple, yet effective tool that can be used to determine whether or not the graph of any given relation represents a function (a specific type of relation where for every input there is one and only one output). To make this determination, simply draw a vertical line through the graph and see how many times the vertical line intersects with the graph of the function. If the vertical line only intersects with the graph one time at any given point, then the graph represents a function. However, if the vertical line intersects with the graph at any point more than once, then the graph does not represent a function.

Recap:

Search Tags: vertical line test, vertical line test function, vertical line test examples, the vertical line test, vertical line test definition math, vertical line test to determine function, vertical line test practice

Keep Learning:

Associative Property of Multiplication Explained in 3 Easy Steps

Associative Property of Multiplication Definition

In math, the associative property of multiplication is a rule which states that the groupings of values or variables being multiplied together does not affect the product or outcome.

The associative property of multiplication can help you to model and solve simple and complex multiplication problems. This rule is a fundamental law in mathematics and applies to any multiplication problem.

The following guide to understanding and applying the associative property of multiplication will share a step-by-step tutorial as well as a free associative property of multiplication worksheet.

Let’s start off by looking at a few examples…

Associative Property of Multiplication Example

Take a look at the equation above:

(a x b) x c = a (b x c)

Notice that the terms (a, b, and c) are in the same order, but grouped differently. On the left side of the equals sign, a and b are in parenthesis. On the right side of the equal side, b and c are in parenthesis. And, according to the order of operations, you must perform operations inside of parenthesis first.

So, according to the associative property of multiplication, the left side of the equal sign and the right side of the equal sign will always equal the same value, no matter what values a, b, and c represent.

Now, let’s go ahead and explore why the associative property proves this equation to be true by substituting numerical values in for a, b, and c:

a=8, b=4, c=2

Step One: Follow the order of operations by performing multiplication inside of the parenthesis first.

In this example, perform (8 x 4) on the left side of the equation and (4 x 2) on the right side of the equation as follows:

(8 x 4 ) x 2 = 8 x (4 x 2)

32 x 2 = 8 x 8

Step Two: After working out the products inside of the parenthesis, the next step is to multiply the next line of the equation. In this case, 32 x 2 on the left side, and 8 x 8 on the right side.

32 x 2 = 8 x 8

64 = 64

Step Three: Verify your answer.

Remember that, according to the associative property of multiplication definition, the groupings of values or variables being multiplied together does not affect the product or outcome. Therefore, the left side of the equation and the right side of the equation should equal the same value.

In this example, the associative property holds true since both sides of the equation are equal to 64.

See Also: Check Out This Awesome Mean, Median, and Mode Activity

Associative Property of Multiplication Example Recap:

(8 x 4 ) x 2 = 8 x (4 x 2)

32 x 2 = 8 x 8

64 = 64 ✓

What About Division?

Now that you understand the associative property of multiplication, let’s see if the inverse of multiplication—division—is also associative.

What if we reused the associative property of multiplication example from before, but changed the multiplication signs to division signs?

Just like the last example, a=8, b=4, and c=2, the order of the terms is the same on both sides of the equal sign, but the terms are grouped differently. And also notice that the operation is no longer multiplication, but division.

Step One: Follow the order of operations by performing division inside of the parenthesis first.

In this example, perform (8 ÷ 4) on the left side of the equation and (4 ÷ 2) on the right side of the equation as follows:

(8 ÷ 4 ) ÷ 2 = 8 ÷ (4 ÷ 2)

2 ÷ 2 = 8 ÷ 2

Step Two: After working out the quotients inside of the parenthesis, the next step is to divide the next line of the equation. In this case, 2 ÷ 2 on the left side, and 8 ÷ 8 on the right side.

2 ÷ 2 = 8 ÷ 2

1 ≠ 4

Step Three: Verify your answer.

Remember that, the associative property states that different groupings of the terms in an equation should not change the result. In the first example, we proved that the associative property works for multiplication since both sides of the equation equaled the same number (64=64).

However, in the division example, we see that the left side of the equation and the right side of the equation result in different values. In this case, 1 and 4, which are obviously nit equal.

Therefore, in this example, the associative property does not hold true for division since both sides of the equation are not equal.

Associative Property of Division Example Recap:

(8 ÷ 4 ) ÷ 2 = 8 ÷ (4 ÷ 2)

2 ÷ 2 = 8 ÷ 2

1 ≠ 4 ✕

Conclusion: Associative Property Math Facts

The previous example has shown us that the associative property works for multiplication, but it does not work for division.

Therefore, as long as all of the terms are being multiplied (not divided), the groupings of values or variables being multiplied together does not affect the product or outcome.

So, (a x b) x c = a x (b x c) for any real number values represented by a, b, and c.

Key idea: The associative property of multiplication can be applied when the terms are in the SAME ORDER, but GROUPED DIFFERENTLY.

The Associative Property of Multiplication Video

Are you looking for a more in-depth and visual explanation of the associative property of multiplication? Check out our free YouTube video using the link below:

Tags: associative property of multiplication, associative property example, associative property of multiplication example, associative property of multiplication definition, associative property in math, associative law of multiplication, the associative property of multiplication

---

Have thoughts? Share your thoughts in the comments section below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

By Anthony Persico

Anthony is the content crafter and head educator for YouTube's MashUp Math. You can often find me happily developing animated math lessons to share on my YouTube channel . Or spending way too much time at the gym or playing on my phone.

More Free Math Resources You Will Love:

Number Bonds Explained: Free Worksheets Included

What is a Number Bond?

Number Bond Definition

What is a number bond? A number bond is a simple visual math aid that is used to show a given number can be represented as the sum of two numbers.

Do you want free K-8 math resources in your inbox every week? Click here to join our mailing list

Number Bond Example: 5

Let’s take a closer look at the number 5. You know that the number 5 can be thought of as the sum of 4 and 1, the sum of 3 and 2, or the sum of 5 and 0. In terms of number bonds, these different ways of expressing the number 5 as the composite sum of two numbers can be visually represented using number bonds as follows:

And, since addition is commutative, meaning that the order of the terms does not matter (e.g. 2+3=5 and 3+2=5), you can also express the above number bonds in reverse order as follows:

Looking to learn more about the commutative math property? Click here to access our free step-by-step guide

Now that you understand what number bonds are and what they represent, it’s time to explore why they are such a useful tool for helping your students to develop strong number sense and fluency with performing operations on numbers.

For starters, the visual nature of number bonds is an excellent way to help students to conceptualize numbers and how they can be expressed as a composite sum of two numbers. By understanding this composite nature of numbers, students are better equipped to perform mental math quickly and accurately and perform advanced operations.

 For example, imagine a student becoming familiar with the following number bonds for 10:

With this understanding, a student could easily and accurately solve an expanded addition problem by combining terms that equal 10 as follows:

Number Bond Example: Number Bonds to 10

Now that you understand the value of having your students practice and understand number bonds, you can start by focusing on number bonds to 10. The diagram below shows the number bonds to 10.

Free Number Bonds Worksheet

 Are you looking for free printable number bonds worksheets (with answer keys included) that will give your students plenty of practice with completing number bonds to 10? If so, use the links below to download your free pdf number bonds worksheet.

➔ Click here to download your free Number Bonds worksheet

Subtraction Number Bonds

Everything related to number bonds that we have covered so far have related to the fact that number bonds represent how a number can be expressed as the sum of two other numbers. But what about subtraction? We know that addition and subtraction share an inverse relationship. This inverse relationship can be explored via number bonds (note that this is an advanced step that should not be explored until your students have completely mastered the number bonds to 10 and possibly beyond.

Number Bond Example 03: Subtraction

For example, how could a student use her understanding of number bonds to solve the problem 10 - ___ = 7?

In this example, the student knows that one of the number bonds for 10 includes the number 7 and that the other number is 3 (because 7+3=10).

With this understanding in mind, it is a logical conclusion that 10 – 3 = 7 meaning that the missing number is 3.

This answer may seem extremely simple to find and you may even think that the use of a number bond is not even necessary. However, if we rewrite this problem as:

10 – X = 7 where x=3

We can see how understanding number bonds can apply to more advanced problems including high school level algebra.

Observe the diagram below that represents this application of subtraction number bonds:

Conclusion

Number bonds are simple visual tools that are used to show how a number can be represented as a composite sum of two other numbers. A number bond includes a whole number with two branches stemming from the number and leading to the two other numbers whose sum is equal to the original whole number. Number bonds are a valuable visual tool for helping students to practice and develop number sense, which is a critical foundational math skill that students will need to be successful at higher level of mathematics.

Do you want free K-8 math resources in your inbox every week? Click here to join our mailing list

Tags:  number bond, number bonds to 10, number bond example, number bonds worksheet, number bonds to 10 worksheet, number bonds to 5, number bonds kindergarten, subtraction number bonds, complete the number bond

---

Share your thoughts in the comments section below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

By Anthony Persico

Anthony is the content crafter and head educator for YouTube's MashUp Math. You can often find me happily developing animated math lessons to share on my YouTube channel . Or spending way too much time at the gym or playing on my phone.

More Free Math Resources You Will Love:

What is the Cube Root of...

What is the Cube Root of…

The following reference posts shares the cube roots of the most often searched values.

What is a cube root?

The cube root of a number is the value that produces that number when cubed.

For example, the cube root of 64 is 4 because 4^3 (4x4x4) equals 64.

What is the Cube Root of 1?

The cube root of 1 is 1 because 1x1x1=1.

What is the Cube Root of 8?

The cube root of 8 is 2 because 2x2x2=8.

What is the Cube Root of 27?

The cube root of 27 is 3 because 3x3x3=9.

What is the Cube Root of 64?

The cube root of 64 is 4 because 4x4x4=64.

What is the Cube Root of 125?

The cube root of 125 is 5 because 5x5x5=125.

Download your free Perfect Cubes and Cube Roots reference sheet.

What is the Cube Root of 216?

The cube root of 216 is 6 because 6x6x6=216

What is the Cube Root of 343?

The cube root of 343 is 7 because 7x7x7=343

What is the Cube Root of 512?

The cube root of 512 is 8 because 8x8x8=512

What is the Cube Root of 729?

The cube root of 729 is 9 because 9x9x9=729

What is the Cube Root of 1000?

The cube root of 1000 is 10 because 10x10x10=1000

What is the Cube Root of 1331?

The cube root of 1331 is 11 because 11x11x11=1331

What is the Cube Root of 1728?

The cube root of 1728 is 12 because 12x12x12=1728

What is the Cube Root of 2196?

The cube root of 2196 is 13 because 13x13x13=2196

What is the Cube Root of 2744?

The cube root of 2744 is 14 because 14x14x14=2744

What is the Cube Root of 3375?

The cube root of 3375 is 15 because 15x15x15=3375

What is the Cube Root of 4096

The cube root of 4096 is 16 because 16x16x16=4096

What is the Cube Root of… (Special Cases)

What if a cube root is not a whole number?

If a number is not a perfect cube, it’s cube root will be a decimal.

What is the Cube Root of 2?

The cube root of 2 is approximately 1.26 because 1.26x1.26x1.26 ≈ 2

What is the Cube Root of 3?

The cube root of 3 is approximately 1.44 because 1.44x1.44x1.44 ≈ 3

What is the Cube Root of 4?

The cube root of 4 is approximately 1.59 because 1.59x1.59x1.59 ≈ 4

What is the Cube Root of 9?

The cube root of 9 is approximately 2.08 because 2.08x2.08x2.08 ≈ 9

What is the Cube Root of 16?

The cube root of 16 is approximately 2.52 because 2.52x2.52x2.52 ≈ 16

What is the Cube Root of 81?

The cube root of 81 is approximately 4.33 because 4.33x4.33x4.33 ≈ 81

Learn How to Graph Cube Roots and Cubic Functions:

Share your ideas, questions, and comments below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

Need Practice Finding Cube Roots?

Click the link below to download your free perfect cubes and cube roots practice worksheet:

PDF: Perfect Cubes and Cube Roots Practice Worksheet

Keep Learning:

Multiplying Square Roots and Multiplying Radicals Explained

Learn How to Multiply Radicals and How to Multiply Square Roots in 3 Easy Steps (Free Worksheet Included)

Learn How to Multiply Radicals (and How to Multiply Square Roots) in 3 Easy Steps

Before you learn how to multiply radicals and how to multiply square roots, you need to make sure that you are familiar with the following vocabulary terms:

Radical vs. Radicand

The radical is the square root symbol and the radicand is the value inside of the radical symbol. The radicand can include numbers, variables, or both.

The Multiplication Property of Square Roots

The key to learning how to multiply radicals is understanding the multiplication property of square roots.

The property states that whenever you are multiplying radicals together, you take the product of the radicands and place them under one single radical.

For example, radical 5 times radical 3 is equal to radical 15 (because 5 times 3 equals 15).

How to Multiply Radicals and How to Multiply Square Roots Example

Now let’s take a look at an example of how to multiply radicals and how to multiply square roots in 3 easy steps.

Problem:

Step One: Simplify the Square Roots (if possible)

In this example, radical 3 and radical 15 can not be simplified, so we can leave them as they are for now.

Step Two: Multiply the Radicands Together

Now you can apply the multiplication property of square roots and multiply the radicands together. In this case, radical 3 times radical 15 is equal to radical 45 (because 3 times 15 equals 45).

Step Three: Simplify the Result (if possible)

The third and final step is to simplify the result if possible.

Can radical 45 be simplified?

The answer is yes.

Since radical 45 is equal to radical 9 times radical 5, and because radical 9 is equal to 3 (since 9 is a perfect square), we can simplify radical 45 to 3 times radical 5 (see the diagram below for a more detailed look on how to simplify square roots).

Finally, we can conclude that the final answer is:

Looking for more sample problems? Check out the free video lesson below to learn more about how to multiply radicals and how to multiply square roots :

---

How to Multiply Radicals and How to Multiply Square Roots Worksheet (with Answer Key)

Are you looking to get some more practice with multiplying radicals, multiplying square roots, simplifying radicals, and simplifying square roots?

Click the link below to access your free practice worksheet from Kuta Software:

Free Multiplying Radicals Worksheet

Share your ideas, questions, and comments below!

(Never miss a Mashup Math blog--click here to get our weekly newsletter!)

Keep Learning:

By Anthony Persico

Anthony is the content crafter and head educator for YouTube's MashUp Math . You can often find me happily developing animated math lessons to share on my YouTube channel . Or spending way too much time at the gym or playing on my phone.